Banking of Road

Science > Physics > Circular Motion > Banking of Road

In this article, we shall study the necessity of the banking of road and the factors affecting it.

Safe Velocity of a Vehicle on an Unbanked Road:

The necessary centripetal force required to negotiate a turn by a vehicle moving along a horizontal unbanked curved road is provided by the force of friction between the wheels (tyres) and the surface of the road.

Let us consider a vehicle of a mass ‘m’ is moving along a horizontal curved road of radius ‘r’ with speed ‘v’.

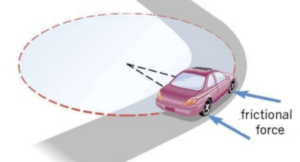
Let μ be the coefficient of friction between the road surface and the wheels then

This expression gives the maximum speed with which a vehicle can be moved safely along a horizontal curved road. If speed is more than this velocity, then there is a danger that the vehicle will get thrown (skid) off the road.

Thus the safe velocity on the unbanked road depends on the coefficient of friction between the road and tyres, the radius of the circular path, and acceleration due to gravity at that place.

Banking of Road:

To make the turning of a vehicle on a curved road safer, the outer edge of the road is raised above the inner edge making some inclination with the horizontal. This is known as banking of road.

When the road is banked then, the inclination of the surface of the road with the horizontal is known as the angle of banking.

Necessity of Banking of Road:

• As the speed of vehicle increases, the centripetal force needed for the circular motion of vehicle also increases. In the case of unbanked road necessary centripetal force is provided by the friction between the tyres and the surface of the road. But there is a maximum limit for frictional force, which depends on the coefficient of friction between the wheels and road.
• When the centripetal force needed exceeds the maximum limit of frictional force, the vehicle skids and tries to go off the curved path resulting in an accident.
• Without proper friction, a vehicle will not be able to move on the curved road with a large speed. To avoid this we may increase the force of friction making the road rough. However, this results in the wear and tear of the tyres of the vehicle. Also, the force of friction is not always reliable because it changes when roads are oily or wet due to rains etc. To eliminate this difficulty, the curved roads are generally banked.
• Due to banking of the road, the necessary centripetal force is provided by the component of the normal reaction.

The Expression for the Angle of Banking of Road:

Consider a vehicle of mass ‘m’ is moving with speed ‘v’ on a banked road of radius ‘r’ as shown in the figure. Let ‘θ’ be the angle of banking. The weight “mg” of the vehicle acts vertically downwards through its centre of gravity G, and N is the normal reaction exerted on the vehicle by banked road AC. N is perpendicular to road AC. Let ‘f’ be the frictional force between the road and the tyres of the vehicle.

Now, the normal reaction is resolved into two components (N cosθ) along the vertical (acting vertically upward) and (N sinθ) along the horizontal (towards left) as shown in the figure. Similarly, the frictional force ‘f’ can be resolved into two components (f sinθ) along the vertical (acting vertically downward) and( f cosθ) along the horizontal (towards left) as shown in the figure.

The free-body diagram of a car is as follows

Considering equilibrium

Total upward force = Total downward force

∴   N cosθ  = mg + f sinθ

∴ mg = N cosθ – f sinθ ….. (1)

The horizontal components N sinθ  and f cosθ  provides the necessary centripetal force

mv²/r = N sinθ + f cosθ …… (2)

Dividing equation (2) by (1)

Now, frictional force f  = μ_sN
Where μ_s = coefficient of friction between road and tyres

This is an expression for the velocity of a vehicle on a curved banked road.

Note:

For the given pair of road and tyre μ_s = tan λ, where λ = angle of friction

Case – I: When the road is horizontal θ = 0°

This is an expression for safe velocity on the unbanked road

Case – II:

When the frictional force between the road and tyres of the vehicle is negligible μ_s = 0.

This is an expression for the angle of banking of a road.

When friction is not mentioned in the problem, use this expression to solve the problems.

Safe Velocity on Banked Road:

The expression for safe velocity on the banked road is

The speed will be maximum when tan θ = 1 i.e. θ = 45°. It means the vehicle can be driven with maximum safe speed only when the angle of banking = 45°.

Factors affecting the angle of banking:

• The angle of banking depends on the speed of the vehicle, the radius of the curved road and the acceleration due to gravity g at that place.
• The expression does not contain the term m representing mass, thus the angle of banking is independent of mass ‘m’ of the vehicle. Thus the angle of banking is the same for heavy and light vehicles.
• the angle of banking depends on the radius (r) of the curved road. The angle of banking is inversely proportional to the radius of curvature.
• For the given radius of the curve, the angle of banking increases with the speed.
• For the given radius of the curve and speed of the vehicle angle of banking is inversely proportional to the acceleration due to gravity at that place. The acceleration due to gravity is more on the pole than that at the equator. Thus for the given radius of the curve and speed of the vehicle angle of banking is less at the pole than that at the equator.

If l is the width of the banked road, then the elevation of an outer edge of the road over the inner edge (provided angle of banking is small) is given by h = l sinθ

In actual practice, some frictional forces are always present even on the banked road. So that the actual safe velocity is always greater than the calculated safe velocity on the banked road.

Characteristics of Angle of Banking:

• The angle of banking is given by

• The angle of banking depends on the speed of the vehicle, the radius of the curved road and the acceleration due to gravity g at that place.
• The angle of banking is independent of mass ‘m’ of the vehicle. Thus the angle of banking is the same for heavy and light vehicles.
• the angle of banking is directly proportional to the square of the velocity (v) of the vehicle, inversely proportional to the radius (r) of curvature of the path and inversely proportional to the acceleration due to gravity (g) at that place.
• The acceleration due to gravity is more on the pole than that at the equator. Thus for the given radius of the curve and speed of the vehicle angle of banking is less at the pole than that at the equator.
• The elevation outer edge of a banked road above the inner edge is given by

h = l sinθ

Characteristics of Safe Velocity on Banked Road:

• The safe velocity on the banked road is given by

• The safe velocity of a vehicle on the banked road depends on the radius of the curved road, the angle of banking, and the acceleration due to gravity g at that place.
• Safe velocity is independent of mass ‘m’ of the vehicle. Thus it is the same for heavy and light vehicles.
• For the given radius of the curve and angle of banking, the safe velocity is more at the pole than that at the equator.

High Speed on Unbanked Road:

• During motorcycle race, the riders negotiate the curve on a flat road in that case they have to tilt their motorcycle and themselves to compensate the angle of banking at that place.

• A cycling race track is called velodrome which has a saucer-shaped track. The rider has to take a position on a track as per his/her speed.

Overturning of Vehicle:

Unbanked Road:

When a vehicle moves along a curved path with very high speed, then there is a chance of overturning of the vehicle. Inner wheel leaves ground first. Let a vehicle of a  mass ‘m’ is negotiating a turn along a curved path of radius ‘r’ with speed ‘v’. Let ‘2a’ be the length of the axle i.e. the distance between the two wheels. Let h be the height of the centre of gravity of the vehicle above the ground. Let R₁ and R₂ be the reactions on the inner wheel and outer wheel of the vehicle exerted by the road surface.

The frictional force ‘F’ provides the necessary centripetal force.

Taking the moment of forces about the centre of gravity ‘G’

Fh  + R₁a = R₂a  ………… (2)

Reactions are balanced by the weight of the vehicle

R₁ + R₂ = mg …….. (3)

Solving equations |(1), (2) and (3)

From these equations, we can see that as the speed increases reaction R₁ decreases while reaction R₂ increases. When reaction R1 is zero overturnings of the vehicle takes place.

This is an expression for maximum velocity of the vehicle by which it can be driven beyond which overturning of the vehicle takes place.

Banked Road:

If μ < d/h, vehicle skids and if μ >d/h, vehicle overturns

Previous Topic: Problems on Centripetal and Centrifugal Force

Next Topic: Numerical Problems on Banking of Road

Science > Physics > Circular Motion > Banking of Road