Numerical Problems on Dopler Effect

Science > Physics > Wave Motion > Numerical Problems on Dopler Effect

In this article, we shall study to calculate the apparent frequency of a source using the Doppler effect.

Example – 01:

A whistle of frequency 420 Hz is sounded on the roadside. Find the apparent frequency of the whistle for a motorist driving at 60 km/h when approaching and receding. The velocity of sound in air =320 m/s.

Given: Actual Frequency of source = n = 420 Hz, Source stationary v_S = 0, Speed of listener = v_L = 60 km/h = 60 ×5/18 = 16.67 m/s, Velocity of sound in air = v = 320 m/s.

To Find: Apparent frequency = n_a =? when approaching and receding.

Solution:

Part – I: When the listener is approaching the stationary source

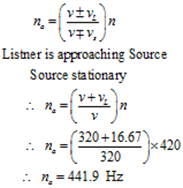
Part – II: When the listener is receding the stationary source

Ans: Apparent frequency when approaching = 441.9 Hz, Apparent frequency when receding = 398.1 Hz

Example – 02:

An engine sounding a whistle of frequency 2000Hz is receding from the stationary observer at 72 km/h. what is the apparent frequency for the observer? The velocity of sound in air =340 m/s

Given: Actual Frequency of source = n = 2000 Hz, Observer stationary V_L = 0, Speed of source = v_S = 72 km/h = 72 ×5/18 = 20 m/s, Velocity of sound in air = v = 340 m/s.

To Find: Apparent frequency = n_a = ? when receding.

Solution:

Ans: Apparent frequency when receding = 1889 Hz

Example – 03:

A source of sound having a frequency of 90 Hz is moving towards a stationary observer with a speed of 1/10ththat of sound. What is the apparent frequency of sound as heard by the observer?

Given: Actual Frequency of source = n = 90 Hz, Observer stationary V_L = 0, Speed of source = v_S = 1/10 v,

To Find: Apparent frequency = n_a = ? when approaching.

Solution:

Ans: Apparent frequency when approaching = 100 Hz

Example – 04:

A source of sound is approaching a stationary observer at 26.4 m/s if the frequency of the source is 512 Hz and the velocity of sound is 340 m/s, find the note as heard by the observer.

Given: Actual Frequency of source = n = 512 Hz, Observer stationary V_L = 0, Speed of source = v_S = 26.4 m/s, Velocity of sound in air = v = 340 m/s.

To Find: Apparent frequency = n_a = ? when approaching.

Solution:

Ans: Apparent frequency when approaching  = 555.1 Hz

Example – 05:

A locomotive passing a stationary observer at 30 m/s is sounding a whistle. Determine the ratio of the frequencies of the notes heard by the observer as the engine approaches and recedes. The velocity of sound in air =320m/s.

Given: Observer stationary V_L = 0, Speed of source = v_S = 30 m/s, Velocity of sound in air = v = 320 m/s.

To Find: Ratio of frequencies n_a approaching /n_a receding   =?

Solution:

Ans: Required ratio is 1.21:1

Example – 06:

The frequency of a sounding source is 1000Hz. An observer is moving at 20 m/s directly towards the stationary source. Find the apparent frequency for the observer. What would the apparent frequency be if the observer is at rest and the source is directly moving towards him at 20 m/s? Velocity of sound =340 m/s.

Given: Actual Frequency of source = n = 1000 Hz, Velocity of sound = v = 340 m/s. 

Part – I: Source stationary v_S = 0, Speed of listener = v_L = 20 m/s, Condition: Approaching.

Part – II: Listener stationary v_L = 0 , Speed of source = v_S = 20 m/s, Condition: Approaching.

To Find: Apparent frequency = n_a = ? when approaching and receding.

Solution:

Part – I: When the listener is approaching the stationary source

Part – II: When the source is approaching the stationary observer

Ans: Apparent frequency when source stationery and listener approaching = 1059 Hz and the apparent frequency when listener stationery and source approaching = 1062.5 Hz

Example – 07:

Two engines pass each other in opposite direction. One of the engines is blowing a whistle of frequency 540 Hz. Calculate the apparent frequency of the whistle for an observer on the other train as they approach and recede. The velocity of both engines is 40 m/s. The velocity of sound in air = 340 m/s.

Given: Speed of listener V_L = 40 m/s, Speed of source = v_S = 40 m/s, Velocity of sound in air = v = 340 m/s. Actual frequency of note = n = 540 Hz

To Find: Apparent frequency when approaching and receding   =?

Solution:

Ans: Apparent frequency when the two engines are approaching each other = 684 Hz and the apparent frequency when the two engines are receding each other = 426.3 Hz

Example – 08:

A train blows the whistle of frequency 640 Hz in air. Find the difference in apparent frequencies of the whistle for a stationary observer when the train moves towards and away from the observer with the speed 72 km/hr. The speed of sound in air is 340 m/s.

Given: Actual frequency of the note = 640 Hz, Speed of listener V_L =0, Velocity of sound in air = v = 340 m/s. Speed of the source = 72 km/h = 72 × 5/18 = 20 m/s.

To Find: n_{a(approaching)}  –  n_a(receding) =?

Solution:

n_{a(approaching)}  –  n_a(receding) =680 – 604.4 = 75.6 Hz

Ans:  The difference in apparent frequencies of the whistle for a stationary observer 75.6 Hz

Example – 09:

A stationary source produces a note of frequency 350 Hz. An observer in a car moving towards the source measures the frequency of sound at 370 Hz. Find the speed of the car. What will be the frequency of sound as measured by the observer in the car if the car moves away from the source at the same speed? Assume speed of sound = 340 m/s.

Given: Source is stationary V_S =0, Velocity of sound in air = v = 340 m/s. Actual frequency of note = n = 350 Hz, Apparent frequency of note when approaching = n_a = 370 Hz

To Find: Speed of car = V_L = ? apparent frequency when receding   =?

Solution:

Ans: Speed of the car is 19.43 m/s and the apparent frequency heard during receding is 330 Hz.

Example – 10:

The speed limit for a vehicle on a road is 100 km/h. A policeman detects a drop of 20% in the pitch of the horn of a car as it passes him. Is the policeman justified in punishing the car driver for crossing the speed limit? The velocity of sound in air = 340 m/s.

Given: Allowed speed = 100 km/h = 100 × 5/18 = 27.78 m/s, Speed of listener  V_L =0, Velocity of sound in air = v = 340 m/s. Drop in frequency when approaching and receding = 20 % = 0.2 i.e. n_a(receding) = n_{a(approaching)}  – 20% n_{a(approaching)}  = 0.8  n_{a(approaching)}

To Find: Justification for policeman

Solution:

Ans: The speed of the car is 37.77 m/s which is more than the allowed speed of 27.78 m/s. Hence policeman is justified in his action.

Example – 11:   

The speed limit for a vehicle on road is 120 km/ hr. A policeman detects a drop of 10 % in the pitch of horn of a car as it passes him. Is the policeman justified in punishing the car driver for crossing the speed limit? Given velocity of sound = 340 m/s.

Given: Allowed speed = 120 km/h = 120 × 5/18 = 33.33 m/s, Speed of listener  V_L =0, Velocity of sound in air = v = 340 m/s. Drop in frequency when approaching and receding = 10 % = 0.1 i.e. n_a(receding) = n_{a(approaching)}  – 10% n_{a(approaching)}  = 0.9  n_{a(approaching)}

To Find: Justification for policeman

Solution:

Ans: Now the speed of the car is 17.89 m/s which is less than the allowed speed of 33.33 m/s. Hence policeman is not justified in his action.

Previous Topic: Theory of Dopler Effect

For More Topics in Physics Click Here

Science > Physics > Wave Motion > Numerical Problems on Dopler Effect