Radius Ratio and its Significance

Science > Chemistry > Solid State > Radius Ratio and its Significance

The ratio of the radius of cations (r⁺) to the radius of the anion (r^–) is known as the radius ratio of the ionic solid.

The significance of radius ratio:

• It is useful in predicting the structure of ionic solids.
• The structure of an ionic compound depends upon stoichiometry and the size of ions.
• In crystals, cations tend to get surrounded by the largest possible number of anions around it.
• Greater the radius ratio, greater is the coordination number of cations and anions.
• If cations are extremely small and anions are extremely large, then the radius ratio is very small. In such case packing of anions is very close to each other and due to repulsion between anions, the system becomes unstable. Hence the structure changes to some suitable stable arrangement.
• The radius ratio at which anions just touch each other, as well as central cation, is called the critical radius ratio.

Effect of Radius Ratio on Coordination Number:

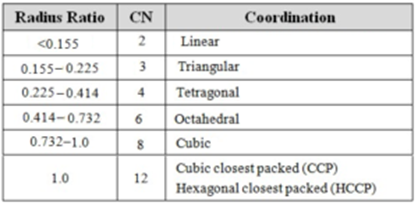
A cation would fit exactly into the octahedral void and would have a coordination number of six if the radius ratio were exactly 0.414. Similarly, a cation would fit exactly into the tetrahedral void and would have a coordination number of four, if the radius ratio were exactly 0.225.

Let us consider a case in which a cation is fitting exactly into the octahedral void of close pack anions and have the coordination number of six, in this case, the radius ratio is exactly 0.414.

When the radius ratio is greater than this, then the anions move apart to accommodate larger cation. This situation is relatively unstable. If the radius ratio is further increased the anions will move farther and farther apart till to reach a stage at which more anions can be accommodated. Now, the bigger cation moves to bigger void i.e. octahedral void whose coordination number is 8. This happens when the radius ratio exceeds 0.732.

In case of radius ratio becomes less than 0.414, the six anions will not be able to touch the smaller cation. To touch the cation, the anions starts overlapping with each other, which is an unstable situation. Hence smaller cation moves to smaller void i.e. tetrahedral void and coordination number decreases from 6 to 4.

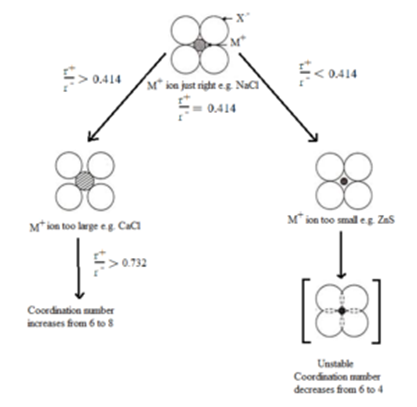
Note: Although a large number of ionic substances obey this rule, there are many exceptions to it.

Radius Ratio of Interstitial Voids:

The vacant space left in the closest pack arrangement of constituent particles is called an interstitial void or interstitial site.

Tetrahedral Voids:

Locating Tetrahedral Voids:

Let us consider a unit cell of ccp or fcc lattice [Fig. (a)]. The unit cell is divided into eight small cubes. Each small cube has atoms at alternate corners as shown. Each small cube has 4 atoms. When joined to each other, they make a regular tetrahedron as shown in the figure. Thus, there is one tetrahedral void in each small cube and eight tetrahedral voids in total in the unit cell. Each of the eight small cubes has one void in one unit cell of ccp structure. The ccp structure has 4 atoms per unit cell. Thus, the number of tetrahedral voids is twice the number of atoms.

Radius Ratio of Tetrahedral Void:

A tetrahedral site in a cube having a tetrahedral void of radius ‘r’ is as shown at the centre of the cube. Let ‘R’ be the radius of the constituent particle of the unit cell. Let ‘a’ be each side of the cube.

Consider face diagonal AB (Right-angled triangle ABC). We have,

AB² = a² + a²

∴  AB² = 2 a²

∴  AB² = √2 a

Now the two-sphere touch each other along face diagonal AB

∴  2R = √2 a

∴  R = √2 a/2

∴  R =  a/√2  ……. (1)

Consider body diagonal AD (Right-angled triangle ABD). We have,

AD² = AB² + BD²

∴  AD² = (√2 a)² + a²

∴  AD² = 2 a² + a² = 3 a²

∴  AD = √3 a

Now the two spheres at the diagonals touch the tetrahedral void sphere along body diagonal AD

∴  2 R + 2r  = √3 a

∴  R + r  = (√3/2)a  ……..  (2)

Dividing equation (2) by (1)

Thus the radius ratio for the tetrahedral void is 0.225

Octahedral Voids:

Locating Octahedral Voids:

Let us consider a unit cell of ccp or fcc lattice [Fig. (a)]. The body centre of the cube, C is not occupied but it is surrounded by six atoms on face centres. If these face centres are joined, an octahedron is generated. Thus, this unit cell has one octahedral void at the body centre of the cube.

Besides the body centre, there is one octahedral void at the centre of each of the 12 edges. [Fig. (b)]. It is surrounded by six atoms, four belonging to the same unit cell (2 on the corners and 2 on face centre) and two belonging to two adjacent unit cells.

Since each edge of the cube is shared between four adjacent unit cells, so is the octahedral void located on it. Only 1/4 th of each void belongs to a particular unit cell.

For cubic close-packed structure:
Number of octahedral void at the body-centre of the cube = 1
12 octahedral voids located at each edge and shared between four unit cells

Thus number of octahedral valve at 12 edges =12 x 1/4 = 3
Thus, the total number of octahedral voids in unit cell = 1 + 3 = 4

In the ccp structure, each unit cell has 4 atoms. Thus, the number of octahedral voids is equal to this number of atoms.

Radius Ratio of Octahedral Void:

A octahedral site in a cube having octahedral void of radius r is as shown at the centre of the cube. Let R be the radius of the constituent particle of the unit cell. Let a be each side of the cube. In this case we can see that a = 2R

Consider Right angled triangle ABC. We have,

AB² = AC² + CB²

∴  (2R + 2r)² = a² + a²

∴  (2R + 2r)² = 2 a²

∴  2R + 2r = √2 a

But from figure a = 2R

∴  2R + 2r = √2 x 2R

∴   R +  r = √2  R

∴   r = √2  R – R

∴   r = (√2  – 1)R

∴   r = (1.414  – 1)R

∴   r = 0.414R

Thus radius ratio for the octahedral void is 0.414

Science > Chemistry > Solid State > Radius Ratio and its Significance