Management > Managerial Statistics > Index Number By Kelly’s Method and Walsh’s Method
Kelly’s Method:
Truman L. Kelly has suggested the following formula for constructing index number. Here weights are the quantities which may refer to some period, not necessarily the base year or current year. Thus the average quantity of two or more years may be used as weights. This method is known as a fixed-weighted aggregative index and is currently in great favour n the construction of index number series.
Steps Involved:
- Denote prices of the commodity in the current year as P1 and its quantity consumed in that year by Q1.
- Denote prices of the commodity in the base year as P0 and its quantity consumed in that year by Q0.
- Find q = (Q0 + Q1)/2 for each commodity
- Find the quantities P0q and P1q for each commodity.
- Find sum of each column of P0q and P1q and denote the sums by ∑ P0q and ∑ P1q respectively.
- Use following formula to find the Price index number

Example – 01:
Compute Price index by Kelly’s Method from the following data.
|
Commodity |
Base Year |
Current Year |
||
|
|
Price |
Quantity |
Price |
Quantity |
|
A |
3 |
25 |
5 |
28 |
|
B |
1 |
50 |
3 |
60 |
|
C |
2 |
30 |
1 |
30 |
|
D |
5 |
15 |
6 |
12 |
Solution:
|
Commodity |
Base Year |
Current Year |
|||||
|
|
P0 |
Q0 |
P1 |
Q1 |
q=(Q0+Q1)/2 |
P0q |
P1q |
|
A |
3 |
25 |
5 |
28 |
16.5 |
49.5 |
82.5 |
|
B |
1 |
50 |
3 |
60 |
31.5 |
31.5 |
94.5 |
|
C |
2 |
30 |
1 |
30 |
31 |
31 |
15.5 |
|
D |
5 |
15 |
6 |
12 |
45 |
45 |
54 |
|
Total |
∑P0q=157 |
∑P1q=246.5 |
|||||
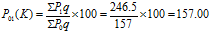
By Kelly’s method the price index number is 157.00
Walsh’s Method:
Here weights are the geometric mean of quantities of two or more years may be used as weights.
Steps Involved:
- Denote prices of commodity in the current year as P1 and its quantity consumed in that year by Q1.
- Denote prices of commodity in the base year as P0 and its quantity consumed in that year by Q0.
- Find q = (Q0 x Q1)1/2 = Square root of (Q0 x Q1) for each commodity
- Find the quantities P0q and P1q for each commodity.
- Find sum of each column of P0q and P1q and denote the sums by ∑ P0q and ∑ P1q respectively.
- Use following formula to find the Price index number
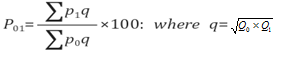
Example – 02:
Compute Price index by Walsh’s Method from the following data.
|
Commodity |
Base Year |
Current Year |
||
|
|
Price |
Quantity |
Price |
Quantity |
|
A |
3 |
25 |
5 |
28 |
|
B |
1 |
50 |
3 |
60 |
|
C |
2 |
30 |
1 |
30 |
|
D |
5 |
15 |
6 |
12 |
Solution:
|
Commodity |
Base Year |
Current Year |
|||||
|
P0 |
Q0 |
P1 |
Q1 |
q=(Q0 x Q1)1/2 |
P0q |
P1q |
|
|
A |
3 |
25 |
5 |
28 |
26.46 |
79.37 |
132.29 |
|
B |
1 |
50 |
3 |
60 |
54.77 |
54.77 |
164.32 |
|
C |
2 |
30 |
1 |
30 |
30.00 |
60.00 |
30.00 |
|
D |
5 |
15 |
6 |
12 |
13.42 |
67.08 |
80.50 |
|
Total |
∑P0q=261.23 |
∑P1q=407.10 |
|||||

Thus Walsh’s price index number is 155.84
An important advantage of these formulae is that like Laspeyres index it does not demand yearly changes in the weights. Moreover, the base period can be changed without necessitating a corresponding change in the weights. This is very important because the construction of appropriate quantity weights for a general propose index usually requires a considerable amount of work.
Previous Topic: Marshall Edgeworth Method
Next Topic: Test of Adequacy of Index Number
One reply on “Kelly’s Method and Walsh Method”
This is really good and detailed to be sincere, both the examples and practical solutions. It’s helpful