Atomic radius: Definition, factors affecting it, trends in a period and group

In this article, we shall discuss a very important periodic property called the atomic radius.

Atomic Radius:

The size of an atom is very small (120 pm). Secondly, since the electron cloud surrounding the atom does not have a sharp boundary, the determination of the atomic size cannot be precise. One practical approach of finding the size of an atom of a non-metallic element is to measure the distance between two atoms when they are bound together by a single bond in a covalent molecule and from this value, the “Covalent Radius” of the element can be calculated.

The atomic radius (atomic size) may be regarded as the distance from the centre of the atom to the outermost (valence) shell of electrons.

The electron density in an atom is greatly influenced by the presence of other atoms around the bonding atom and the nature (type) of bonding with neighbouring atoms, Depending upon this the terms like crystal radius, covalent radius, van der Walls’ radius, tetrahedral radius, etc. are used. Hence the definition given in above point is arbitrary.

Other Terms Related to Atomic Radius:

Crystal Radius:

It is defined as one half of the distance between the centres of nuclei of two adjacent atoms in a metallic crystal. For e.g. the distance between two sodium atoms in a sodium crystal is 372 pm. Hence crystal radius of sodium = 372 / 2 = 186 pm.

Covalent Radius:

Covalent radius is defined as one half of the distance between the centres of the two similar nuclei of two similar atoms bonded together by a single covalent bond. For e.g. the distance between two oxygen atoms in molecular oxygen is 132 pm. Hence crystal radius of oxygen = 132 / 2 = 66 pm. Covalent radii are additive. This property can be used to find the internuclear distance between two molecules forming a single covalent bond among themselves.

van der Walls’ Radius:

van der Walls’ radius is defined as one half of the distance between the nuclei of the two atoms of the same substance at their closest approach. For e.g. the closest distance between two hydrogen atoms without forming the bond is 240 pm. Hence van der Walls’ radius of sodium = 240 / 2 = 120 pm. Generally, atomic radii of inert gases are expressed in terms of van der Walls’ radius. van der Wall’s forces are weaker hence the distance between the atoms is larger.

The covalent radius is the smallest of all the radii because covalent bonds are formed due to overlapping of orbitals and there is penetration of one atom in another.

Factors Affecting Atomic Size:

Number of Shells:

Atomic size increases with the increase in the number of electronic shells. Thus atomic radius is directly proportional to the number of electronic shells.

Nuclear Charge:

As the nuclear charge increases the atomic radius decreases due to an increase in the attractive force on the outermost electrons. Thus atomic radius is inversely proportional to the nuclear charge.

Screening Effect:

In an atom having more electrons and particularly more electron shells, it is observed that the inner orbits decrease the attraction between the electrons in the outer orbit and nucleus. Thus they act as a screen or shield between the nucleus and electrons of outer orbit. This effect is known as the screening effect. As the screening effect increases, the atomic radius increases. Thus atomic radius is directly proportional to the screening effect. For a given quantum shell, the shielding ability of inner electrons decreases in the order of s > p > d > f.

Effective nuclear charge: The effective nuclear charge is the difference between the actual nuclear charge and the screening effect constant.charge. Z_eff = Z – σ. The atomic radius is inversely proportional to the effective nuclear charge.

Periodic Trend in Atomic Radius Along the Period:

The atomic radii of the elements of the second period and the graphical representation of variation for the second period are given below.

Elements	Symbol	Atomic Number	Electronic Configuration	Number of Shells	Nuclear Charge	Atomic Radius (pm)
Lithium	Li	3	[He]2s¹	2	+3	152
Beryllium	Be	4	[He]2s²	2	+4	111
Boron	B	5	[He]2s²2p¹	2	+5	88
Carbon	C	6	[He]2s²2p²	2	+6	77
Nitrogen	N	7	[He]2s²2p³	2	+7	74
Oxygen	O	8	[He]2s²2p⁴	2	+8	66
Fluorine	F	9	[He]2s²2p⁵	2	+9	64

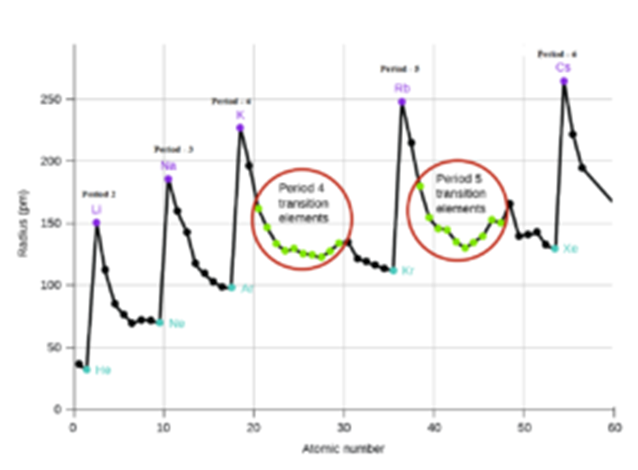
The atomic radii of the elements of the third period are given below.

Elements	Symbol	Atomic number	Electronic configuration	Number of shell	Nuclear Charge	Atomic Radius (pm)
Sodium	Na	11	[Ne]2s¹	3	+11	186
Magnesium	Mg	12	[Ne]2s²	3	+12	160
Aluminium	Al	13	[Ne]2s²2p¹	3	+13	143
Silicon	Si	14	[Ne]2s²2p²	3	+14	117
Phosphorus	P	15	[Ne]2s²2p³	3	+15	110
Sulphur	S	16	[Ne]2s²2p⁴	3	+16	104
Chlorine	Cl	17	[Ne]2s²2p⁵	3	+17	99

The trend for ‘s’ block and ‘p’ block elements:

The atomic size generally decreases across a period.

Explanation:

As we move from left to right in a period atomic number increases, hence the nuclear charges increases. The valence electrons are added to the same orbit of all the elements in the same period, hence screening effect and number of shells are the same. The effective nuclear charge increases across the period. Hence the attractive force on the electrons in the outermost shell increases. Hence the atomic radius decreases.

Thus in a period alkali metals have the largest atomic radius and it gradually decreases across the period and it is minimum for the halogen elements. The inert gases have the largest atomic radii in the period because for them van der Wall’s radii are considered. Radii of aluminium and gallium are equal in spite of the fact they belong to the same group. This is due to the presence of then d – block elements. screening power of d elements is less.

The trend for ‘d’ block elements:

In case of d block elements as we move from left to right across the period, atomic number increases. The nuclear charge increases. But the electrons are added to penultimate i.e. (n-1) shell, hence the electron cloud density of inner shells increases which increases the screening effect. Thus nuclear charge increases and screening effect increases. Hence there is decreases in the atomic radius but the extent of variation is very small compared to s block and p block elements. The extent of variation is so small that all of them can be considered to have almost equal atomic radii.

The trend for ‘f’ block elements:

In case of ‘f’ block elements as we move from left to right across the period, atomic number increases. The nuclear charge increases. But the electrons are added to pre-penultimate i.e. (n-2) shell, hence the electron cloud density of inner shells increases which increases the screening effect strongly. Thus nuclear charge increases and screening effect increases. The effect of nuclear charge is almost balanced by the screening effect. Hence there is a very small decrease in the atomic radius compared to d block elements. Hence the properties of ‘f’ block elements are almost similar. This type of contraction among the first and second series of ‘f’ block elements is called lanthanide contraction.

Periodic Trend in Atomic Radius Along the Group:

Trend: The atomic size increases down the group.

Explanation: As we move down the group atomic number increases, hence the nuclear charges increases. The number of shells increases hence screening effect increases. Hence the attractive force on the electrons in the outermost shell decreases. Hence the atomic radius increases.

The atomic radii of the elements of the group 1 are given below.

Elements	Symbol	Atomic number	Electronic configuration	Number of Shells	Nuclear Charge	Atomic Radius (pm)
Hydrogen	H	1	1s¹	1	+1	53
Lithium	Li	3	[He]2s¹	2	+3	152
Sodium	Na	11	[Ne]3s¹	3	+11	186
Potassium	K	19	[Ar]4s¹	4	+19	231
Rubidium	Rb	37	[Kr]5s¹	5	+37	244
Caesium	Cs	55	[Xe]6s¹	6	+55	262

The atomic radii of the halogens elements of the group 17 are given below.

Elements	Symbol	Atomic number	Electronic configuration	Number of Shells	Nuclear Charge	Atomic Radius (pm)
Fluorine	F	9	[He]2s²2p⁵	2	+9	72
Chlorine	Cl	17	[Ne]3s²3p⁵	3	+17	99
Bromine	Br	35	[Ar]3d¹⁰4s²4p⁵	4	+35	114
Iodine	I	53	[Kr]4d¹⁰5s²5p⁵	5	+53	133
Astetine	At	85	[Kr]4f¹⁴5d¹⁰6s²6p⁵	6	+85	140