Packing Efficiency of Unit Cell

Science > Chemistry > Solid State > Packing Efficiency of Unit Cell

In this article, we shall study the packing efficiency of different types of unit cells.

In whatever way the constituent particles atoms, molecules or ions are packed, there is always some free space in the form of voids.

The fraction of the total space in the unit cell occupied by the constituent particles is called packing fraction. Mathematically

Packing efficiency is the percentage of total space filled by the constituent particles in the unit cell.

Packing efficiency = Packing Factor x 100

A vacant space not occupied by the constituent particles in the unit cell is called void space.

The fraction of void space = 1 – Packing Fraction
% Void space = 100 – Packing efficiency.

Packing Efficiency of Simple Cubic Crystal Lattice (SCC):

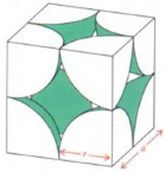
In a simple cubic lattice, the atoms are located only on the corners of the cube. The particles touch each other along the edge as shown. Thus, the edge length (a) or side of the cube and the radius (r) of each particle are related as a = 2r. Thus the radius of an atom is half the side of the simple cubic unit cell.

The volume of the cubic unit cell = a³ = (2r)³ = 8r³

Since a simple cubic unit cell contains only 1 atom.

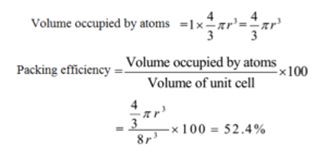
The packing efficiency of the simple cubic cell is 52.4 %. Thus 47.6 % volume is empty space (void space) i.e. almost half the space is empty. Hence the simple cubic crystalline solid is loosely bonded.

Packing Efficiency of Body Centred Cubic Crystal Lattice (BCC):

In a body-centred cubic lattice, the eight atoms are located on the eight corners of the cube and one at the centre of the cube. Let the edge length or side of the cube ‘a’, and the radius of each particle be r. The particles along the body diagonal touch each other.

∴ AD = r  + 2r + r = 4r   ……. (1)

Thus the radius of an atom is √3/₄ times the side of the body-centred cubic unit cell.

The volume of the cubic unit cell

Since a body-centred cubic unit cell contains 2 atoms

The packing efficiency of the body-centred cubic cell is 68 %. Thus 32 % volume is empty space (void space).

Packing Efficiency of Face Centred Cubic Crystal Lattice (FCC):

In a face-centred cubic lattice, the eight atoms are located on the eight corners of the cube and one at the centre of the cube. Let the edge length or side of the cube ‘a’, and the radius of each particle be r. The particles along face diagonal touch each other.

∴ AB = r + 2r + r = 4r  ……. (1)

Thus the radius of an atom is 1 / _√8   times the side of the face centred cubic unit cell.

The volume of the cubic unit cell

Since a face centred cubic unit cell contains 4 atoms,

The packing efficiency of the face centred cubic cell is 74 %. Thus 26 % volume is empty space (void space).

Summary of the Three Types of Cubic Structures:

Type of Unit Cell	Number of atoms in unit cell	coordination number	Relation Between a and r	Packing Efficiency	Void Space
Simple cubic (scc)	1	6	a = 2r	52.4 %	47.6 %
Body centred cubic (bcc)	2	8	a = (4/√3)r	68 %	32 %
Face centred cubic (fcc)	4	12	a = 2√2 r	74 %	26 %

Nearest Neighbouring Atoms:

Type of Lattice	First nearest	Second nearest	Third nearest
Simple cubic	a Along the edge of lattice	a√2 Along the face diagonal	a√3 Along the body diagonal
Body centred	(√3/2) a Along the body diagonal	a Along the edge of lattice	a√2 Along the face diagonal
Face centred	a/√2 Along the face diagonal	a Along the edge of lattice	a√2 Along the face diagonal

Note:

From the unit cell dimensions, it is possible to calculate the volume of the unit cell. Knowing the density of the metal, we can calculate the mass of the atoms in the unit cell. The determination of the mass of a single atom gives an accurate method of determination of Avogadro constant.

Steps involved in finding the density of a substance:

• Find the volume of the unit cell using formula Volume = a³
• Find the type of cubic cell. Find the number of particles (atoms or molecules) in that type of cubic cell. Let it be denoted by ‘n’
• Find the mass of one particle (atoms or molecules) using formula

Mass of one particle = Molar (Atomic) mass of substance / Avogadro’s number

Mass of one particle = M/N

Where M = Molecular mass of the substance

N = Avogadro’s number = 6.022 x 10^-23 mol^-1.

• Find the mass of each unit cell using formula

Mass of unit cell = Mass of each particle x Number of particles in the unit cell

Mass of unit cell = (M/N) x n = nM/N

• Find the density of the substance using the formula

Steps involved in finding the radius of an atom:

• Find the type of cubic cell. Find the number of particles (atoms or molecules) in that type of cubic cell.  Let it be denoted by ‘n.
• ‘Find molar mass of one particle (atoms or molecules) using formula
• Find the mass of one particle (atoms or molecules) using formula

Mass of one particle = Molar (Atomic) mass of substance / Avogadro’s number

Mass of one particle = M/N

Where M = Molecular mass of the substance

N = Avogadro’s number = 6.022 x 10²³ mol^-1.

• Find the mass of each unit cell using formula

Mass of unit cell = Mass of each particle x  Number of particles in the unit cell

Mass of unit cell = (M/N) x n = nM/N

• Find the length of the side of the unit cell

• Write the relation between a and r for the given type of crystal lattice and calculate r.

Steps involved in finding atomic mass:

• Find the volume of the unit cell using formula Volume = a³
• Find the type of cubic cell. Find the number of particles (atoms or molecules) in that type of cubic cell. Let it be denoted by ‘n’
• Find the value of M/N from the following formula.

Now M/N gives the atomic mass

Science > Chemistry > Solid State > Packing Efficiency of Unit Cell