Tetrahedral Voids and Octahedral Voids

Science > Chemistry > Solid State > Tetrahedral Voids and Octahedral Voids

In this article, we shall study two types of voids formed during hexagonal closed packing namely, a) Tetrahedral voids and b) octahedral voids.

Let us consider a closed pack hexagonal packing in the first layer as shown. There are empty spaces between the particles (sphere) are called voids.

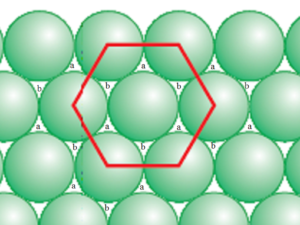
All the voids are equivalent in the first layer they have marked alternately as ‘a’ and ‘b’. The spheres of the second layer can be either placed on voids which are marked ‘a’ or ‘b’ but it is impossible to place spheres on both types of voids simultaneously. When spheres of the new layer are placed on voids marked ‘a’ then voids marked as ‘b; remain unoccupied.

Thus there is no void above ‘a’ in the second layer but there is void above ‘b’ even in the second layer. The void between the first layer and second layer at ‘a’ is tetrahedral void, while the void between the first layer and the second layer at ‘b’ is octahedral void.

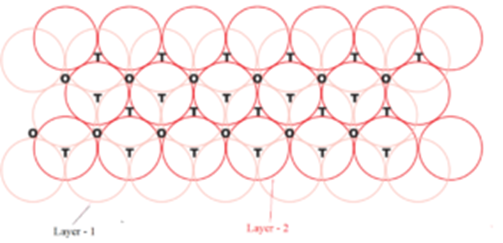
T = Tetrahedral void and O = Octahedral void

Tetrahedral Voids:

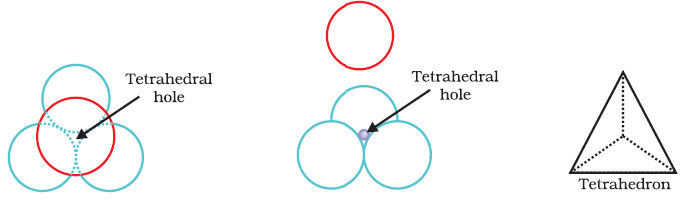
Wherever a sphere of the second layer is above the void of the first layer (or vice versa) a tetrahedral void is formed. These voids are called tetrahedral voids because a tetrahedron is formed when the centres of these four spheres are joined. These voids have been marked as ‘T’ in the figure.

Octahedral Voids:

At other places, the triangular voids in the second layer are above the triangular voids in the first layer, and the triangular shapes of these do not overlap then the octahedral void is formed. One of them has the apex of the triangle pointing upwards and the other downwards. These voids have been marked as ‘O’ in the figure.

Number of Voids:

The number of these two types of voids depends upon the number of close-packed spheres.

Let the number of close-packed spheres be N, then, the number of octahedral voids generated = N and the number of tetrahedral voids generated = 2N

Characteristics of Tetrahedral Voids:

• The vacant space or void is surrounded by four atomic spheres. Hence co-ordination number of the tetrahedral void is 4.
• The atom in the tetrahedral void is in contact with four atoms placed at four corners of a tetrahedron.
• This void is formed when a triangular void made coplanar atoms (first layer) is in contact with the fourth atom above or below it (second layer).
• The volume of the void is much smaller than that of the spherical particle.
• If R is the radius of the constituent spherical particle, then the radius of the tetrahedral void is 0.225 R.
• If the number of close-packed spheres is N, then the number of tetrahedral voids is 2N.

Characteristics of Octahedral Voids:

• The vacant space or void is surrounded by six atomic spheres. Hence, the coordination number of the tetrahedral void is 6.
• The atom in the octahedral void is in contact with six atoms placed at six corners of an octahedron.
• This void is formed when two sets of equilateral triangles pointing in the opposite direction with six spheres.
• The volume of the void is small.
• If R is the radius of the constituent spherical particle, then the radius of the octahedral void is 0.414 R.
• If the number of close-packed spheres is N, then the number of octahedral voids is N.Characteristics of Voids

Packing Voids in Ionic Solids

We have already seen that when particles are close-packed resulting in either cubic closed packing (ccp) or hexagonal close packing (hcp) structure, two types of voids are generated. The number of octahedral voids present in a lattice is equal to the number of close-packed particles, the number of tetrahedral voids generated is twice this number.

Ionic solids are formed from cations and anions. The charge is balanced by an appropriate number of both types of ions so that net charge on solid is zero (neutral).

In ionic solids, the bigger ions (usually, anions) form the close-packed structure and the smaller ions (usually, cations) occupy the voids. If the smaller ion is small enough then tetrahedral voids are occupied, if bigger, then octahedral voids are occupied.

Not all octahedral or tetrahedral voids are occupied. In a given compound, the fraction of octahedral or tetrahedral voids that are occupied, depends upon the chemical formula of the compound, as can be seen from the following examples.

Example 1:

A compound is formed by two elements X and Y. Atoms of the element Y (as anions) make cubic closed packing (ccp) and those of the element X (as cations) occupy all the octahedral voids. Obtain the formula of the compound.

Solution:

Given that the cubic closed packing (ccp) lattice is formed by the element Y.

The number of octahedral voids generated would be equal to the number of atoms of Y present in it.

Since all the octahedral voids are occupied by the atoms of X, their number would also be equal to that of the element Y. Thus, the atoms of elements X and Y are present in equal numbers or 1:1 ratio.

Therefore, the formula of the compound is XY.

Example 2:

Atoms of element B form hexagonal close packing (hcp) lattice and those of element A occupy 2/3rd of tetrahedral voids. Obtain the formula of the compound formed by the elements A and B.

Solution:

The number of tetrahedral voids formed is equal to twice the number of atoms of element B

Only 2/3rd of these are occupied by the atoms of element A. Hence the ratio of the number of atoms of A and B is 2 × (2/3):1 or 4:3

Hence the formula of the compound is A₄B₃.

Science > Chemistry > Solid State > Tetrahedral Voids and Octahedral Voids