In this article, we shall study to find the displacement, velocity, and acceleration of a particle, by the calculus method.
Example – 01:
A particle is moving in such a way that is displacement ’s’ at any time ‘t’ is given by s = 2t2 + 5t +20. Find the velocity and acceleration of the particle after 2 seconds.
Solution:
The displacement of the particle is given by
s = 2t2 + 5t +20 ……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 4t + 5 ……………… (2)
Differentiating both sides of equation (2) w.r.t. t
Acceleration = a = dv/dt = 4 ……………… (3)
To find velocity after 2 seconds i.e. t = 2 s
Substituting in equation (2)
Velocity = v = 4(2) + 5 = 8 + 5 = 13 unit/s
To find acceleration after 2 seconds i.e. t = 2 s
Acceleration = a = 4 units/s2
Ans: The velocity and acceleration of the particle after 2 seconds are 13 units/s and 4 units/s2 respectively.
Example – 02:
The displacement ‘s’ of a particle at a time ‘t’ is given by s = 5 + 20t – 2t2. Find its acceleration when its velocity is zero.
Solution:
The displacement of a particle is given by
s = 5 + 20t – 2t2 ……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 20 – 4t ……………… (2)
Differentiating both sides of equation (2) w.r.t. t
Acceleration = a = dv/dt = – 4 ……………… (3)
Given velocity is zero
20 – 4t = 0
4t = 20
T = 5 s
To find acceleration after 5 seconds i.e. t = 5 s
Acceleration = a = – 4 units/s2
Ans: The acceleration of the particle after 5 seconds is – 4 units/s2
Example – 03:
A particle is moving in such a way that is displacement’s’ at any time ‘t’ is given by s = t3 – 4t2 – 5t. Find the velocity and acceleration of the particle after 2 seconds.
Solution:
The displacement of the particle is given by
s = t3 – 4t2 – 5t ……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 3t2 -8t -5 ……………… (2)
Differentiating both sides of equation (2) w.r.t. t
Acceleration = a = dv/dt = 6t – 8 ……………… (3)
To find velocity after 2 seconds i.e. t = 2 s
Substituting in equation (2)
Velocity = v = 3(2)2 -8(2) -5 = 12 – 16 – 5 = – 9 unit/s
To find acceleration after 2 seconds i.e. t = 2 s
Acceleration = a = 6(2) – 8 = 12 – 8 = 4 units/s2
Ans: The velocity and acceleration of the particle after 2 seconds are – 9 units/s and 4 units/s2 respectively.
Example – 04:
A particle is moving in such a way that is displacement’s’ at any time ‘t’ is given by s = 2t3 – 5t2 + 4t – 3. Find the time when acceleration is 14 ft/s2. Also, find velocity and displacement at that time.
Solution:
The displacement of the particle is given by
s = 2t3 – 5t2 + 4t – 3……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 6t2 – 10t + 4 ……………… (2)
Differentiating both sides of equation (2) w.r.t. t
Acceleration = a = dv/dt = 12t – 10 ……………… (3)
Given acceleration = a = 14 ft/s2
Substituting in equation (3)
12t – 10 = 14
12t = 24
T = 2 s
Substituting t = 2 in equation (2)
Velocity = v = 6t2 – 10t + 4 = 6(2)2 – 10(2) + 4 = 24 – 20 + 4 = 8 ft/s
Substituting t = 2 in equation (1)
Displacement = s = 2(2)3 – 5(2)2 + 4(2) – 3 = 16 – 20 + 8 – 3 = 1 ft
Ans: After 4 s the acceleration will be 14 ft/s2
The velocity is 8 ft/s and displacement is 1 ft
Example – 05:
A particle moves according to law s = t3 – 6t2 + 9t + 15, find the velocity when t = 0
Solution:
The displacement of the particle is given by
s = t3 – 6t2 + 9t + 15 ……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 3t2 – 12t + 9 ……………… (2)
To find velocity at t = 0
Substituting t = 0 in equation (2)
Velocity = v = 3t(0)2 – 12t(0) + 9 = 9 units/s
Ans: The velocity at t = 0 is 9 units/s
Example – 06:
A particle moves under the law s = t3 – 4t2 – 5t. Find the displacement and velocity of particle when its acceleration is 4 units.
Solution:
The displacement of the particle is given by
s = t3 – 4t2 – 5t ……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 3t2 – 8t – 5 ……………… (2)
Differentiating both sides of equation (2) w.r.t. t
Acceleration = a = dv/dt = 6t – 8 ……………… (3)
Given acceleration = a = 4 units
Substituting in equation (3)
6t – 8 = 4
6t = 12
t = 2 s
Substituting t = 2 in equation (1)
Displacement = s = (2)3 – 4(2)2 – 5(2) = 8 – 16 – 10 = – 18 units
Substituting t = 2 in equation (2)
Velocity = v = 3(2)2 – 8(2) – 5 = 12 – 16 – 5 = – 9 units/s
Ans: When acceleration is 4 units displacement is -18 units and velocity is – 8 units/s
Example – 07:
A particle moves under the law s = t3/3 – t2/2 – t/2 +6. Find (i) its velocity at end of 4 s and (ii) acceleration and displacement when its velocity is 3/2 units.
Solution:
The displacement of the particle is given by
s = t3/3 – t2/2 – t/2 +6……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 3t2/3 – 2t/2 – 1/2 = t2 – t – 1/2 ……………… (2)
Differentiating both sides of equation (2) w.r.t. t
Acceleration = a = dv/dt = 2t – 1 ……………… (3)
(i) To find its velocity at end of 4 s, t = 4 s
Substituting t = 4 in equation (2)
Velocity = v = (4)2 – (4) – 1/2 = 16 – 4 – 1/2 = 11.5 units/s
(ii) To find acceleration and velocity when its velocity is 3/2 units.
t2 – t – 1/2 = 3/2
t2 – t – 2 = 0
(t – 2)(t + 1) = 0
t – 2 = 0 and t + 1 = 0
t = 2 and t = – 1
Time cannot be negative hence t = -1 not possible
t = 2 s
Substituting t = 2 in equation (3)
Acceleration = 2(2) – 1= 3 units/s2
Substituting t = 2 in equation (1)
Displacement = s = (2)3/3 – (2)2/2 – (2)/2 +6 = 8/3 – 2 – 1 + 6 = 17/3 units
Ans: Velocity at end of 4 s is 11.5 units/s
When velocity is 3/2 units, acceleration is 3 units/s2 and displacement is 17/3 units
Example – 08:
The displacement x of a particle at time t is given by x = 160 t – 16t2, show that its velocity at t = 1 and t =9 are equal in magnitude and opposite in direction.
Solution:
The displacement of the particle is given by
s = 160 t – 16t2……………… (1)
Differentiating both sides of equation (1) w.r.t. t
Velocity = v = ds/dt = 160 – 32t ……………… (2)
Velocity at t = 1
Velocity = 160 – 32(1) = 128 units/s
Velocity at t = 9
Velocity = 160 – 32(9) = – 128 units/s
We can seet hat the velocities at t = 1 and t =9 are equal in magnitude and opposite in direction. (Proved)
Example – 09:
A particle is moving in a straight line and its displacement x from a fixed point O on the line at time t is given by ![]() . Show that the acceleration at time t is x-3.
. Show that the acceleration at time t is x-3.
Solution:
The displacement of the particle is given by
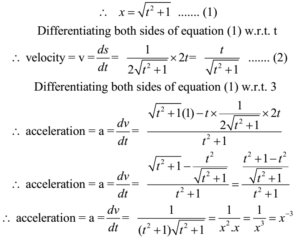
Thus acceleration at time t is x-3 (Proved as required)
One reply on “Displacement, Velocity, and Acceleration”
nice @#