The Inclination of a Line

Science > Mathematics > Coordinate Geometry > Straight Lines > The Inclination of a Line

In this article, we shall study the concept of inclination of a line and the slope of the line

The inclination of a Line:

A line in a coordinate plane forms two angles with the x-axis, which are supplementary.  The angle (say) θ made by the line l with the positive direction of the x-axis and measured anti-clockwise is called the inclination of the line. Thus 0° ≤ θ ≤ 180°.

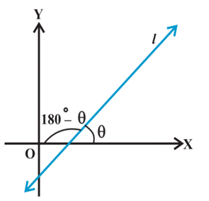
• The lines parallel to the x-axis, or the coinciding with the x-axis, have the inclination of 0°.
• The inclination of a vertical line (parallel to or coinciding with y-axis) is 90°.

The slope of a Line:

If θ is the inclination of a line l, then tan θ, (θ ≠ 90°) is called the slope or gradient of the line l. Note that the slope of a line whose inclination is 90° is not defined. The slope of a line is denoted by the letter, ‘m’. Thus by definition m = tan θ, θ ≠ 90°

• The slope of the x-axis is zero and the slope of the y-axis is not defined

Equation for Slope of a Line:

Let P(x₁, y₁) and Q(x₂, y₂) be two points on non-vertical line l whose inclination is θ. As the line is vertical, x₁ ≠ x₂, The inclination of the line l may be acute or obtuse. Let us consider both of these cases.

Case – 1: When the inclination is acute

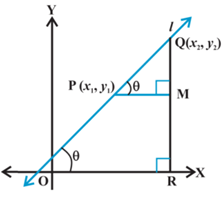
Draw perpendicular QR to the x-axis and PM perpendicular to RQ as shown

MQ = y₂ – y₁ and MP = x₂ – x₁∠MPQ = θ ………… (1)

Therefore, the slope of line l = m = tan θ.

But in ∆MPQ, we have

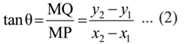
∴ Slope of line l = (y₂ – y₁)/(x₂ – x₁)

Case – 2: When the inclination is obtuse

Draw perpendicular QR to the x-axis and PM perpendicular to RQ as shown

MQ = y₂ – y₁ and MP = x₁ – x₂∠MPQ =  π – θ

Therefore, the slope of line l = m = tan θ = – tan (π – θ).

But in ∆MPQ, we have

∴ Slope of line l = (y₂ – y₁)/(x₂ – x₁)

Thus, in either case, the slope of the line is m = (y₂ – y₁)/(x₂ – x₁)

Sign of Slope:

The slope can be positive, zero or negative

• Positive slope: This means the angle of inclination θ is such that 0° < θ < 90°. i.e. the angle of inclination is acute.
• Zero slope: This means the line is parallel to the x-axis.
• Negative slope: This means the angle of inclination θ is such that 90° < θ < 180°. i.e. the angle of inclination is obtuse.
• If the line is perpendicular to the x-axis its slope is not defined.

Conditions for parallelism of lines in terms of their slopes

In a coordinate plane, suppose that non-vertical lines l₁ and l₂ have slopes m₁ and m₂, respectively. Let their inclinations be α and β, respectively.

If the line l₁ is parallel to l₂ (Fig 10.4), then their inclinations are equal,

i.e., α = β, and hence, tan α = tan β

Therefore m₁ = m₂, i.e., their slopes are equal.

Conversely, if the slope of the two lines l₁ and l₂ are the same, i.e., m₁ = m₂.
By the property of tangent function (between 0° and 180°), α = β. Therefore, the lines are parallel. Hence, two non-vertical lines l₁ and l₂ are parallel if and only if their slopes are equal

Conditions for perpendicularity  of lines in terms of their slopes:

If the lines l₁ and l₂ are perpendicular,  then by exterior angle property, 

β = α + 90°.

∴  tan β = tan (α + 90°)

∴  tan β = – cotα

∴  tan β = –  1/ tan α

∴  tanα . tan β = –  1

∴  m₁ . m₂ = – 1

Conversely, if m₁ m₂ = – 1, i.e., tan α tan β = – 1.

Then tanα = – cot β = tan (β + 90°) or tan (β – 90°)

Therefore,α and β differ by 90°.

Thus, lines l₁ and l₂ are perpendicular to each other.

Hence, two non-vertical lines are perpendicular to each other if and only if their slopes are negative reciprocals of each other, or the product of their slopes is – 1.

Slope of Line from Inclination of a Line:

Tangent Functions of Some Angles:

Angle	15°	30°	45°	60°	75°	105°	120°	135°	150°
tanθ	2 – 2√3	1/√3	1	√3	2 + √3	-(2 + √3)	– √3	– 1	– 1/√3

Example – 01:

Find the slope of lines whose inclinations are

• 45°

Given θ = 45°

∴  The slope of a line = m = tan θ = tan 45° = 1

• 60°

Given θ = 60°

∴  The slope of a line = m = tan θ = tan 60° = √3

• 30°

Given θ = 30°

∴  The slope of a line = m = tan θ = tan 30° = 1/√3

• 120°

Given θ = 120°

∴  The slope of a line = m = tan θ = tan 120°

∴  m = tan (90° + 30°) = – cot 30° = – √3

• (3π/4)^c

Given θ = (3π/4)^c

∴  The slope of a line = m = tan θ = tan (3π/4)^c

∴  m = tan (π/2 + π/4)^c = – tan (π/4)^c = – 1/√2

• (5π/6)^c

Given θ = (5π/6)^c

∴  The slope of a line = m = tan θ = tan (5π/6)^c

∴  m = tan (π – π/6)^c = – tan (π/6)^c = – 1/√3

• 105°

Given θ = 105°

∴  The slope of a line = m = tan θ

= tan 105° = tan (60° + 45°)

• The line makes an angle of 30° with the positive direction of the y-axis measured anticlockwise.

The angle made by line with the positive direction of the y-axis = – 30° (anticlockwise)

The angle made by line with positive direction of the x-axis = 90° – 30° = 60°

Now θ = 60°

∴  The slope of a line = m = tan θ = tan 60° = √3

Example – 02:

Find the inclinations of lines whose slopes are

• 1

The slope of line = m = tan θ =1

∴  θ = tan^-1(1) = 45° or (π/4)^c

• – 1

The slope of line = m = tan θ = -1

∴  θ = tan^-1(-1) = 135° or (3π/4)^c

• √3

The slope of line = m = tan θ =√3

∴  θ = tan^-1(√3) = 60° or (π/3)^c

•  – √3

The slope of line = m = tan θ = -√3

∴  θ = tan^-1(- √3) = 120° or (2π/3)^c

• 1/√3

The slope of line = m = tan θ =1/√3

∴  θ = tan^-1(1/√3) = 30° or (π/6)^c

•  – 1/√3

The slope of line = m = tan θ = – 1/√3

∴  θ = tan^-1(- 1/√3) = 150° or (5π/6)^c

Science > Mathematics > Coordinate Geometry > Straight Lines > The Inclination of a Line