Angular Displacement, Velocity, and Acceleration

Science > Physics > Circular Motion > Angular Displacement, Velocity, and Acceleration

Angular Displacement:

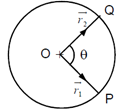
For a particle performing a circular motion the angle, traced by the radius vector at the centre of the circular path in a given time is called the angular displacement of the particle at that time. It is denoted by ‘θ’. Its S.I. unit is radian (rad). It is a dimensionless quantity. [MºLºTº]

The direction of angular displacement: 

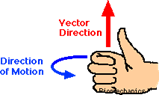
For smaller magnitude (infinitesimal) angular displacement is a vector quantity and its direction is given by the right-hand thumb rule.

Right-Hand Thumb Rule:

If we curl the fingers of our right hand and hold the axis of rotation with fingers pointing in the direction of motion then the outstretched thumb gives the direction of the angular displacement vector.

Sign Convention: 

An angular displacement in counter clock-wise direction is considered positive and that in the clockwise direction is considered as negative.

Vector relation between linear and angular displacement is

Characteristics of Angular Displacement:

• It is the angle, traced by the radius vector at the centre of the circular path in a given time is called the angular displacement of the particle at that time.
• For smaller magnitude (infinitesimal) angular displacement is a vector quantity and its direction is given by the right-hand thumb rule.
• Finite angular displacement is a vector quantity.
• Instantaneous angular velocity is a vector quantity.
• The direction of angular displacement in an anticlockwise sense is considered as positive, while the direction of angular displacement in a clockwise sense is considered as negative.
• The angular displacement of the particle performing uniform circular motion in equal time is equal.
• It is denoted by ‘θ’. Its S.I. unit is radian (rad). It is a dimensionless quantity. [MºLºTº].

Larger angular displacement is not treated as a vector quantity.

If a quantity has both the direction and magnitude then it seems to be vector quantity but it can only be treated as vector quantity if its satisfies laws of vector addition. Consider the following two cases of angular displacement

The commutative law of vector addition which states that if we add two vectors, the order in which we add them does not matter.

We can see that if the order is interchanged the final outcome is different. Thus the angular displacement fails to obey the law of vector addition. Hence larger angular displacement is not a vector quantity.

Angular Velocity:

The rate of change of angular displacement with respect to time is called the angular velocity of the particle. It is denoted by the letter ‘ω’. Its S.I. unit is radians per second (rad s^-1). Its dimensions are [MºLºT ^-1].

Mathematically,

For uniform circular motion, the magnitude of angular velocity is given by

Where ω = Angular speed, T = Period
N = Angular speed in r.p.m., n = Angular speed in r.ps. or Hz.
θ = Angular displacement, t = time taken

The Direction of Angular Velocity: 

For smaller magnitude (infinitesimal) the angular velocity is the vector quantity. Its direction is given by the right-hand thumb rule. It states that “If we curl the fingers of our right hand and hold the axis of rotation with fingers pointing in the direction of motion then the outstretched thumb gives the direction of the angular velocity vector”. Thus, the direction of angular velocity is the same as that of angular displacement.

By this rule, the direction of the angular velocity of the second hand, the minute hand, and the hour hand is perpendicular to the dial and directed inwards.

Angular Speed:

The angle traced by radius vector in unit time is called the angular speed or The magnitude of angular velocity is known an angular speed.

Uniform motion is that motion in which both the magnitude and direction of velocity remain constant. In UCM the magnitude of velocity is constant but its direction changes continuously. Hence UCM is not uniform motion. For uniform circular motion, the angular velocity is constant.

For uniform circular motion, the magnitude of velocity at P = magnitude of velocity at Q = magnitude of velocity at R and the direction of velocity at P ≠ direction of velocity at Q ≠ direction of velocity at R. In uniform circular motion a body moves in a circle describes equal angles in equal interval of time. Thus for a body performing UCM has uniform speed.

For non-uniform circular motion, The magnitude of velocity at P ≠ magnitude of velocity at Q ≠ magnitude of velocity at R and the direction of velocity at P ≠ direction of velocity at Q ≠ direction of velocity at R.  In non-uniform circular motion a body moves in a circle describes unequal angles in equal interval of time.

Characteristics of Angular Velocity:

• The rate of change of angular displacement with respect to time is called the angular velocity of the particle.
• Its direction is given by the right-hand thumb rule.
• The direction of angular velocity is the same as that of angular displacement.
• For uniform circular motion, the magnitude of angular velocity is constant.
• The magnitude of angular velocity (ω) is related to the magnitude of linear velocity (v) by the relation v = rω.
• It is denoted by the letter ‘ω’. Its S.I. unit is radians per second (rad s-1). Its dimensions are [MºLºT ^-1].

Example – 1:

The graph shows angular positions of a rotating disc at different instants. What is the sign of angular displacement and angular acceleration?

The angular velocity at any instant is given by ω = dθ/dt,

At t = 1 second the graph is rising up, thus the slope (dθ/dt) of the tangent at t = 1 second is positive. Hence angular velocity is positive.

At t = 2 seconds the graph reaches the topmost point, thus the slope (dθ/dt) of the tangent at t = 2 seconds is zero. Hence angular velocity is zero.

At t = 3 seconds the graph is going down, thus the slope (dθ/dt) of the tangent at t = 3 seconds is negative. Hence angular velocity is negative.

We can see the change in angular velocity as positive → zero → negative. Thus angular velocity is decreasing. Hence angular acceleration is negative.

Angular Acceleration: 

The average angular acceleration is defined as the time rate of change of angular velocity. It is denoted by the letter ‘α’. Its S.I. unit is radians per second square (rad /s²). Its dimensions are [MºLºT ^-2]. Mathematically,

If the initial angular velocity of the particle changes from initial angular velocity ω₁  to final ω₂ angular velocity in time ‘t’ then

The direction of Angular Acceleration:

The direction of angular acceleration is given by right-hand thumb rule. If the angular velocity is increasing then the angular acceleration has the same direction as that of the angular velocity.

If the angular velocity is decreasing then the angular acceleration has the opposite direction as that of the angular velocity.

For uniform circular motion angular acceleration is zero.

Characteristics of Angular Acceleration:

• The average angular acceleration is defined as the time rate of change of angular velocity.
• If the angular velocity is increasing angular acceleration is positive (e.g. the angular acceleration of the tip of a fan just switched on). If the angular velocity is decreasing angular acceleration is negative (e.g. the angular acceleration of the tip of a fan just switched off)
• If the angular velocity is increasing then the angular acceleration has the same direction as that of the angular velocity. If the angular velocity is decreasing then the angular acceleration has the opposite direction as that of the angular velocity.
• For uniform circular motion angular acceleration is zero.
• The magnitude of angular acceleration (α) is related to the magnitude of linear acceleration (a) by the relation a = rα.
• It is denoted by the letter ‘α’. Its S.I. unit is radians per second square (rad /s²). Its dimensions are [M⁰L⁰T^-2].

Right Handed Screw Rule:

When a right-handed screw is rotated in the sense of revolution of the particle, then the direction of the advance of the screw gives the direction of the angular displacement vector.

Relation Between Linear Velocity and Angular Velocity:

Consider a particle performing uniform circular motion, along the circumference of the circle of radius ‘r’ with constant linear velocity ‘v’ and constant angular speed ‘ω’ moving in the anticlockwise sense as shown in the figure.

Suppose the particle moves from point P to point Q through a distance ‘δx’along the circumference of the circular path and subtends the angle ‘δθ’ at the centre O of the circle in a small interval of time ‘δt’. By geometry

δx = r . δθ

If the time interval is very very small then arc PQ can be considered to be almost a straight line. Therefore the magnitude of linear velocity is given by

Thus the linear velocity of a particle performing uniform circular motion is radius times its angular velocity. In vector form above equation can be written as

The linear velocity can be expressed as the vector product of angular velocity and radius vector.

The following figure shows relative positions of the linear velocity vector, angular velocity vector, and radius or position vector.

For smaller magnitudes angular displacement, angular velocity are vector quantities. Let ( r) be the position vector of the particle at some instant. Let the angular displacement in small time δt be ( δθ). Let the corresponding linear displacement (arc length) be ( δs). By geometry

Dividing both sides of the equation by δt and taking the limit

Period of Revolution:

Let us consider particle performing a uniform circular motion. Let ‘T’ be its period of revolution. During the periodic time (T), particle covers a distance equal to the circumference 2pr of the circle with linear velocity v.

This is an expression for the period of revolution for particle performing the uniform circular motion.

The Expression for Angular Acceleration:

When a body is performing a non-uniform circular motion, its angular velocity changes. Hence the body possesses angular acceleration.
The rate of change of angular velocity w.r.t. time is called as the angular acceleration. We know that acceleration is the rate of change of velocity with respect to time.

r = radius of circular path = constant.

ω = angular velocity of the particle performing a circular motion

Where ‘α’ is angular acceleration. Hence,

linear acceleration = radius x angular acceleration.

If speed is increasing linear acceleration is in the same direction as that of linear velocity. If speed is decreasing linear acceleration is in the opposite direction to that of linear velocity. It is also referred as tangential acceleration. For uniform circular motion α = 0.

Previous Topic: Concept of Uniform Circular Motion

Next Topic: Numerical Problems on Circular Motion

Science > Physics > Circular Motion > Angular Displacement, Velocity, and Acceleration