Science > Physics > Wave Theory of Light >Application of Huygens Wave Theory of Light
In the last article, we had a brief idea of the Huygens wave theory of light. In this article, we shall study its use to explain the phenomena of reflection and refraction of light.
Huygens Principle:
Huygens Proposed that
- Every point on a wavefront behaves as if it is a secondary source of light sending secondary waves in all possible directions.
- The new secondary wavelets are more effective in the forward direction only.
- The envelope (tangent) of all the secondary wavelets at a given instant in the forward direction gives the new wavefront at that instant.
Applications of Huygens Wave Theory of Light:
Huygens Construction of Spherical wavefront:
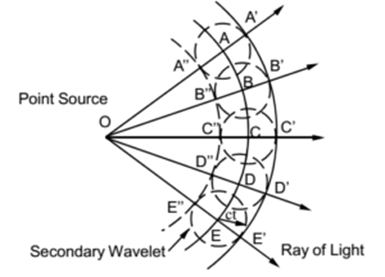
Consider a point source O of light giving rise to a spherical wavefront ABCDE at any instant. According to the Huygens principle, each point on a wavefront acts as a secondary source of light producing secondary wavelets in all directions.
Let ‘c’ be the velocity of light in air and ‘t’ be the time after which position of the wavefront is to be found. During time ‘t’, the light wave will travel a distance of ‘ct’. To find the position and shape of the wavefront after time ‘t’, a number of spheroids of radius ‘ct’ are drawn with their centres A, B, C, D, and E.
The tangential spherical envelope (surface) joining the points A’, B’, C’, D’ and E’ in the forward direction is the new wavefront at that instant. The secondary wavelets are effective only in the direction of wave normal. Therefore, a backward wavefront is absent.
Huygens Construction of Plane Wavefront:
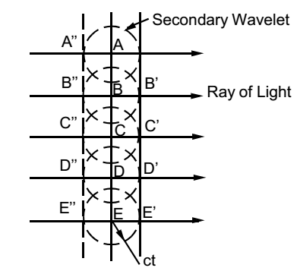
Consider a plane wavefront ABCDE at any instant. According to Huygens’ principle, each point on a wavefront acts as a secondary source of light producing secondary wavelets in all directions.
Let ‘c’ be the velocity of light in air and ‘t’ be the time after which position of the wavefront is to be found. During time ‘t’ the light wave will travel a distance of ‘ct’. To find the position and shape of the wavefront after time ‘t’, a number of spheroids of radius ‘ct’ are drawn with their centres A, B, C, D, and E.
The tangential envelope (surface) joining the points A’, B’, C’, D’ and E’ in the forward direction is the new wavefront at that instant. The secondary wavelets are effective only in the direction of wave normal. Therefore, a backward wavefront is absent.
Reflection of Light on the basis of Huygens Theory:
Laws of Reflection:
- The angle of incidence is equal to the angle of reflection
- The incident ray and the reflected ray lie on either side of the normal at the point of incidence
- The incident ray, reflected ray and the normal at the point of incidence lie in the same plane.
Explanation:

Consider a plane wavefront bounded by parallel rays PA and QB, traveling through air be obliquely incident on a plane reflecting surface XY. At an instant when the plane wavefront AB just touches the reflecting surface, point A’ becomes a secondary source to send out backward secondary wavelets.
As the incident plane, wavefront proceeds further let ‘t’ be the time required by end B of this wavefront to reach the reflecting surface at C. if ‘c’ is the velocity of light in the air then, BC = c t.
During this time, the secondary wavelet, originating from point A spreads over, a hemisphere. In time ‘t’ the radius of this spherical wavelet is AD = c t
Draw a tangent from point C to the secondary spherical wavelet to meet it at D. As C and D are in the same phase, the tangent CD represents the reflected plane wavefront after time ‘t’.
From the ray diagram:
∠ PA’N = ∠ BCN’ = i, angle of incidence
∠ B’CA’ = 90° – i ,
∠ NA’D = r, angle of reflection
∠ DA’C = 90° – r
In Δ A’B’C and Δ CDA’
The angle between plane wavefront and wave normal is 90°
∠ A’B’C = ∠ A’DC = 90°
B’C = A’D = ct
A’C is common to both the triangles
Hence, A’B’C and DCDA’ are congruent. (Hypotenuse side theorem)
∠ B’CA’ = ∠ DA’C (CACT)
∴ 90° – i = 90° – r
∴ i = r
Thus the angle of incidence is equal to angle of reflection,
From the diagram, it can be seen that the incident ray and the reflected ray lie on either side of the normal at the point of incidence. Similarly the incident ray, the reflected ray and the normal at the point of incidence lie in the same plane i.e. plane of the paper. Hence laws of reflection are proved. Thus the phenomenon of reflection is explained on the basis of the Huygens’ wave theory of light.
Reflection of Light on the Basis of Huygens Theory:
Laws of Refraction:
- The ratio of the sine of the angle of incidence to the sine of the angle of refraction is always constant and is equal to the refractive index of the medium. This law is known as Snell’s law.
- The incident ray and the refracted ray lie on the opposite side of the normal at the point of incidence.
- The incident ray, refracted ray and the normal at the point of incidence lie in the same plane.
Explanation:

Consider a plane wavefront bounded by parallel rays PA and QB, traveling through a rarer medium of refractive index μ1 be obliquely incident on a plane refracting surface XY. At an instant when the plane wavefront AB just touches the refracting surface at point A’, point A’ becomes a secondary source to send out secondary wavelets in the second medium of refractive index μ2.
As the incident plane, wavefront proceeds further let ‘t’ be the time required by end B of this wavefront to reach the reflecting surface at B’. If c1 is the velocity of light in the air then, B’C = c1 t.
During this time, the secondary wavelet, originating from point A’ spreads over, a hemisphere in the second medium. Let c2 be the speed of the light in the second medium. In time ‘t’ the radius of this spherical wavelet is A’D = c2 t
Draw a tangent from point C to the secondary spherical wavelet to meet it at D. As C and D are in the same phase, the tangent CD represents the refracted plane wavefront after time ‘t’.
Construct NN’, normal to the refracting surface at A’.
From ray diagram:
∠AA’N + ∠ NA’B’ = 90° ……. (1)
∠NA’B’ + ∠ B’A’C = 90° ……. (2)
From (1) & (2)
∠ AA’N + ∠ NA’B’ = ∠ NA’B’ + ∠ B’A’C
∴ ∠ AA’N = ∠ B’A’C = i
∠N’A’D + ∠ DA’C = 90° ….. (3)
∠DA’C + ∠ A’CD = 90° …. (4)
From (3) & (4)
∠ N’A’D + ∠ DA’C = ∠ DA’C + ∠ A’CD
∴ ∠ N’A’D = ∠ A’CD = r
The angle between plane wavefront and wave normal is 90°
∠ A’B’C = ∠ A’CD = 90°

Thus Snell’s law is proved. From the diagram, it can be seen that the incident ray and the refracted ray lie on the opposite side of the normal at the point of incidence. Similarly the incident ray, the refracted ray and the normal at the point of incidence lie in the same plane i.e. plane of the paper. Hence laws of refraction are proved. Thus the phenomenon of refraction is explained on the basis of the Huygens’ wave theory of light.
For an optically denser medium n > 1, hence the velocity of light in an optically rarer medium is more than optically denser medium.
Next Topic: Numerical Problems on the wave Theory