Introduction to Electromagnetic Induction

Science > Physics > Electromagnetic Induction > Introduction to Electromagnetic Induction

In this article, we shall study the concept of electromagnetic induction i.e. conversion of magnetic energy into electrical energy.

The phenomenon of producing an induced e.m.f. in a conductor or conducting coil due to changing magnetic flux or field is called electromagnetic induction. This phenomenon was discovered independently by Michael Faraday and Joseph Henry.

Magnetic Flux:

The total number of magnetic field line passing through a given area is called the magnetic flux through that area. It is denoted by symbol ‘ø’. Its S.I. unit is weber (Wb)

Magnetic Induction:

Magnetic induction is defined as magnetic flux per unit area. It is denoted by letter ‘B’. Mathematically, B = ø/A. its S.I. unit is weber per square metre Wb/m² or tesla (T).

Faradays Coil Magnet Experiment:

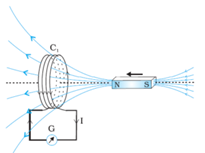
A coil of insulated copper wire is connected to a centre zero galvanometer. A bar magnet is held near the coil. Initially, the galvanometer shows zero deflection. When the magnet is moved towards the coil, a momentary deflection is observed towards one side. When the magnet comes to rest, the deflection also becomes zero. If the speed of motion of the magnet is increased, the deflection also increased.

When the magnet is moved away from the coil, a momentary deflection is observed in the opposite direction. This direction is also observed as long as the magnet is in motion.

Faradays Coil and Coil Experiment:

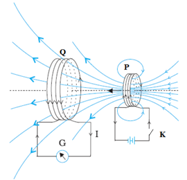
Two coils of insulated copper wire a primary coil P and secondary coil S kept very near to each other. The coil P is connected to a cell and tap key K. The other coil S is connected to a centre zero galvanometer G.

By pressing the tap key, when a current is passed through P. the galvanometer shows a momentary deflection towards one side. When the current in P is switched off, a momentary deflection is observed in the galvanometer in the opposite direction.

Faraday’s Laws of Electromagnetism:

Faraday’s First Law of Electromagnetic Induction:

Whenever there is a change in magnetic flux linked with a coil, an e.m.f is induced in the coil.

Faraday’s Second Law of Electromagnetic Induction:

The magnitude of e.m.f. induced in a coil is directly proportional to the rate of change of magnetic flux linked with it.

Explanation:

If dø is the change in magnetic flux in time dt, then the induced e.m.f.

In S.I. system, e is measured in volts, dø in weber and dt in seconds

This formula gives the magnitude of induced e.m.f.  The direction is given by Lenz’s law.

Lenz’s Law of Electromagnetic Induction:

Lenz’s law states that the direction of e.m.f. induced in a coil is son as to oppose the change in magnetic flux that causes it.

Applying Lenz’s Law, the Faraday’s Law can be expressed as

Negative sign shows that the induced e.m.f. opposes the rate of change of flux.

Explanation:

Lenz’s Law is a direct consequence of the principle of conservation of energy. When N-pole of a magnet approaches a coil, the induced current flows in such a direction that its face attains N-polarity. Hence the magnet is repelled. Therefore, work has to be done in moving the magnet against repulsion, in order to maintain induced current. In other words, there is a conversion of mechanical energy into electrical energy.

Proof of Faraday’s Laws of Electromagnetic Induction:

Consider a rectangular loop of conducting wire PQRS of width ‘l’ be arranged with its plane in the plane of the page.  A uniform magnetic field is applied perpendicular to the loop i.e. perpendicular and towards the page.  The magnetic field arranged in such a way that a part loop is in the field.  If ‘x’ is the width of the loop in the field, then the area of the loop in the field is

A = l . x

Also, the magnetic flux passing through this area due to the field is

ø  = BA

∴  ø  = B . l . x

The loop is now pulled out of the field towards the right with a uniform velocity v.  The flux linked with the loop i.e. B l x undergoes a change.  If dx is the displacement of the loop in time dt, then

But, dx/dt = v = the velocity of the loop

This shows that the flux linked with the loop changes at a constant rate.  Therefore by Faraday’s Law an induced e.m.f. is produced in the loop.  The induced e.m.f. sets up an induced current which flows through the loop in a clockwise direction. A conductor which carries a current in a magnetic field experiences a force given by

The direction of the force is given by Fleming’s left-hand rule. Therefore the side PS of length ‘l’ experiences a force acting towards left is given by

F = I l B sin 90° = F = I l B

This force is unbalanced.

However, the force acting on side PQ and SR are of the same magnitude but opposite in direction. Therefore they balance each other.

When the loop is moved towards the right through distance ‘dx’ in time ‘dt’ in the ‘dt’, the work done against force is given by

dW =  -Fdx

Negative sign shows that displacement is opposite to force.   Substituting for F

dW =  – I l B dx

Therefore, the rate of doing work is given by

This is the mechanical power supplied to the loop the mechanical power generates an equal amount of electrical power by maintaining an induced current. If ‘I’ is the induced current due to an induced e.m.f. ‘e’, then

Electrical power = e i

According to the principle of conservation of energy

Electrical power = Mechanical power

e i  =  -Bi l v

e   = -B l v   …. (2)

Substituting from equation (1) in equation (2), we get

Thus Faraday’s law of electromagnetic induction is proved

Fleming’s Right-Hand Rule:

Stretch the forefinger, middle finger and thumb of right hand mutually perpendicular to each other. If the forefinger points the direction of the magnetic field, and the thumb shows the direction of motion of the conductor then the middle finger shows the direction of induced e.m.f.

Science > Physics > Electromagnetic Induction > Introduction to Electromagnetic Induction