Formation of Beats

Science > Physics > Wave Motion > Formation of Beats

In this article, we shall study the formation of beats, conditions required for its formation, and expression for the period and frequency of beats.

Principle of Superposition of Waves:

When two waves arrive at a point simultaneously, each wave produces its own effect at that point as if that wave alone was passing through the point. Hence, the resultant displacement of the particle at that point is given by the vector sum of the displacements due to individual waves meeting at that point.

Explanation:

If y₁ and y₂ are the displacements produced by two waves arriving at a point simultaneously, then the resultant displacement of the particle is the vector sum of the displacement due to two waves.

Case – I:

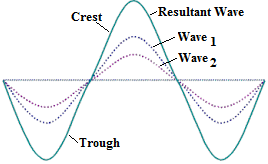
When two waves arrive at a point simultaneously in phase such that the crest of the first wave matches with the crest of the second and trough of the first wave matches with the trough of the second then the resultant displacement of the particle is the vector sum of the displacement produced by the particle by each wave.

In this case, the two waves meet each other in phase and hence the two displacements adds up and the resultant wave has a higher amplitude than any of the two waves. If the amplitudes of the two waves are equal then the resultant amplitude is double the amplitude of each wave.

Case – II:

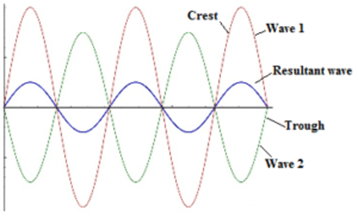
When two waves arrive at a point simultaneously in opposite phase such that the crest of the first wave matches with the trough of the second wave and the trough of the first wave matches with the crest of the second then the resultant displacement of the particle is vector sum of the displacement produced by the particle by each wave.

In this case, the two waves are in opposite phase and hence the two displacement tries to cancel each other and the resultant displacement is the difference between the displacements produced by the two waves. If the amplitudes of the two waves are equal, then the resultant amplitude is zero.

Phenomenon of Beats:

The physical effect of the superposition of two or more waves is called interference.  The phenomenon of beats is an example of interference in time because in this case, we consider the variation in the intensity of sound with time at a given, place.

When two sound waves having equal amplitudes but of slightly different frequencies travel in a medium in the same direction and arrive at a point simultaneously, they interfere and we hear alternate maxima and minima in the resultant intensity of sound. The maximum of sound is called ‘Waxing’ and the minimum of sound is called ‘’Waning’.

The formation of periodic waxing and waning of sound due to interference (superposition) of two sound waves of the same amplitude but of slightly different frequencies, is called beats. One waxing and one waning which are consecutive form one beat.

The time interval between two successive maxima or minima (waxing or waning) is called the period of beats. The number of beats heard per second is called the frequency of beats.

Conditions of Formation of Beats:

• The amplitude of the two interfering waves should be the same.
• The difference between the frequencies of interfering waves should be small. The beats can be heard only if the frequency difference is less than 10.

Terminology of Beats:

• Waxing: When two sound waves having equal amplitudes but of slightly different frequencies travel in a medium in the same direction and arrive at a point simultaneously, they interfere and produce alternate maxima and minima in the resultant intensity of sound. The maximum of sound is called ‘Waxing’
• Waning: When two sound waves having equal amplitudes but of slightly different frequencies travel in a medium in the same direction and arrive at a point simultaneously, they interfere and produce alternate maxima and minima in the resultant intensity of sound. The minimum of sound is called ‘Waning.
• Beats: The formation of periodic waxing and waning of sound due to interference (superposition) of two sound waves of the same amplitude but of slightly different frequencies, is called beats.  One waxing and one waning form one beat.
• Periods of beats: The time interval between two successive maxima or minima (waxing or waning) is called the period of beats.
• Frequency of Beats: The number of beats heard per second is called the frequency of beats.

Quincke’s Experiment to Study Beats Phenomenon:

The phenomenon of interference between two longitudinal waves in the air can be demonstrated by Quincke’s tube shown in the figure. Quincke’s tube consists of U shaped glass tubes A and B. The tube SAR has two openings at S (source)  and R (receiver or listener). The other tube B can slide over the tube A. A sound wave from S (source) travels along both the paths SAR  and  SBR  in the opposite directions and meet at R (receiver end).

If the path difference between the two waves i.e. SAR ~ SBR is an integral multiple of the wavelength, the intensity of the sound will be maximum due to constructive interference. In this case, the path difference between the waves is even multiples of λ/2. The corresponding phase difference between the two waves is even multiples of π. i.e.   2pπ where p = 0, 1, 2, 3 ….

If the tube B is gradually slid over A, a stage is reached when the intensity of sound is zero at R due to destructive interference. Then no sound will be heard at R, In this case, the path difference between the waves is odd multiples of λ/2, the intensity of the sound will be minimum. The corresponding phase difference f between the two waves is odd multiples of π. i.e. (2p + 1)π where p = 0, 1, 2, 3 …..

Expression for Frequency of Beats / Period of Beats:

Consider two sources of sound-emitting sound waves of equal amplitudes ‘a’ and traveling in the same direction at the same speed.  They have slightly different frequencies say n₁ and n₂ where n₁ > n₂. The displacements produced by the two waves is given by

y₁ = a Sin ω₁t   = a Sin 2πn₁t

y₂ = a Sin ω₂t   = a Sin 2πn₂t

By the principle of superposition of waves, the resultant displacement is given by

y = y₁ + y₂

∴   y = a Sin 2πn₁t + a Sin 2πn₂t

∴ y =   a (Sin 2πn₁t + Sin 2πn₂t )

By using the following formula of trigonometry

The form of the equation shows that the resultant motion is also a Simple Harmonic Wave of mean frequency but its amplitude R changes with time. As the intensity is proportional to the square of the amplitude, the resultant intensity will also vary and it will be maximum when R is maximum.

Waxing:

The maximum value of R is ± 2 a and it occurs when,

We find that at instants t₀ , t₁, t₂₁ , t₃ the intensity is maximum or there is waxing of sound. From the above relations, the time interval between two successive maxima (waxing) is given by

Frequency of beats   = Difference between the frequencies of the two waves.

Waning:

The minimum value of R is 0 and it occurs when,

We find that at instants t₀, t₁, t₂₁ , t₃ the intensity is minimum or there is waning of sound. From the above relations, the time interval between two successive minima (waning) is given by

Frequency of beats   = Difference between the frequencies of the two waves.

From the above expressions, we also find that between two successive maxima (waxing), there is a minimum (waning) i.e. Waxing and waning are alternate and equally spaced. Thus the number of beats produced per second is the difference between the frequencies of the two waves.

Applications of Beats:

• Beats are used in tuning musical instruments like sitar, violin, etc. The musical instrument is sounded with another instrument of known frequency.  Generally, the beats are heard.  Then it is slightly adjusted so that there are no beats.  This is called tuning.
• In the Sonometer experiment, beats can be used to adjust the vibrating length between the two bridges.
• To find the frequency (N) of the given tuning fork beats can be used.
• Detection of harmful gases in mines. Two identical organ pipes, one filled with pure air and the other filled with air from the mine are blown together.  If there are no beats then the mine air is pure but if beats are heard the mine-air is impure.

Previous Topic: Reflection of waves

Next Topic: Numerical Problems on Beats

Science > Physics > Wave Motion > Formation of Beats