Science > Physics > Gravitation > Concept of Gravitational Field Intensity
In this article, we shall study the concept of the gravitational field, its characteristics, the concept of inertial and gravitational mass, and numerical problems on the gravitational field.
The Concept of Inertial Mass and Gravitational Mass
Inertial Mass:
The inertial mass of a body is related to its inertia in linear motion. Let a body of mass ‘m’ moves with an acceleration ‘a’ along a straight line under the action of force ‘F’. Then By Newton’s second law of motion,
F = ma, hence a = F/m
Let a = 1 then m = F. Thus the inertial mass of a body is equal to the magnitude of external force required to produce a unit acceleration in the body.
Characteristics of Inertial Mass:
- Inertial mass is a measure of the inertia of a body and is proportional to the quantity of matter contained in the body.
- The inertial mass of a body is independent of size, shape, and state of a body.
- It is not dependent on the temperature of the body.
- It is not affected by the presence or absence of other bodies near it.
- It obeys the simple algebraic law of addition when combined and the simple algebraic law of subtraction when separated or removed.
- In a chemical reaction, the inertial mass of a substance is conserved.
- The inertial mass of a body increases with its speed. The new inertial mass is given by
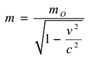
Where ‘mo’ is the rest mass of a body, ‘c’ is the speed of light and ‘v’ is the speed of the body.
- Inertial mass is affected only if the speed of the body is comparable with that of light.
Gravitational Mass:
The gravitational mass of a body is related to the gravitational pull on the body.
If a body of mass ‘m’ is at rest on the surface of the Earth of radius ‘R’ and mass ‘M’, then gravitational force on the body is given by
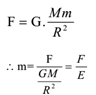
Where ‘E’ is gravitational intensity.
Let E = 1, then m = F. Thus, the gravitational mass of a body is defined as the magnitude of a gravitational pull experienced by the body in a gravitational field of unit intensity.
The characteristics of gravitational mass are the same as that of inertial mass.
Comparison of Inertial Mass and Gravitational Mass:
- Both are scalar quantities and have the same units of measurement.
- The gravitational mass of a body is affected by the presence or absence of other bodies near it. Whereas inertial mass is not affected by the presence or absence of other bodies near it.
- The inertial mass of a body is calculated by finding acceleration in the body and the force causing the acceleration without considering gravity. Whereas gravitational mass of a body is calculated by finding gravitational pull on the body without considering the acceleration in the body and the force causing the acceleration.
- The gravitational mass is measured by spring balance and inertial mass is measured by an inertial balance.
Equivalence of Inertial Mass and Gravitational Mass:
Let us consider two bodies say A and A’ of gravitational masses Mg and Mg’ respectively. Let they are kept in the gravitational field of Erath of gravitational mass ‘M’ at equal distance ‘R’ from the Earth. Then the force on body A is given by

Let Mi and Mi’ respectively be their inertial masses of the two bodies. Now let the two bodies allowed to fall downward in the vacuum from the same height under gravity, where the acceleration due to gravity is ‘g’.

Thus the gravitational mass of a body is directly proportional to its inertial mass. i.e. gravitational mass and inertial mass are equivalent.
This equivalency concept is used by Einstein to develop his Theory of Relativity.
The concept of Gravitational Intensity:
Gravitational Field:
Material particle, when placed in a space, modifies the space around it. This modified space is called the gravitational field. When another particle is brought in this field, it experiences a force of gravitational attraction. The gravitational field is the space around a mass or an assembly (system) of masses over which it can exert gravitational forces on other masses. Theoretically, the gravitational field extends up to infinity, but actually, it becomes very weak to be measured beyond a certain distance.
Gravitational Intensity or Gravitational Field Strength:
Gravitational intensity at a point in a gravitational field is represented in magnitude and direction by the force acting on a unit mass placed at that point.
Thus the magnitude of gravitational intensity I = E = F/m
Where F gravitational force experienced by mass m.
It is a vector quantity, whose direction is the same as that of the gravitational field. It is denoted by E. Its S.I. unit is N kg-1 or m s-2. and c.g.s unit is dyne g-1 or cm -2. Its Dimensions are [M0 L1 T-2]
Expression for Gravitational Intensity:
Let us consider a body of mass ‘m’ at a distance of ‘r’ from the centre of the earth. Let ‘M’ be the mass of the earth. By Newton’s law of gravitation, the force of gravitational attraction is given by


By definition of gravitational mass
E = F/m …………. (2)

Substituting the value of equation (1) in (2)
This is the expression for intensity at a point due to earth’s gravitational field. Where G is universal gravitational constant.
If the test mass ‘m’ is free to move in a gravitational field, then it will move with acceleration towards the mass ‘M’ creating the field. Let the acceleration of the body be ‘a’. Then the force acting on the test mass is given by

Thus the gravitational intensity at a point in the gravitational field is equal to the free acceleration of the test mass placed at that point. If the body creating this gravitational field is earth, then the free acceleration of the test mass is equal to the acceleration due to gravity.

Thus the gravitational field strength at a place is equal to the acceleration produced in a freely falling body at that place.
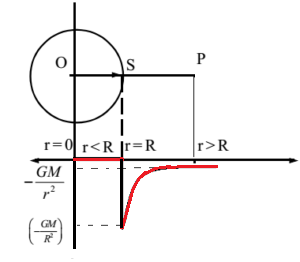
Gravitational Intensity due to the Earth at Different Points:


Inside the earth, the gravitational intensity is zero. On the surface, it is maximum, as the distance of the point from the centre of the earth increases, the gravitational intensity decreases. It is negligible (almost zero) at infinity. This distribution is the same for a solid sphere.
Gravitational Intensity due to the Spherical Shell (Hollow Sphere) at Different Points:


Numerical Problems on Gravitational Intensity
Example – 01:
The Earth-Moon distance is 3.8 x 105 km. The mass of the earth is 81 times that of the moon. Determine the distance from the earth to the point where the gravitational field due to earth and moon cancels out (or resultant gravitational intensity is zero).
Given: Distance between the earth and moon = 3.8 x 105 km, Mass of earth = 1/81 mass of moon i.e. ME = 1/81MM,
To Find: Distance of the point from the earth at gravitational intensity = 0
Solution:
Let the distance between the Erath and Moon be ‘r’. Let x be the distance of the point from the centre of the Earth at which the gravitational field due to earth and moon cancels out. Hence the distance between the point and the centre of the Moon is (r – x)

∴ 9r – 9x = x
∴ 10x = 9r
∴ x = 0.9 r = 0.9 x 3.8 x 105 km = 3.42 x 105 km
Ans: At a distance of 3.42 x 105 km the gravitational field due to earth and moon cancels out.
Example – 02:
Calculate the gravitational intensity due to a point mass 100 kg at a distance of 10 m. Given: G = 6.67 x 10-11 Nm2/kg2.
Given: Mass of body = M = 100 kg, Distance from mass = 1 m, G = 6.67 x 10-11 Nm2/kg2.
To Find: Gravitational intensity; EG =?
Solution:

Ans: The gravitational intensity at the point is 6.67 x 10-9 N/kg.
Example – 03:
Two masses 800 kg and 600 kg are at a distance of 0.25 m apart. Calculate the magnitude of gravitational field intensity at a distance of 0.20 m from 800 kg mass and 0.15 m from the 600 kg mass. Given: G = 6.67 x 10-11 Nm2/kg2.
Solution:

The sides of the triangle are such that they form right angle triangle at the point where gravitational intensity is to be found.
The gravitational intensity due to 800 kg mass at A

It acts along PA
The gravitational intensity due to 600 kg mass at B

It acts along PB
Now the two intensities are at a right angle to each other. Their resultant intensity is given by

Ans: The gravitational intensity at the point is 2.22 x 10-6 N/kg.
Previous Topic: Numerical Problems on Newton’s Law of Gravitation
Next Topic: Concept of Gravitational Potential