Science > Physics > Interference of Light > Interference of Light
In this article, we shall study the phenomenon of interference of light, conditions for constructive and destructive interference.
Terminology:
Constructive Interference of Light:
When two light waves of same frequency arriving at a point, meet each other in the same phase i.e. the crest due to one wave matches with the crest due to other wave and the trough due to first wave matches with the trough due to another wave, then the interference is called constructive interference.
Destructive Interference of Light:
When two light waves of same frequency arriving at a point, meet each other in opposite phase i.e. the crest due to one wave matches with the trough due to other wave and the trough due to first wave matches with the crest due to another wave, then the interference is called destructive interference.
Interference of Light:
The modification in the intensity of light (redistribution of light energy) produced by the superposition of two or more light waves is called interference of light.
Steady Interference Pattern:
An interference pattern in which the intensity of light at any given point remains constant is called a steady or stationary interference pattern.
Monochromatic Sources of Light:
Two sources of light are said to be monochromatic only when they emit light of the same wavelength, i.e. same frequency.
Coherent Sources:
Two sources are said to be coherent if there always exists a constant phase difference between the waves emitted by these sources.
Principle of Superposition of Waves:
Statement: If two or more waves arrive at a point simultaneously, each wave produces its own displacement (effect) at that point as if the other waves are not present.
Hence the resultant displacement of that point at that instant is the vector sum of the displacements due to all the waves.
If two waves arrive at a point simultaneously, and the displacements due to these waves at that point are y1 and y2 respectively, then by the principle of superposition of wave resultant displacement at that point is given by
y = y1 + y2
The Phenomenon of Interference of Light:
The phenomenon of enhancement or cancellation of displacements and the subsequent redistribution of intensity is called interference. The modification in the intensity of light (redistribution of light energy) produced by the superposition of two or more light waves is called interference of light.
When two light waves of same frequency arriving at a point, meet each other in the same phase i.e. the crest due to one wave matches with the crest due to other wave and the trough due to first wave matches with the trough due to another wave, then the constructive interference takes place. At this point intensity is maximum and maximum intensity or brightness is obtained at that point. This point is the bright point.
When two light waves of same frequency arriving at a point, meet each other in opposite phase i.e. the crest due to one wave matches with the trough due to other wave and the trough due to first wave matches with the crest due to another wave, then the destructive interference takes place. At this point intensity is the minimum and minimum intensity or brightness is obtained at that point. This point is the dark point.
If this change in intensity is of light due to interference is obtained on screen, then alternate bright and dark bands or rings are obtained. This pattern is known as the interference pattern.
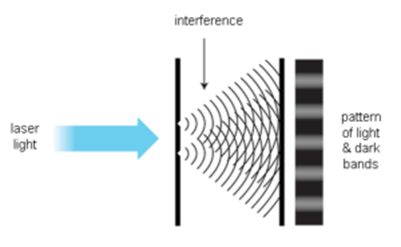
Conditions for Constructive Interference of Light (Formation of Bright Point):
In order to obtain constructive interference or brightness at a point, the two light waves from the two sources should arrive at the point in the same phase.
Thus for brightness, required path difference is 0, λ, 2λ, 3λ, 4λ, 5λ, …. etc. or phase difference is 0, 2π, 4π, 6π, 8π,… etc.
In general for obtaining a bright band at a point, Path difference = nλ where n = 0, 1,2,3…….
Conditions for Destructive Interference of Light (Formation of dark Point):
In order to obtain destructive interference or darkness at the point, the two light waves from the two sources should arrive at the point in the opposite phase.
Thus for darkness, the required path difference is λ/2, 3λ/2, 5λ/2, …. etc. or phase difference is π, 3π, 5π, 7π,… etc.
In general for obtaining a dark band at a point, Path difference = (2n – 1)λ/2 where n = 1,2,3……. etc.
Steady Interference Pattern:
An interference pattern in which the intensity of light at any given point remains constant is called a steady or stationary interference pattern. In such a pattern intensity of light not changes with time i.e. bright point remains bright and a dark point always remains dark. The steady interference pattern consists of alternately bright and dark bands or rings called interference fringes.
Conditions for Obtaining Steady Interference Pattern:
- The two sources of light must be coherent. That is these two sources should emit light waves with a constant phase difference. In such a case, coherent sources are obtained from a single source.
- The amplitudes of waves must be equal. i.e. the brightness of the two sources should be the same.
- The sources of light should be narrow.
- The two sources of light must be monochromatic.
- The distance between the sources and the screen must be reasonably large.
- The distance between coherent sources must be small.
- The sources should emit light waves in nearly the same direction.
- The two interfering waves must be in the same state of polarization.
For obtaining steady interference pattern the two sources of light must be coherent:
Two sources are said to be coherent if there always exists a constant phase difference between the waves emitted by these sources. Two sources are coherent only if they are obtained from one and the same original sources e.g. the two images produced by a biprism of a monochromatic slit can be treated as coherent sources.
It is essential to have coherent sources to produce a stationary interference pattern. Because, if the sources are not coherent then the phase difference will change abruptly at any point and hence the intensity of light will change abruptly. But when the sources are coherent, then the resultant intensity of light at a point will remain constant and so interference fringes will remain stationary.
For obtaining steady interference pattern the light used is monochromatic:
Two sources of light are said to be monochromatic only when they emit light of the same wavelength and the same frequency. If the sources emit light waves of more than one wavelength (frequency) then constructive Interference or brightness at a point due to one may overlap with destructive Interference or darkness due to the other wavelength. Thus interference pattern will be diffused. Therefore, the two coherent sources of light should be monochromatic.
For obtaining steady interference pattern the two light sources should be of equal intensity:
The intensity of light is directly proportional to the square of the amplitude of light waves. In an interference pattern, at some points, complete darkness or zero intensity can be obtained only when the interfering waves arrive at that point in exactly the opposite phase and cancel each other. Therefore, the two sources should emit light waves of same amplitude i.e. same intensity (The sources should be equally bright)
For obtaining steady interference pattern the two sources of light should be narrow:
A wide source of light is equivalent to a number of narrow sources. If the sources are broad, then light waves emitted from different points of the same source itself would interfere. This, therefore causes some pattern to overlap above the actual interference bands making it diffused or less sharp.
For obtaining steady interference pattern the two sources of light should be close to each other:
In an interference pattern, the fringe width or bandwidth (X) is inversely proportional to the distance between the two coherent sources (d). When these sources are close to each other, the fringes will be widely spaced and can be seen clearly. This helps in measuring fringe width accurately.
For obtaining steady interference pattern the distance between the sources and the screen should be large:
In an interference pattern, the fringe width or bandwidth (X) is directly proportional to the distance between the sources and the screen (D). When this distance is large, the fringes will be widely spaced and can be seen clearly. This helps in measuring fringe width accurately.
Next Topic: Numerical Problems on Formation of Bright and Dark Bands