Science > Physics > Stationary Waves > Melde’s Experiment
In this article, we shall study Melde’s experiment of vibrating string and find the relation between the number of loops and the tension in the string.
Melde’s Experiment for Longitudinal or Parallel Position:
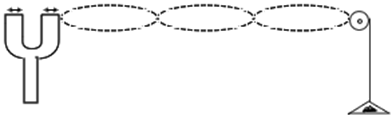
Melde’s experiment set up consists of a light string is tied to one of the prongs of a tuning fork which is mounted on a sounding board. The other end of the string is passed over a horizontal pulley and a light pan is suspended from the free end. The tension in the string can be adjusted by changing the weights placed in the pan, while the vibrating length can be altered by moving the pulley towards or away from the fork.
Experimental Arrangement:
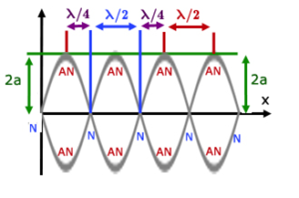
The fork is adjusted so that its arms vibrate parallel to (longitudinal position) the length of the string. The fork is set into vibrations by gently hammering a prong, and the vibrating the length or the tension in the string is adjusted to obtain a number of clear loops on the string. The loops are formed due to interference between the wave starting from the fork and traveling towards the pulley and the wave reflected back from the pulley.
When the prongs vibrate parallel to the length of the string, the frequency of the fork is twice the frequency of the string. Since the string is vibrating in the fundamental mode, its frequency ‘n’ is given by
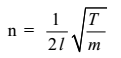
Therefore the frequency of the fork N will be

Hence by measuring the length of the string, the tension T, the mass per unit length m, and by counting the number of loops p, the frequency N of the tuning fork can be found out.
To Prove p²T = Constant:
Frequency of a tuning fork is given by

Melde’s Experiment for Transverse or Perpendicular Position:
In Melde’s experiment setup a light string is tied to one of the prongs of a tuning fork which is mounted on a sounding board. The other end of the string is passed over a horizontal pulley and a light pan is suspended from the free end. The tension in the string can be adjusted by changing the weights placed in the pan, while the vibrating length can be altered by moving the pulley towards or away from the fork.
The fork is adjusted so that its arms vibrate perpendicular to (transverse position) the length of the string. The fork is set into vibrations by gently hammering a prong, and the vibrating the length or the tension in the string is adjusted to obtain a number of clear loops on the string. The loops are formed due to interference between the wave starting from the fork and traveling towards the pulley and the wave reflected back from the pulley.

When the prongs are arranged so that they vibrate at a right angle to the length of the string, the frequency of fork is the same as the frequency of the string. Since the string is vibrating in the fundamental mode, its frequency ‘n’ is given by

Hence by measuring the length of the string, the tension T, the mass per unit length m, and by counting the number of loops p, the frequency N of the tuning fork can be determined.
To Prove p²T = Constant:
Frequency of a tuning fork is given by

To Show that the number of loops is doubled when parallel position is changed to the perpendicular position:
Suppose that the fork is adjusted in the longitudinal position and p1 loops are obtained on the string. Then,

Next, without changing the value of or T, the fork is adjusted in the transverse position and suppose that p2 loops are obtained on the string.

From equation (1) and (2) we get

Thus, the number of loops in the transverse position is twice the number of loops in the longitudinal position for the same values of l and T.
Previous Topic: Sonometer Experiment
Next Topic: Vibrations of Air Column