Couple

Science > Physics > Force > Couple

In this article, we shall study the concept of a couple, its characteristics, and applications.

Parallel Forces:

Forces which are not concurrent and their lines of action are not the same and parallel to each other then the forces are called parallel forces.

Example: Children sitting on a see-saw. Their weights are the example of parallel forces.

If there are two forces which are equal and opposite they form a couple.

Couple:

When two forces of equal magnitude opposite in direction and acting along parallel straight lines, then they are said to form a couple. The perpendicular distance between the two force forming a couple is called the arm of the couple.

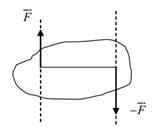
Effects of Couple:

Due to the action of a couple, a torque acts on a body which makes the body to rotate about a fixed point. The torque acting on the body is given by

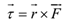
The magnitude of torque = Magnitude of force × perpendicular distance between the two forces.

Characteristics of the Couple:

• As the two forces forming couple are equal and opposite the couple doesn’t produce translational motion.
• When it acts on a body net resultant force acting on a body is zero.
• It produces pure rotational motion in the body this is because the algebraic sum of the moments of the two forces about any point in their plane is not zero.
• The moment of a couple about any point in its plane is constant, both in magnitude and direction.

Practical Application of a Couple:

Couple is required

• to turn a car the driver applies a couple to the steering wheel
• to wind the spring of an alarm clock, it  is applied by the fingers
• to open or close a water tap
• to turn a key of a lock.
• to open or close a cap of a bottle.
• to turn a screwdriver.

Moment of a Couple:

The ability of a couple to produce rotational motion in a body is measured in terms of torque. The magnitude of the torque is equal to the product of one of the forces and the perpendicular distance between the lines of action of the two forces. The moment of a couple about any point in its plane is constant and is equal to the product of one of the forces and the perpendicular distance between the lines of action of the two forces.

Moment of a Couple About Any Point in its Plane is Constant:

Case – I: When Point O is between A and B

Taking moment about O

τ = F . O B × F . O A

τ = F .(OB + OB)

τ= F. (AB)

Case – II: When Point A is between O and B

Taking moment about O.

τ = – F . O A + F . O B

τ = F.(AB)

Case – III: When Point B is between O and A

Taking moment about O.

τ = – F . OB + F . O A

τ = F . (OA – OB)

τ = F. (AB)

In each case, the moment of couple or torque has the same magnitude. It can be proved for any point in the plane of the forces. This proves that the moment of the couple about any point in its plane is constant and is equal to the product of one of the forces and the perpendicular distance between the lines of action of the two forces.

Notes:

A couple or torque produces only the rotational motion of a body.

Let us assume that a couple is applied to a body at rest. The forces constituting the couple are equal in magnitude but opposite in direction. Therefore their resultant is zero.  As there is no resultant force acting on the body, the body does not have translational motion. However, the moment of the couple about any point in its plane is not equal to zero. As a result of this moment, the body is set into purely rotational motion.

Torque and work have the same dimensions but they are different physical quantities.

Work and moment of force (torque) each are the product of force and distance. Hence their dimensions are the same. The dimensions of the moment of force (torque) are [M¹L²T-2] which are the same as work.

When calculating work, the force and the distance are measured in the same direction while the calculating moment of force (torque) the force and the distance are measured in the direction perpendicular to each other.

Moment of force is vector quantity while the work in scalar quantity. Hence though the torque and work have the same dimensions, they are different physical quantities.

The moment of force changes with the change in the axis of rotation while the moment of a couple about any axis is constant.

The magnitude of the moment of a force about the given axis depends on the magnitude of the force and the perpendicular distance of the line of action of a force from the axis of rotation. If the position of the axis of rotation changes the distance of the force from the axis of rotation changes. Hence the moment of force changes.

The magnitude of the moment of couple or torque is equal to the product of one of the forces and the perpendicular distance between the lines of action of the two forces. The calculation is not linked with the axis of rotation. Hence it is independent of the position of the axis of rotation. Hence its magnitude remains constant, even if the axis is changed.

Equilibrium of a Body:

For a body to be in translational equilibrium the resultant force acting on a body should be zero. In such an equilibrium, the body has no translation motion.

∴   ∑ F = 0

For a body to be in rotational equilibrium the resultant moment acting on a body should be zero. In this equilibrium, the body has no rotational motion.

∴   ∑ M = 0

For a body in total equilibrium, the body neither has translational motion nor has rotational motion.

i.e ∑ F = 0 and ∑ M = 0

Previous Topic: Concept of Moment of Force

Next Topic: Centre of Mass

Science > Physics > Force > Couple