Science > Physics > Force > Newton’s Second Law of Motion
In this article, we should study the concept of momentum and Newton’s Second Law of Motion.
To apply Newton’s second law of motion, the body should be acted upon by an unbalanced force. Thus to apply this law we should consider a net force acting on the body.
Statement: The rate of change of momentum of a body is directly proportional to the impressed (applied) force and takes place in the direction of force.
Force is a vector quantity. The direction of the net force is in the same direction as that of the acceleration. Thus, if the direction of the acceleration is known, then the direction of the net force is also known.
Concept of Momentum:
Catching a ball which is moving with a high speed is more difficult than catching a ball which is moving with lesser speed. It is easier to catch a ball of less mass than to catch a ball of more mass. The associated physical quantity is called the momentum of the body. The physical quantity which depends on the velocity of the body and its mass and which determines how much force is required to bring them to rest is known as momentum.
The product of mass and velocity of a body is called the momentum of the body. It is denoted by p. Thus the magnitude of momentum is given by
p = mv.
Since mass is scalar and velocity is a vector, momentum is a vector quantity whose direction is the same as that of velocity.
The S.I. unit of momentum is kilogram metre per second (kg m s-1). The c.g.s. unit is gram centimetre per second (g cm s-1). Dimensions of momentum are [M1L1T-1].
If the body is moving along a straight line path then it is said to possess linear momentum and if it is rotating then it is said to possess angular momentum.
Expression for Force:
Let us assume a body of mass m moving with velocity u. Let a constant force F acts on the body for t seconds and changes its velocity from u to v. then:

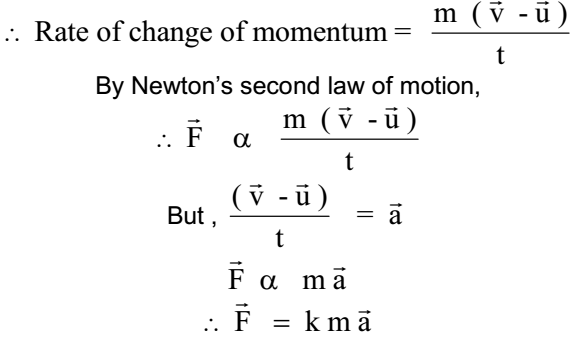
Where k is constant and unit of force is defined such that the value of k is 1. Therefore in scalar form mathematical expression of Newton’s second law of motion is given by
F = ma.
This equation is also called the fundamental equation of classical mechanics.
Note:
- When the mass of a body is constant, then its acceleration is directly proportional to the force acting on it. When the force acting on a body is constant, then its acceleration is inversely proportional to the mass of the body.
- When a rocket is launched upward in space, its acceleration increases. As the fuel in the rocket is burned and exhausted to propel the rocket, the mass of the rocket decreases continuously. But the propulsion force is almost constant. Hence acceleration increases.
Newton’s First Law as Special Case of Newton’s Second Law:
The mathematical expression of Newton’s second law of motion is given by
F = ma.
If we substitute F = 0, in the above equation, the value of ‘a’ the acceleration is found to be zero. Thus there is no change in the magnitude and direction of velocity when there is no force. It means the body at rest is remaining at rest and the body in uniform motion in a straight line is remaining in the uniform motion in the same straight line. Thus Newton’s first law of motion can be considered as a special case of the second law of motion.
Dimensions of force are [M1L1T-2]
Units of Force:
Definition of Unit Force:
Force = Mass x Acceleration .
Hence Unit force = Unit mass x Unit acceleration.
Unit force is that force which produces a unit acceleration in a body of unit mass.
SI unit of force is newton (N) and the cgs unit is dyne. Gravitational units of force in m.k.s. system is kgf and c.g.s. system is gf
Definition of 1 newton (1N):
1 newton = 1 kg x 1 ms-2
One newton is that force which produces an acceleration of 1 ms-2 in a body of mass 1 kg.
Definition of 1 dyne:
1 dyne = 1 gm x 1 cm s-2
One dyne is that force which produces an acceleration of 1 cm s-2 in a body of mass 1 gram.
Definition of 1 kilogram force (1 kgf):
It is a force which is required to produce an acceleration of 9.8 m s-2 in a body of mass 1 kg.
1 kgf = 1 kg x 9.8 m s-2 , Thus 1 kgf = 9.8 N.
Definition of 1 gram force (1 gf):
It is a force which is required to produce an acceleration of 980 cm s-2 in a body of mass 1 g.
1 gf = 1 g X 980 cm s-2 , Thus 1 gf = 980 dyne
The relation between newton and dyne:
1 newton = 1 kg x 1 m s-2 1 , thus newton = 1000 g x 100 cm s-2
Thus 1 newton = 100000 g cm s-2 i.e 1 newton = 105 dyne
Impulse of a Force:
When a large force acts on a body for a short interval of time then the body is said to be subjected to impulse. Impulse is denoted by letter J. e.g. Hammer struck on a nail, the ball struck by a bat.

Thus impulse of force = change in the momentum of the body.
The S.I. unit of impulse is newton-second (Ns). In c.g.s. system its unit is dyne second. Its dimensions are [M1L1T-1] . These dimensions are the same as that of momentum.
Previous Topic: Newton’s First Law of Motion (Concept of Inertia)
Next Topic: Newton’s Third Law of Motion
