Projectile Motion

Science > Physics > Projectile Motion > Analytical Treatment of Projectile Motion

When a body is thrown making an acute angle with the horizontal, the body is said to perform the projectile motion. The path of the body performing projectile motion is called a trajectory. Example: A ball thrown by a fielder to the wicketkeeper, A shell fired from a battle tank.

• Angle of Projection: The angle with the horizontal at which the body is projected is called the angle of projection.
• Velocity of Projection: The velocity with which body is thrown is called the velocity of projection.
• Point of Projection: The point from which the body is projected in the air is called a point of projection.
• Trajectory of Projectile: The path followed by a projectile in the air is called the trajectory of the projectile.
• Range of Projectile: The horizontal distance travelled by the body performing projectile motion is called the range of the projectile.

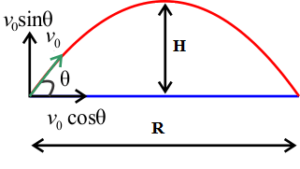
Where V₀ = Velocity of projection, θ = Angle of projection H = Max. height reached

R = Range of projectile t = time required to attain max. height, T = time of flight

Characteristics of Projectile Motion:

• The direction and magnitude of the velocity of the body performing projectile motion change continuously.
• The trajectory of the body performing projectile motion is a parabola.
• During the motion of the body, the vertical component of velocity of projection of body performing projectile motion changes continuously.
• During the motion, the horizontal component of velocity of projection of body performing projectile motion remains constant.
• Projectile motion is a superposition of two motions i.e. motion under gravity and uniform motion along a straight line in the horizontal direction.

The Equation of Path of Projectile:

Let v₀ = Velocity of projection and θ = Angle of projection. Resolving v₀  into two component, viz. v₀ Cosθ the horizontal component and v₀ Sinθ the vertical component. Consider vertical Component v₀ Sinθ. Due to this component, there is the vertical motion of the body.

Let us consider a rectangular Cartesian system of axes such that the origin lies at the point of projection and x-axis is along horizontal and in the plane of projection. Let P(x, y) be the position of the particle after time t from the time of projection.

x coordinate of the position of a particle after time ‘t’ is the horizontal distance travelled by the projectile

x = v₀ Cosθ.  t

y coordinate of the position of a particle after time ‘t’ is the vertical distance travelled by the projectile

y = v₀ Sinθ. t –  g t² ………..(2)

Substituting values of equation (1) in (2) we get

This relation is called the equation of the trajectory of a particle performing projectile motion. In this equation v₀, g and q are constant. This equation is in the form y = a + bx². Where a and b are constant. Thus the trajectory of the projectile is a parabola.

The Velocity of Projectile at Any Instant:

The horizontal component of velocity is always constant. Hence V_H = v₀ Cosθ.

Consider vertical component v₀ Sinθ. Due to this component, there is a vertical motion for the projectile. Thus initial vertical velocity is u = v₀ Sinθ. The vertical velocity of the particle after time ‘t’ is given by

V_V = v₀ Cosθ  – gt
Hence the velocity of a particle performing projectile motion after time ‘t’ is given by

The angle made by the velocity vector with horizontal is given by

Time of Ascent:

The time taken by the body to reach the maximum height is called the time of ascent.

Let v₀ = Velocity of projection and θ = Angle of projection. Resolving v₀  into two components viz. v₀ Cosθ the horizontal component.  And v₀ Sinθ the vertical component. Consider vertical Component v₀ Sinθ. Due to this component, there is the vertical motion of the body.

Initial speed u = v₀ Sinθ

Let the time taken to reach the max. height = time of ascent = t, and

At max. height, final velocity v  = 0

We have, v = u + gt

0 = v₀ Sinθ – gt

∴   gt = v₀ Sinθ

This is an expression for the time required to reach maximum height i.e. time of ascent

Time of Flight:

Time taken by a projectile to cover entire trajectory is called the time of flight.

Initial speed u = v₀ Sinθ

Let the time taken to complete the trajectory = T

as the projectile is reaching the same level of projection vertical displacement y  = 0

We have, s = ut + ½ at²

0 = v₀ Sinθ . T – ½ gT²

∴   0 = v₀ Sinθ  – ½ gT

∴   ½ gT = v₀ Sinθ

Time of Descent:

The time taken by the body to reach from maximum height to the lowest level of the trajectory is called the time of descent.

Time of flight = Time of ascent + Time of descent

∴   Time of descent  = Time of flight – Time of ascent

This is an expression for the time of the descent of a projectile. Also, we can note that time of ascent = time of descent

Maximum Height Reached:

we know that time os ascent, i.e. time taken to reach maximum height is given by

This is an expression for the maximum height reached by the projectile.

Range of Projectile:

The horizontal distance travel by the body performing projectile motion is called the range of the projectile.

The component v₀ cos θ causes the horizontal displacement of the body.

Distance = velocity ´ time

Condition for Maximum Range:

The range of a projectile is given by the formula

In this case, the velocity of projection v₀, the acceleration due to gravity ‘g’ is constant. Hence the range of projectile varies directly with the quantity ‘Sin 2θ’. The range is maximum when the value of the quantity ‘Sin 2θ’ is maximum. The maximum possible value of sine function is ± 1. As the angle of projection is always acute it can take only + 1 value.

Sin 2θ = 1

2θ = Sin ^-1 (1)

2θ = 90°

θ = 45°

Thus for a given velocity of projection, the horizontal range is maximum when the angle of projection is 45°.

Relation Between Maximum Range and Maximum Height Reached by Projectile:

The maximum height reached H by the projectile is given by

The horizontal range R of the projectile is given by

Dividing equation (2) and (1) we have

Thus, for a given velocity of a projection, the maximum range of a projectile is 4 times the maximum height reached by it.

To Show that two complementary angles of projections give the same range of the projectile.

Le θ and (90° – θ) be the two complementary angles of projections. The range in two cases is given by

Thus R₁ = R₂

Hence for two complementary angles of projections give the same range of the projectile.

A body projected horizontally from some height moves along a parabolic path. (Horizontal projection):

Let v_o be the velocity of horizontal projection. Let ’t’ be the time for which the body is in the air. The body has no acceleration in the horizontal direction i.e. the body performs a uniform motion in the horizontal direction. The distance travelled by the body in the horizontal direction is given by

x= v₁.t

As the body is projected horizontally the vertical component of its velocity is zero. Hence the initial velocity in the vertical direction is zero. u = 0

The quantities in the bracket are constant. Hence this equation represents a parabola. Hence the body moves along the parabolic path.

Next Topic: Numerical Problems on Oblique Projection

Science > Physics > Projectile Motion > Analytical Treatment of Projectile Motion