Propagation of Errors

Science > Physics > Units and Measurements > Propagation of Errors

In this article, we shall study the propagation of errors in different mathematical operations.

Propagation of Errors in Addition:

Suppose a result x is obtained by addition of two quantities say a and b

i.e.  x = a + b

Let Δ a and Δ b are absolute errors in the measurement of a and b and Δ x be the corresponding absolute error in x.

∴ x  ± Δ x  = ( a ± Δ a) + ( b ± Δ b)

∴ x  ± Δ x  = ( a + b ) ±  ( Δ a  + Δ b)

∴ x  ± Δ x  = x   ±  ( Δ a  + Δ b)

∴ ± Δ x  = ±  ( Δ a  + Δ b)

∴ Δ x   = Δ a  + Δ b

Thus maximum absolute error in x  = maximum absolute error in a + maximum absolute error in b

Thus, when a result involves the sum of two observed quantities, the absolute error in the result is equal to the sum of the absolute error in the observed quantities.

Propagation of Errors in Subtraction:

Suppose a result x is obtained by subtraction of two quantities say a and b  

i.e.  x = a – b

Let Δ a and Δ b are absolute errors in the measurement of a and b and Δ x be the corresponding absolute error in x.

∴ x  ± Δ x  = ( a ± Δ a) – ( b ± Δ b)

∴ x  ± Δ x  = ( a – b ) ±  Δ a  – + Δ b

∴ x  ± Δ x  = x   ±  ( Δ a  + Δ b)

∴ ± Δ x  = ±  ( Δ a  + Δ b)

∴ Δ x   = Δ a  + Δ b

Thus the maximum absolute error in x  = maximum absolute error in a + maximum absolute error in b.

Thus, when a result involves the difference of two observed quantities, the absolute error in the result is equal to the sum of the absolute error in the observed quantities.

Propagation of Errors in Product:

Suppose a result x is obtained by the product of two quantities say a and b

i.e.  x = a × b    ………..  (1)

Let Δ a and Δ b are absolute errors in the measurement of a and b and Δ x be the corresponding absolute error in x.

∴ x  ± Δ x  = ( a ± Δ a) x ( b ± Δ b)

∴ x  ± Δ x  =  ab  ±  a Δ b ±  b Δ a ± Δ aΔ b

∴ x  ± Δ x  =  x  ±  a Δ b ±  b Δ a ± Δ aΔ b

∴ ± Δ x  =  ±  a Δ b ±  b Δ a ± Δ aΔ b ……  (2)

Dividing equation (2) by (1) we have

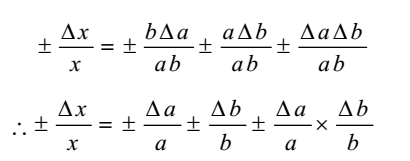
The quantities Δa/a,  Δb/b and  Δx/x are called relative errors in the values of a, b and x respectively. The product of relative errors in a and b i.e. Δa × Δb is very small hence is neglected.

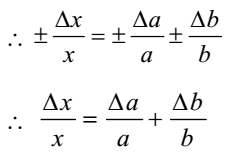
Hence maximum relative  error in x = maximum relative error in a + maximum relative error in b

Thus maximum %  error in x = maximum % error in a + maximum % error in b

Thus, when a result involves the product of two observed quantities, the relative error in the result is equal to the sum of the relative error in the observed quantities.

Propagation of Errors in Quotient:

Suppose a result x is obtained by the quotient of two quantities say a and b.

i.e.   x = a / b    ………..  (1)

Let Δ a and Δ b are absolute errors in the measurement of a and b and Δ x be the corresponding absolute error in x.

The values of higher power of Δ b/b are very small and hence can be neglected.

Now the quantity  (Δ aΔ b / ab)is very small. hence can be neglected.

The quantities Δa/a,  Δb/b and  Δx/x are called relative errors in the values of a, b and x respectively.

Hence maximum relative  error in x  = maximum relative error in a + maximum relative error in b

Thus maximum %  error in x = maximum % error in a + maximum % error in b

Thus, when a result involves the quotient of two observed quantities, the relative error in the result is equal to the sum of the relative error in the observed quantities.

Propagation of Errors in Product of Powers of Observed Quantities:

Let us consider the simple case . Suppose a result x is obtained by following relation

x = aⁿ    ………..  (1)

Let Δ a be an absolute error in the measurement of a and Δ x be the corresponding absolute error in x.

The values of higher power of Δa/a are very small and hence can be neglected.

The quantities  Δa/a and  Δx/x are called relative errors in the values of a and x respectively.

Hence the maximum relative error in x  = n x maximum relative error in a. i.e. maximum relative error in x is n times the relative error in a.

Consider a general relation

The quantities Δa/a,  Δb/b, Δc/c, and  Δx/x are called relative errors in the values of a, b, c and x respectively.

Thus maximum %  error in x is

Examples Explaining Propagation of Error:

Example – 01:

The lengths of the two rods are recorded as  25.2 ± 0.1 cm and 16.8 ± 0.1 cm. Find the sum of the lengths of the two rods with the limit of errors.

Solution:

We know that in addition the errors get added up

The Sum of Lengths = (25.2 ± 0.1) + (16.8 ± 0.1) = (25.2 + 16.8) ± (0.1 + 0.1)  = 42.0 ± 0.2 cm

Example – 02:

The initial temperature of liquid is recorded as 25.4 ± 0.1 °C and on heating its final temperature is recorded as 52.7 ± 0.1 °C. Find the increase in temperature.

Solution:

We know that in subtraction the errors get added up

The increase in temperature = (52.7 ± 0.1) – (25.4 ± 0.1) = (52.7 – 25.4) ± (0.1 + 0.1)  = 27.3 ± 0.2 °C.

Example – 03:

During the study, the flow of a liquid through a narrow tube by experiment following readings were recorded.  The values of p, r, V and l  are 76 cm of Hg, 0.28 cm, 1.2 cm³ s^-1 and 18.2 cm respectively. If these quantities are measured to the accuracies of 0.5 cm of Hg, 0.01 cm, o.1 cm³ s^-1 and 0.1 cm respectively, find the percentage error in the calculation of η if formula used is

Solution:

Example – 04:

 The percentage errors of measurements in a, b, c and d are 1%, 3%, 4% and 2% respectively. These quantities are used to calculate value of P. Find the percentage error in the calculation of P, If the formula used is

Solution:

Previous Topic: Errors and Their Types

For More Topics in Physics Click Here

Science > Physics > Units and Measurements > Propagation of Errors