Science > Physics > Oscillations: Simple Harmonic Motion > The Vibration of Vertical Spring
In this article, we shall study the vibration of vertical spring, when a mass is attached to it.
Oscillation of Mass Due to a Vertical Spring:
Let us consider light and elastic spring of length L suspended vertically from a rigid support. In a relaxed state the spring is unstretched. Let a small mass m be attached to its free end. Due to the action of deforming force mg, the spring extends. Let the extension in the spring be l.
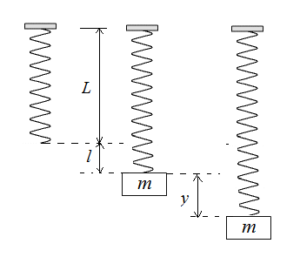
By Hooke’s law, Restoring force ∝ extension
F ∝ – l
F = – k l ……… (1)
Where k = constant called the force constant of the spring.
The negative sign indicates that the direction of restoring force (upward) is opposite to the direction of extension (downward). Let the mass be pulled down by small force ΔF and let the corresponding increase in length be y.
F + ΔF = – k (l + y) ……… (2)
Subtracting equation (1) from (2)
(F + ΔF) – F = – k (l + y) + k l .
ΔF = – k y
This is the restoring force which will create an acceleration in the mass when released.

Thus the acceleration in the mass is directly proportional to the displacement of the mass and opposite to it. But this is the defining character of the linear S.H.M. Thus when the pulled down mass is released it starts performing linear S.H.M. The time period of a particle performing linear S.H.M. in terms of force constant is given by

By Hooke’s Law F = – k l
Considering magnitude only F = k l
This restoring force is equal to the weight of the mass attached
mg = kl

Numerical Problems on Vibration of Vertical Spring:
Example – 01:
A load of 200 g increases the length of a light spring by 10 cm. Find the period of its vertical oscillations when a mass of one kg is attached to the free end of the spring. Take g = 10 m/s2.
Given: Stretching load = F = 200 g = 200 x 10-3 kg= 200 x 10-3 x 10 = 2 N, Increase in length = l = 10 cm = 10 x 10-2 m, mass attached = m = 1 kg, g = 10 m/s2.
To Find: Period = T = ?
Solution:
Force constant k = F/l = 2 / 10 x 10-2 = 20 N/m

Ans: Period of oscillation = 1.41 s
Example – 02:
A vertical light spring is stretched by 2 cm when a weight of 10 g attached to its free end. The weight is further pulled down by 1 cm and released. Compute its frequency and amplitude.
Given: Stretching load = F = 10 g = 10 x 10-3 kg= 10 x 10-3 x 9.8 = 9.8 x 10-2 N, increase in length = l = 2 cm = 2 x 10-2 m, mass attached = m = 10 g = 10 x 10-3 kg, g = 9.8 m/s2, distance through which the mass is pulled down = y = 1 cm = 1 x 10-2 m
To Find: Frequency = ?, amplitude = ?
Solution:
Force constant k = (9.8 x 10-2)/( 2 x 10-2) = 4.9 N/m

The amplitude = distance through which mass is pulled down.
amplitude = a = 1 cm = 0.01 m
Ans: Frequency of oscillation = 3.52 Hz and amplitude = 1 cm
Example – 03:
A mass of 250 g is suspended from a spring of constant 9 N/m. The mass is pulled 10 cm from its equilibrium position and released. Find its speed when it crosses the equilibrium position.
Given: Force constant = 9 N/m, mass attached = m = 250 g = 0.250 kg, distance through which the mass is pulled down = y = 10 cm = 0.1 m
To Find: The speed at equilibrium = v = ?
Solution:

the amplitude = distance through which mass is pulled down.
amplitude = a = 10 cm = 0.1 m
The speed at equilibrium is maximum
vmax = ωa = 6 x 0.1 = 0.6 m/s
Ans: The speed at equilibrium is 0.6 m/s
Example – 04:
One end of steel spiral spring of length 8 cm is fixed to a rigid support. When a mass is suspended from the free end, its length becomes 14 cm. Calculate the periodic time of oscillations of the mass, if displaced vertically.
Given: Original length = 8cm, final length = 14 cm, extension in spring = l = 14 – 8 = 6 cm = 0.06 m,
To Find: Periodic time = T = ?
Solution:
Let m be the mass attached to spring
Force constant k = mg/l = mg / 0.06 = 100mg/6 N/m

Ans: Period of oscillation = 0.4917 s
Example – 05:
A mass M attached to a light spring oscillates with a period of 2 s. If the mass is increased by 2 Kg, the period increases by 1 s. Find M.
Given: m1 = M, T1 = 2 s, m2 = M + 2, T2 = 2 + 1 = 3 s
To Find: M = ?
Solution:
Let k be the force constant of the spring

∴ 9M = 4M + 8
∴ 5M = 8
∴ M = 8/5 = 1.6 kg
Ans: The value of M is 1.6 kg
Example – 06:
Body A and a body B have the same mass. The body A suspended from a light spring of constant k1 and body B is suspended from a light spring of constant k2. The bodies are set into oscillations vertically in such a way that their maximum velocities are equal. Find the ratio of the amplitudes of vibration of the bodies.

Their maximum velocities are equal

Ans: The ratio of amplitude = (k2/k1)1/2.
Example – 07:
A body is supported by a spiral spring and causes a stretch of 1.5 cm is the spring. If the mass is now set into vertical oscillations of small amplitudes what is the periodic time of oscillations? g = 9.8 m/s2.
Given: Elongation in wire = l = 1.5 cm, g = 9.8 m/s2.
To Find: Period of oscillation = T =?
Solution:
Let m be the mass attached to the spring

Ans: Period of oscillation is 0.246 s.
Example – 08:
A spring elongates 2 cm when stretched by a load by 80 g. A body of mass 0.6 kg is attached to the spring and then displaced through 8 cm from its equilibrium position. Calculate the energy of the system in the position. Also, determine the speed of the body when it is 4 cm from the equilibrium position.
Given: Elongation in length of spring = l = 2 cm , mass attached = m = 80 g, g = 9.8 m/s2. Displacement of mass = 8 cm = 0.08 m
To Find: Energy of system = ? Speed at x = 4 cm
Solution:
Force constant k = F/l = 80 x 980 / 2 = 3.92 x 104 dyne/cm

Energy of system = 1/2m ω2a2 = ½ x 80 x (22.14)2(8)2
Energy of system = 1.254 x 106 erg = 0.1254 x 107 erg = 0.1254 J

∴ v = 153.4 cm/s = 1.53 m/s
Ans: Energy of the system = 0.1254 J and speed at the displacement of 4 cm is 1.53 m/s
Previous Topic: Numerical Problems on Simple Pendulum
For More Topics in Physics Click Here
One reply on “The Vibration of Vertical Spring”
Amazing explanation!