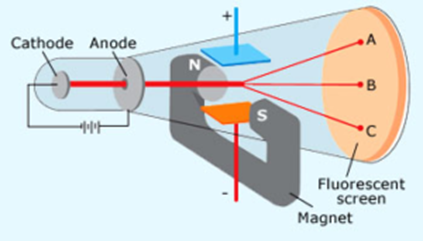
Science > Physics > Photoelectric Effect > Problems on Specific Charge
In this article, we shall see problems based on Thomson’s experiment to calculate the specific charge (e/m).
Example – 01:
In Thomson’s experiment, a beam of electrons travelling at 6.8 x 107 m/s is bent into a circular path of radius 4 cm in a magnetic field of induction 10-2 Wb/m2 normal to its path. Find the specific charge (e/m).
Given: The speed of electron = v = 6.8 x 107 m/s, Radius of circular path = r = 4 cm = 4 x 10-2 m, Magnetic induction = B = 10-2 Wb/m2.
To Find: e/m =?
Solution:
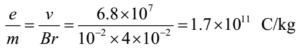
Ans: The value of e/m is 1.7 x 1011 C/kg
Example – 02:
In Thomson’s experiment, a beam of electrons travelling at 10.2 x 107 m/s is bent into a circular path of radius 6 cm in a magnetic field of induction 10-2 Wb/m2 normal to its path. Find the specific charge (e/m).
Given: The speed of electron = v = 10.2 x 107 m/s, Radius of circular path = r = 6 cm = 6 x 10-2 m, Magnetic induction = B = 10-2 Wb/m2.
To Find: e/m =?
Solution:
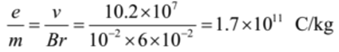
Ans: The specific charge ratio e/m is 1.7 x 1011 C/kg
Example – 03:
In Thomson’s experiment, a beam of electrons travelling at 2.652 x 107 m/s is bent into a circular path in a magnetic field of induction 0.5 Wb/m2 normal to its path. Find the radius of circular path if e/m = 1.76 x 1011 C/kg.
Given: The speed of electron = v = 2.652 x 107 m/s, Magnetic induction = B = 0.5 Wb/m2, e/m = 1.76 x 1011 C/kg.
To Find: Radius of circular path = r =?
Solution:

Ans: The radius of the circular path is 3.01 x 10-4 m
Example – 04:
In Thomson’s experiment, an electron is accelerated from rest in an electric field through a P.D. of 100 V. It then enters normally in the magnetic field of induction 10-3 Wb/m2. Find the radius of curvature of the path.
Given: P.D. = V = 100 V, Magnetic induction = B = 10-3 Wb/m2, e/m = 1.76 x 1011 C/kg.
To Find: Radius of circular path = r =?
Solution:
Let v be the speed of the electron

Ans: The radius of the circular path is 3.37 cm
Example – 05:
In Thomson’s experiment, an electron is accelerated from rest in an electric field through a P.D. of 4000 V. It then enters normally in the magnetic field of induction 10-2 Wb/m2. Find the velocity of electron and the radius of curvature of the path. Mass of electron = 9.1 x 10-31 kg, charge on electron = 1.6 x 10-19 C.
Given: P.D. = V = 4000 V, Magnetic induction = B = 10-2 Wb/m2, mass of electron = m = 9.1 x 10-31 kg, charge on electron = e = 1.6 x 10-19 C.
To Find: Radius of circular path = r =?
Solution:
Let v be the speed of the electron

Ans: Velocity of electron is 3.75 x 107 m/s
The radius of the circular path is 0.0213 m
Example – 06:
In Thomson’s experiment, an electron is accelerated from rest in an electric field through a P.D. of 2000 V. It then enters normally in the magnetic field of induction 3 x 10-4 Wb/m2. Find the velocity of electron and the radius of curvature of the path. Mass of electron = 9.1 x 10-31 kg, charge on electron = 1.6 x 10-19 C.
Given: P.D. = V = 4000 V, Magnetic induction = B = 10-2 Wb/m2, mass of electron = m = 9.1 x 10-31 kg, charge on electron = e = 1.6 x 10-19 C.
To Find: Radius of circular path = r =?
Solution:
Let v be the speed of the electron

Ans: Velocity of electron is 2.625 x 107 m/s
The radius of the circular path is 0.503 m
Example – 07:
In Thomson’s experiment, an electron with kinetic energy 2000 eV enters normally in the magnetic field of induction 0.02 Wb/m2. Find the velocity of electron and the radius of curvature of the path. Mass of electron = 9.1 x 10-31 kg, charge on electron = 1.6 x 10-19 C.
Given: Kinetic energy = 2000 eV = 2000 x 1.6 x 10-19 J, Magnetic induction = B = 10-2 Wb/m2, mass of electron = m = 9.1 x 10-31 kg, charge on electron = e = 1.6 x 10-19 C.
To Find: Radius of circular path = r =?
Solution:
Let v be the speed of the electron

Ans: Velocity of electron is 2.625 x 107 m/s
The radius of the circular path is 7.54 mm
Example – 08:
A beam of electrons travelling at 8.5 x 106 m/s is bent into a circular path in a magnetic field of induction 0.1 Wb/m2 normal to its path. Find the radius of circular path if e/m = 1.7 x 1011 C/kg. Find its angular speed in the magnetic field in the number of revolution.
Given: The speed of electron = v = 8.5 x 106 m/s, Magnetic induction = B = 0.1 Wb/m2, e/m = 1.7 x 1011 C/kg.
To Find: Radius of circular path = r =?, number of revolutions per second = n = ?
Solution:

We have v = r ω
ω = v/r = 8.5 x 106 / 5 x 10-4 = 1.7 x 1010 rad/s
ω = 2πn
n = ω/2π = 1.7 x 1010 / 2 x 3.142 = 2.71 x 109 r.p.s.
Ans: The radius of the circular path is 5 x 10-4 m
The angular speed is 2.71 x 109 r.p.s.
Example – 09:
In Thomson’s experiment, electrons accelerated from rest through a P.D. of 2500 V are collimated into a fine beam to pass through a space between two metal plates where crossed electric and magnetic field is applied. If the magnetic induction is 6 x 10-3 Wb/m2 and the electric intensity is 1.8 x 105 V/m, the electrons beam pass undeviated along a path perpendicular to both electric and magnetic field. Find the e/m ratio.
Given: P.D. applied = V = 2500 V, Magnetic induction = B = 6 x 10-3 Wb/m2, Electric intensity = E = 1.8 x 105 V/m.
To Find: e/m =?
Solution:

Ans: The specific charge ratio e/m is 1.8 x 1011 C/kg
Example – 10:
In Thomson’s experiment, electrons accelerated from rest through a P.D. of 1000 V are collimated into a fine beam to pass through a space between two metal plates where crossed electric and magnetic field is applied. If the magnetic induction is 10-4 Wb/m2 and the electric intensity is 2000 V/m, the electrons beam pass undeviated along a path perpendicular to both electric and magnetic field. Find the e/m ratio and speed of the electron.
Given: P.D. applied = V = 1000 V, Magnetic induction = B = 10-4 Wb/m2, Electric intensity = E = 2000 V/m.
To Find: e/m =?
Solution:

v = E/B = 2000 / 10-4 = 2 x 107 m/s
Ans: The specific charge ratio e/m is 2 x 1011 C/kg
Velocity of electron is 2 x 107 m/s
Example – 11:
In Thomson’s experiment, electrons accelerated from rest through a P.D. of 1000 V enter the space between two metal plates between which a P.D. of 250 V is maintained. The separation between the plates is 5 cm. What magnetic field should be applied perpendicular to the electric field so that the beam remains at right angles to both the fields passes through undeviated. e/m = 1.76 x 1011 C/kg.
Given: P.D. applied = V = 1000 V, P.D. across plate = Vp = 250 V, separation between plates = 5 cm = 5 x 10-2 m, e/m = 1.76 x 1011 C/kg
To Find: Magnetic induction = B =?
Solution:
E = Vp/d = 250/5 x 10-2 = 5 x 103 V/m

Ans: The applied magnetic field is 2.67 x 10-4 Wb/m2
Example – 12:
In Thomson’s experiment, electrons accelerated from rest through a P.D. of 2000 V enter the space between two metal plates between which a P.D. of 800 V is maintained. The separation between the plates is 1 cm. What magnetic field should be applied perpendicular to the electric field so that the beam remains at right angles to both the fields passes through undeviated. e/m = 1.76 x 1011 C/kg.
Given: P.D. applied = V = 2000 V, P.D. across plate = Vp = 800 V, separation between plates = 1 cm = 1 x 10-2 m, e/m = 1.76 x 1011 C/kg
To Find: Magnetic induction = B =?
Solution:
E = Vp/d = 800/1 x 10-2 = 8 x 104 V/m

Ans: The applied magnetic field is 3.02 x 10-3 Wb/m2
Example – 13:
In Thomson’s experiment, a beam of electrons enters the space between two parallel metal plates with a velocity of 2.5 x 107 m/s to encounter a magnetic induction field B = 1.8 x 10-3 Wb/m2 at right angles to its path. What is the radius of curvature of path of the electrons? Find the P.D. to be applied between the plates which are 0.5 cm apart so that the electrons beam passes undeviated. Given e/m = 1.76 x 1011 C/kg
Given: velocity of electron = v = 2.5 x 107 m/s, Magnetic induction = B = 1.8 x 10-3 Wb/m2, separation between plates = d – 0.5 cm = 0.5 x 10-2 m, e/m = 1.76 x 1011 C/kg
To Find: radius of curve path = r =? P.D. across plates = Vp =?
Solution:

Now v = E/B
E = v x B = 2.5 x 107 x 1.8 x 10-3 = 4.5 x 104 V/m
Now, E = V/d
V = E x d = 4.5 x 104 x 0.5 x 10-2 = 225 V
Ans: 225 V
Example – 14:
In Thomson’s experiment to find e/m, the deflector plates were 1.2 cm apart and a P.D. of 820 V was maintained between them. A magnetic field of induction 2.4 x 10-3 Wb/m2 is applied at right angles to the electric field and the direction of motion of the electron beam to produce null deflection. On switching off the electric field, the electron beam traces a circle of radius 7 cm. Find the velocity and e/m of electrons.
Given: P.D. applied = V = 820 V, The separation between plates = d = 1.2 cm = 1.2 x 10-2 m, Magnetic induction = B = 2.4 x 10-3 Wb/m2, Radius of circular path of electron = r = 7 cm = 7 x 10-2 m.
To Find: velocity of electron =? e/m =?
Solution:
E = V/d = 820/1.2 x 10-2 = 6.833 x 104 V/m
Now, the velocity of the electron
v = E/B = 6.833 x 104 / 2.4 x 10-3 = 2.847 x 107 m/s

Ans: The velocity of the electron is 2.847 x 107 m/s
The value of e/m is 1.695 x 1011 C/kg
Example – 15:
In Thomson’s experiment to find e/m, A crossed electric field of strength 8000 N/C and a magnetic field of induction 2 x 10-3 Wb/m2 is applied at right angles to the electric field and the direction of motion of the electron beam to produce null deflection. On switching off the electric field, the electron beam traces a circle of radius 11.4 mm. Find the velocity and e/m of electrons.
Given: Electric intensity = 8000 N/C, Magnetic induction = B = 2.4 x 10-3 Wb/m2, Radius of circular path of electron = r = 11.4 mm = 11.4 x 10-3 m.
To Find: velocity of electron =?, e/m =?
Solution:
velocity of electron v = E/B = 8000 / 2 x 10-3 = 4 x 106 m/s

Ans: The velocity of the electron is 4 x 106 m/s
The value of e/m is 1.754 x 1011 C/kg
Example – 16:
In Thomson’s experiment, a beam of electrons passes undeviated through cross electric and magnetic field. If E = 1.5 x 103 V/m and B = 0.4 Wb/m2, find velocity of electron if its direction is perpendicular to both E and B.
Given: E = 1.5 x 103 V/m, B = 0.4 Wb/m2.
To Find: Velocity of electron = v =?
Solution:
V = E/B = 1.5 x 103 / 0.4= 3.75 x 103 m/s
Ans: The velocity of electron is 3.75 x 103 m/s
Previous Topic: Thomson’s Experiment to Find Specific Charge (e/m Ratio)
Next Topic: Photoelectric Effect