Numerical Problems on Motion Under Gravity Set 01

Science > Physics > Motion in a Straight Line > Numerical Problems on Motion Under Gravity Set – 01

In this article, we shall study to solve problems to calculate time of ascent, time of descent, time of flight, maximum height reached, velocities at various points on path.

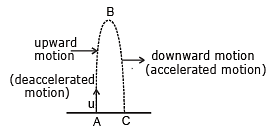
Example – 01:

Show that for a body thrown vertically upwards, the time of ascent is equal to time of descent.

Le u be the initial velocity by which the body is thrown vertically upward.

v = u + g t

∴ 0 = u – g t

∴ g t = u

∴ t = u/g = Time of ascent …….. (1)

Body is returning back to same position hence h = 0 and t = T = Time of flight

h = u t + ½ gt²

∴ 0 = u T – ½ gT²

∴ 0 = u – ½ gT

∴ ½ gT = u

∴ T = 2u/g = Time of flight ……….. (2)

Now Time of flight = Time of ascent + Time of descent

∴ Time of Descent = Time of flight – Time of ascent

∴ Time of Descent = 2u/g – u/g …………. (3)

∴ From equations (1) and (3)

Time of ascent = Time of descent   (Proved)

Example 02:

A body is thrown vertically upwards with a velocity of 78.4 m s^-1. Find how high it will rise and how much time it will take to return to its point of projection. g = 9.8 ms^-2.

Given: Velocity of projection = u = +78.4 m s^-1, acceleration due to gravity = g = – 9.8 m s^-2.

To Find: Height reached = h =?, Time of flight = T =?

Solution:

Calculation of maximum height reached:

At the highest point of its journey, final velocity v = 0

v² = u² + 2gh

∴ 0² = 78.4² + 2(-9.8)h

∴ 0² = 78.4² + 2(-9.8)h

∴ 19.6 h = 78.4 x 78.4

∴ h = 4 x 78.4 = 313.6 m

Calculation of time of flight:

Now, v = u + gt

∴ 0 = 78.4 – 9.8 t

∴ 9.8 t = 78.4

∴ t = 78.4/9.8 = 8 s

Time of flight = Time of ascent + Time of descent

∴ Time of flight = 8 + 8 = 16 s

Ans: Height reached by the body is 313.6 m and time taken to come down is 16 s.

Example – 03:

A body is thrown vertically upwards with a velocity of 14 m s^-1. Find how high it will rise and time of descent.

Given: Velocity of projection = u = +78.4 m s^-1, acceleration due to gravity = g = – 9.8 m s^-2.

To Find: Height reached = h =?, Time of flight = T =?

Solution:

Calculation of maximum height reached:

At the highest point of its journey, final velocity v = 0

v² = u² + 2gh

∴ 0² = 14² + 2(-9.8)h

∴ 0² = 14² + 2(-9.8)h

∴ 19.6 h = 14 x 14

∴ h = 196/19.6 = 10 m

Calculation of time of descent:

Now, v = u + gt

∴ 0 = 14 – 9.8 t

∴ 9.8 t = 14

∴ t = 14/9.8 = 1.428 s

∴ Time of ascent = 1.428 s

In such case, Time of ascent = Time of descent

∴ Time of descent = 1.428 s

Ans: Height reached by the body is 10 m and time of descent = 1.428 s.

Example – 04:

A body thrown vertically upwards is back in the hands of the thrower after 6 s. How high did it go and where was it 4 s after start? g = 9.8 m s^-2.

Given: Velocity of projection = u = +78.4 m s^-1, acceleration due to gravity = g = – 9.8 m s^-2.

To Find: Height reached = h =?, Position of the body after 4 s =?

Solution:

Calculation of maximum height reached:

Body is returning back to same position hence

h = 0 and t= T = Time of flight

h = ut + ½ gt²

∴ 0 = uT – ½ gT²

∴ 0 = u – ½ gT

∴ u = ½ gT = ½ x 9.8 x 6 = 29.4 m/s

Now, Time of ascent = Time of flight/2 = 6/2 = 3 s

h = ut + ½ gt²

∴ h = 29.4 x 3 – ½ x 9.8 x 3²

∴ h = 88.2 – 44.1 = 44.1 m

To find position of the body after 4 s

h = ut + ½ gt²

∴ h = 29.4 x 4 – ½ x 9.8 x 4²

∴ h = 117.6 – 78.4 = 39.2 m

Ans: Height reached = 44.1 m and position of the body is 39.2 m above the ground after 4 s.

Example 05:

A body is projected vertically upwards with a velocity of 21 m s^-1. Find

a) the maximum height reached by the body

b) time taken by it to reach its height

c)  the velocity with which it passes a point A at a height of 10m.

d)  the time that elapses when the body passes through A on its downward journey from the instant of projection. g = 9.8 m s^-2.

Given: Velocity of projection = u = + 21 m/s, acceleration due to gravity = g = – 9.8 m s^-2.

To Find: Maximum height reached = h =? Time required to reach maximum height = t =? Velocity =? at height h = 10 m, Time t =? at height h = 10 m.

Solution:

To find maximum height reached:

At the highest point of its journey, final velocity v = 0

v² = u² + 2gh

∴ 0² = 21² + 2(-9.8)h

∴ 0² = 441 + 2(-9.8)h

∴ 19.6 h = 441

∴ h = 441/19.6 = 22.5 m

To find time taken to reach maximum height:

Now, v = u + gt

∴ 0 = 21 + (-9.8) t

∴ t = 21/9.8 = 2.14 s

To find velocity at point A at height of 10 m:

Now, v² = u² + 2gh

∴ v² = 21² + 2(-9.8)(10)

∴ v² = 441 – 196

∴ v² = 245

∴ v = 15.65 ms^-1

To find the time that elapses when the body passes through A:

v = u + gt

v = -15.65 ms^-1 for downward journey

∴ -15.65 = 21 + (-9.8)t

∴ 9.8 t = 36.65

∴ t = 36.65/9.8 = 3.74 s

Example 06:

A ball is thrown vertically upward from the ground level with a velocity of 19.6 m/s. How long does it take to reach the maximum height? What maximum height does it reach? What is the velocity of the ball at t = 3s.

Given: Velocity of projection = u = + 19.6 m/s, acceleration due to gravity = g = – 9.8 m s^-2.

To Find: Maximum height reached = h =? Time required to reach maximum height = t =? Velocity =? At t = 3 s.

Solution:

To find maximum height reached:

At the highest point of its journey, final velocity v = 0

v² = u² + 2gh

∴ 0² = 19.6² + 2(-9.8)h

∴ 0² = 19.6 x 19.6 + 2(-9.8)h

∴ 19.6 h = 19.6 x 19.6

∴ h = 19.6 m

To find time required to reach maximum height:

Now, v = u + gt

∴ 0 = 19.6 + (-9.8) t

∴ t = 19.6/9.8 = 2 s

To find velocity at time t = 3 s:

Now, v = u + gt

∴ v = 19.6 + (-9.8) x 3

∴ v = 19.6 – 29.4

∴ v = – 9.8 ms^-1

negative sign indicates downward direction.

Ans: Maximum height reached = 19.6 m, time taken to reach maximum height = 2 s, Velocity at t = 3s is 9.8 ms^-1 downward.

Example 07:

Find the velocity at which a rifle bullet must be fired vertically so as to reach a height of 1 km. What time will elapse before the bullet passes through the firing point on its return journey? g = 9.8 m s^-2.

Given: Maximum height reached = 1 km = 1000 m, Acceleration due to gravity = g = 9.8 m s^-2

To Find: Velocity of projection = u = ?, Time of flight = T =?

Solution:

To find velocity of projection:

At the highest point of its journey, final velocity v = 0

v² = u² + 2gh

∴ 0² = u² + 2(-9.8)(1000)

∴ 0² = u² – 4900

∴ u² = 4900

∴ u = 70 m s^-1

To find time of flight:

v = u + gt

∴ 0 = 70 + (-9.8) t

∴ t = 70/9.8 = 7.14 s

∴ Time of ascent = 7.14 s

Time of flight = 2 x Time of ascent

∴ Time of flight = 2 x 7.14 = 14.28 s

Ans: Velocity of projection is 70 ms-1 and time required to reach back at point of fire is 14.28 s

For More Topics in Motion in Straight Line Click Here

For More Topics in Physics Click Here