Numerical Problems on Projectile Motion – 01

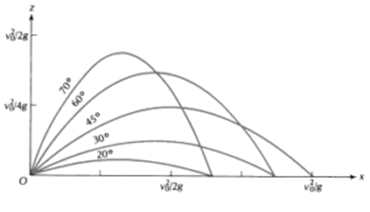
Science > Physics > Projectile Motion > Numerical Problems on Projectile Motion – 01

Example – 01:

A body is projected with a velocity of 49 m/s at an angle of 30^o with the horizontal. Find a) the maximum height reached by the body, b) the time of ascent, c) the time of flight, d) horizontal range and e) The direction of its velocity after 1 s.

Given: velocity of projection = v_o = 49 m/s, Angle of projection = θ = 30^o, g = 9.8 m/s².

To Find: Maximum height reached = H =?, Time of ascent = t_a =?, time of flight = T =?, horizontal range = R =?, the direction of velocity = α =? At t = 1 s.

Solution:

The maximum height reached is given by

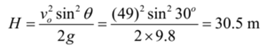
The time of ascent is given by

The time of flight is given by

The horizontal range is given by

The direction of velocity after time t is given by

Ans: Maximum height reached = 30.6 m, time of ascent = 2.5 s, time of flight = 5s, Horizontal range = 212.2 m, Direction of velocity after 1 s is 19^o6’ with horizontal.

Example – 02:

A body is projected with the velocity of 60 m/s at an angle of 30^o with the horizontal. Find a) the maximum height reached by the body, b) the time of flight, c) horizontal range

Given: velocity of projection = v_o = 60 m/s, Angle of projection = θ = 30^o, g = 9.8 m/s².

To Find: Maximum height reached = H =?, time of flight = T =?, horizontal range = R =?,

Solution:

The maximum height reached is given by

The time of flight is given by

The horizontal range is given by

Ans: Maximum height reached = 45.92 m, time of flight = 6.12 s, horizontal range = 318.1 m

Example – 03:

A cricket ball is thrown at a speed of 28 m/s in a direction 30° above the horizontal. Calculate (a) the maximum height, (b) the time taken by the ball to return to the same level, and (c) the distance from the thrower to the point where the ball returns to the same level

Given: velocity of projection = v_o = 28 m/s, Angle of projection = θ = 30^o, g = 9.8 m/s².

To Find: Maximum height reached = H =?, time of flight = T =?, horizontal range = R =?,

Solution:

The maximum height reached is given by

The time of flight is given by

The horizontal range is given by

Ans: Maximum height reached = 10.0 m, time of flight = 2.86 s, horizontal range = 69.28 m

Example – 04:

A body is projected with a velocity of 98 m/s at an angle of 60^o with the horizontal. Find a) the maximum height reached by the body, b) horizontal range

Given: velocity of projection = v_o = 98 m/s, Angle of projection = θ = 60^o, g = 9.8 m/s².

To Find: Maximum height reached = H =?, horizontal range = R =?,

Solution:

The maximum height reached is given by

The horizontal range is given by

Ans: Maximum height reached = 367.5 m, horizontal range = 848.7 m

Example – 05:

A shell is projected with a velocity of 196 m/s at an angle of 30^o with the horizontal. Find a) the maximum height reached by the shell, b) the time of flight, c) horizontal range

Given: velocity of projection = v_o = 196 m/s, Angle of projection = θ = 30^o, g = 9.8 m/s².

To Find: Maximum height reached = H =?, time of flight = T =?, horizontal range = R =?,

Solution:

The maximum height reached is given by

The time of flight is given by

The horizontal range is given by

Ans: Maximum height reached = 490 m, time of flight = 20 s, horizontal range = 3395 m

Example – 06:

A body is projected with a velocity of 40 m/s at an angle of 30^o with the horizontal. Find a) the maximum height reached by the shell, b) the time taken to reach maximum height, c) horizontal range

Given: velocity of projection = v_o = 40 m/s, Angle of projection = θ = 30^o, g = 9.8 m/s².

To Find: Maximum height reached = H =?, time of ascent  = t_a =?, horizontal range = R =?,

Solution:

The maximum height reached is given by

The time to reach maximum height is given by

The horizontal range is given by

Ans: Maximum height reached = 20.41 m, time required to reach maximum height = 2.041 s, horizontal range = 141.4 m

Example – 07:

A bullet is fired from a rifle, attains a maximum height of 25 m and a horizontal range of 200 m. Find the angle of projection.

Given: maximum height reached = H = 25 m, horizontal range = R = 200m, g = 9.8 m/s².

To Find: Angle of projection = θ =?

Solution:

The maximum height reached is given by

The horizontal range is given by

Dividing equation (1) by (2)

Ans: The angle of projection is 26^o34’

Example – 08:

A projectile attains a maximum height of 100 m and a horizontal range of 400 m. Find the angle of projection and velocity of projection.

Given: maximum height reached = H = 100 m, horizontal range = R = 400m, g = 9.8 m/s².

To Find: Angle of projection = θ =?, velocity of projection = v_o =?

Solution:

The maximum height reached is given by

The horizontal range is given by

Dividing equation (1) by (2)

The range of a projectile is given by

Ans: The angle of projection is 45^o and the velocity of projection is 62.61 m/s

Example – 09:

The horizontal range of a projectile is equal to the maximum height attained by the body. What is the angle of projection?

Given: maximum height reached (H) = horizontal range (R), g = 9.8 m/s².

To Find: Angle of projection = θ =?

Solution:

The maximum height reached is given by

The horizontal range is given by

Given R = H

Ans: The angle of projection is 75^o48’

Example – 10:

The maximum horizontal range of a projectile is 980 m. Find the velocity of its projection.

Given: maximum horizontal range = R = 980 m, g = 9.8 m/s².

To Find: velocity of projection  = v_o =?

Solution:

For maximum range θ = 45^o

The horizontal range is given by

Ans: Velocity of projection is 98 m/s

Previous Topic: The Concept and Terminology of Projectile Motion

For More Topic in Physics Click Here

Science > Physics > Projectile Motion > Numerical Problems on Projectile Motion – 01