Science > Physics > Stationary Waves > Vibration of String
In this article, we shall study different modes of vibrations of string, the expression for its fundamental frequency of vibration.
Concept of Overtones:
- Whenever a string or an air column is set into vibrations, the vibrations consist of the fundamental frequency accompanied by certain higher frequencies. These higher frequencies are called overtones.
- They need not necessarily be all the integral multiples of the fundamental frequency.
- All overtones are present. Overtones are called first, second, etc. In case of vibrations of a string, the first overtone is the second harmonic second overtone is the third harmonic and so on.
Concept of Harmonics:
- Harmonics are simply integral multiples of the fundamental frequency. Thus if the fundamental frequency is n, the harmonics are 2n, 3n, 4n, etc.
- They are always the integral multiples of the fundamental frequency.
- They may be or may not be present in the given sound.
- There is nothing like first harmonic. If the fundamental frequency is n, 2n, is called second harmonic, 3n is called third harmonic, etc.
- In case of vibrations of string, the first overtone is the second harmonic second overtone is the third harmonic and so on.
- In case of air column vibrating in a pipe closed at one end only odd harmonics are present. In the case of an air column vibrating in a pipe open at both ends, all harmonics are present.
Vibrations of String:
If ‘T’ is the tension in the string and ‘m’ is its linear density, then the velocity of the transverse wave along its length of a stretched wire is given by the expression
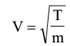
Now let us consider a string of finite length and fixed at its two ends. The wave disturbance, originating at the point at which the string is plucked, travels up to the ends and is reflected back. The incident and reflected waves interfere to produce a stationary wave. The particles of the string, at the two ends, which are fixed, always remain at rest. Hence, the two ends of the string always become nodes. However, the other particles in the string can vibrate in different ways giving rise to what is called the different modes of vibration of the string.
Vibrations of String (Fundamental Mode):
In the following figure, the string is shown to be vibrating in the simplest mode called the fundamental mode. In this mode, the frequency of vibration is the least. The two ends are nodes and there is an antinode exactly midway between the two ends. In other words, one complete loop is formed on the string. Since the distance between the two consecutive nodes is λ/2, the length of the string (l).
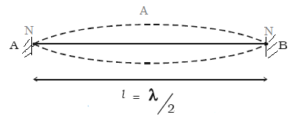

This is the frequency of the fundamental mode of vibration of the stretched string.
Vibrations of String (First Overtone):
In the following figure, the string is shown to have broken up into two complete loops, there is a node midway between the two nodes and an antinode at a distance equal to a quarter of the length of the string from each end. This mode of vibration is called the first overtone. If the wavelength corresponding to this mode is λ1 and frequency is n1.


This is the frequency of the first overtone of vibration of the stretched string. Thus the first overtone is the second harmonic.
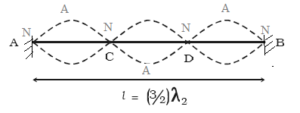
Vibrations of String (Second Overtone):
In the following figure, the string is shown to have broken up into three complete loops. This is the second overtone. If the wavelength corresponding to this mode is λ2and frequency is n2. Then.


This is the frequency of the second overtone of vibration of the stretched string. Thus the second overtone is the third harmonic.
Thus we can conclude that, in the case of vibrating string
- The stretched string vibrates with all harmonics.
- The pth overtone is (p + 1)th harmonic.
- Frequency of pth overtone = (p +1)n
- Frequency of pth harmonic = pn
- Mode of vibration of string gives frequency n, 2n, 3n, …… so on.
Frequency of the Fundamental Mode in Terms of its Radius of Cross-section and Density of the Material of the String:
The frequency (n) of the fundamental mode of transverse vibration of a stretched string is given by

This is an expression for the fundamental mode of transverse vibration of a string in terms of its radius of cross-section and density of the material.
- For a given material of the wire, having a constant length and same tension, the frequency of transverse vibration is inversely proportional to its radius.
- For a wire having the same radius, same length and same tension, the frequency of transverse vibration is inversely proportional to the square root of the density of its material.
Frequency of the Fundamental Mode in Terms of Young’s Modulus of Elasticity of the Material of the String:
The frequency (n) of the fundamental mode of transverse vibration of a stretched string is given by


Substituting the value of equation (2) and (3) in (1)

This is an expression for the fundamental mode of transverse vibration of a string in terms of Young’s modulus of elasticity of the material.
Frequency of the Fundamental Mode in Terms of Coefficient of Thermal Expansion of the Material of the Wire:
The frequency (n) of the fundamental mode of transverse vibration of a stretched string is given by

m = area of the cross-section of wire × density
∴ m = π r² ρ ………… (2)
Now, T = F = tension in the wire
Substituting these values in equation (1)

This is an expression for the fundamental mode of transverse vibration of a string in terms of the coefficient of thermal expansion of the material.
Effect of dipping Stretching Load in a Liquid on Frequency of Vibrating Wire:
The specific gravity of a liquid is defined as the ratio of the weight of the body in the air to the loss of the weight of the body when immersed in the water. Thus, the specific gravity of a liquid is given by

This relation gives the new frequency of vibration of wire on immersing the load in a liquid of specific gravity σ.
Previous Topic: Formation of Stationary waves
Next Topic: Laws of Vibrating String and Sonometer Experiment