Escape Velocity of a Body

Science > Physics > Gravitation > Escape Velocity of a Body

If the velocity of upward projection is increased, a stage will be reached when the velocity given to the body is such that, the kinetic energy of the body is sufficient to overcome the gravitational influence of the earth. This velocity is known as escape velocity.

The escape velocity of a body which is at rest on the earth’s surface Is defined as that minimum velocity with which It should be projected from the surface of the earth so that it escapes from the earth’s gravitational influence.

Escape the velocity of a body does not depend on the direction in which the body is thrown from the surface of the planet.

Expression for Escape Velocity of a Satellite:

Consider a body of mass ‘m’ which is at rest on the surface of the earth.  Let M be the mass of the earth and R be the radius of the earth.  Then the binding energy of the body on the surface of the earth is given by

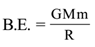
Where G is Universal gravitational constant.

Let V_e be the escape velocity, then the kinetic energy given to the body is given by

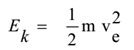
It means Satellite should be given this much kinetic energy so that it can go out of earth’s gravitational influence.

K.E. = B.E.

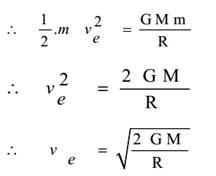
This is an expression for the escape velocity of a satellite on the surface of the earth.

This equation shows that the escape velocity of a satellite is independent of the mass of the satellite (as term ‘m’ is absent).  The escape velocity is the same for all bodies from the given planet.  Escape velocity depends on the mass of the planet and its radius. For earth escape velocity is 11.2 km/s.

Expression in terms of Acceleration Due to Gravity:

We know that   GM = R²g

This is the expression for the escape velocity of a satellite in terms of acceleration due to gravity

Factors Affecting Escape Velocity:

• The escape velocity of a body is directly proportional to the square root of mass (M) of the planet (earth) around which the satellite is orbiting.
• The escape velocity of a body is inversely proportional to the square root of the radius of the Planet
• The equation does not contain the term, ‘m’ which shows that the critical velocity is independent of the mass of the satellite.
• The escape velocity of a body is independent of the direction of projection.

Relation Between Escape Velocity and Critical Velocity of a Satellite Moving Very Close to the Earth’s Surface:

For an orbiting satellite, critical velocity is given by

Where G = Universal gravitational constant

M = the mass of the earth

R = the radius of the earth

h = height of the satellite above the earth’s surface.

For a satellite orbiting very close to the earth, h can be neglected as h < < R. Hence it can be neglected. Therefore the critical velocity of the satellite orbiting very close to the earth’s surface.

The escape velocity of a satellite oh the surface of the earth is given by

Dividing equation (1) by (2)

 Thus the escape velocity of a body from the surface of the planet (earth) is √2  times the critical velocity of the body when it is orbiting close to the planet’s (earth’s) surface.

Expression in Terms of Density of Material of a Planet (earth):

The escape velocity of a satellite on the surface of the planet is given by

Where G = Universal gravitational constant

M = the mass of the Planet

R = the radius of the Planet

Let d    = density of the material of the planet

Now, Mass of Planet   = Volume of Planet x Density

This is an expression for escape velocity in terms of the density of the material of the planet.

Numerical Problems:

Example – 01:

The radius of earth is 6400 km, calculate the velocity with which a body should be projected so as to escape earth’s gravitational influence. Does the escape velocity depend upon the direction in which the body is projected? g =9.8 m/s².

Given: radius of earth = R = 6400 km = 6.4 x 10⁶ m, Acceleration due to gravity = 9.8 m/s².

To Find: v_e =?

Solution:

Ans: Thus the body should be thrown with a speed of 11.2 km/s. We are supplying kinetic energy to the body by throwing it. Hence it is independent of the direction of the throw.

Example – 02:

Calculate the escape velocity on the surface of the earth if the radius of the earth = 6400 km, G = 6.67 x 10^-11 Nm² /kg², and Density of the earth is 5500 kg /m³.

Given: Radius of earth = R = 6400 km = 6.4 x 10⁶ m, G = 6.67 x 10^-11 Nm² /kg². d = 5500 kg /m3, density = d = 5500 kg /m³.

To Find:  v_e =?

Solution:

Ans: Escape velocity is 11.2 km/s

Example – 03:

Calculate the escape velocity on the surface of the planet having radius 1100 km and acceleration due to gravity on the surface of the planet 1.6 m/s².

Given: radius of planet = R = 1100 km = 1.1 x 10⁶ m, Acceleration due to gravity = 1.6 m/s².

To Find: v_e =?

Solution:

Ans: Escape velocity on the planet is 1.876 km/s

Example – 04:

Calculate the escape velocity on the surface of the planet having radius 2000 km and acceleration due to gravity on the surface of the planet 2.5 m/s².

Given: radius of planet = R = 2000 km = 2 x 10⁶ m, Acceleration due to gravity = 2.5 m/s².

To Find: v_e =?

Solution:

Ans: Escape velocity on the planet is 3.162 km/s

Example – 05:

A satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the earth. Find its height above the earth’s surface.

Given: v_c =1/2 v_e

To Find: height of satellite above the surface of earth = h =?

Solution:

Ans: The height above the earth’s surface is ‘R’.

Example – 06:

Taking the mass of moon as 7.35 x 10²² kg and radius as 1750 km and G = 6.67 x 10^-11 Nm² /kg², find g at the surface of the moon and the escape velocity of a body from the surface of the moon.

Given: Mass of moon = M = 7.35 x 10²² kg, Radius of moon = R = 1750 km = 1.750 x 10⁶ m, G = 6.67 x 10^-11 Nm² /kg²,

To find: acceleration due to gravity =? v_e = ?

Solution:

Ans: Acceleration on the surface of moon is 1.6 m/s², Escape velocity on the planet is 2.367 km/s

Example – 07:

A satellite is revolving around the earth in a circular orbit of radius 7000 km. Calculate its period given that the escape velocity from the earth’s surface is 11.2 km/s and g = 9.8 ms/s²

Given: radius of orbit = r = 7000 km = 7 x 10⁶ m, g = 9.8 ms/s², escape velocity = v_e = 11.2 km/s = 11.2 x 10³ m/s,

To find: Period = T =?

Solution:

Ans: Thus the period of the satellite is 5809 s or 1.61 hr

Example – 08:

The mass of the moon is 1/80th that of the earth and the diameter of the moon is 1/4th that of the earth. Given that the escape velocity from the earth’s surface 11.2 km/s, find that from the moon’s surface.

Given: mass of moon = 1/80 mass of earth i.e. M_M = 1/80M_E, Diameter of moon = 1/4 diameter of earth i.e. R_M = 1/4 R_E, V_eE = 11.2 km/s

To find:  V_eM=?

Solution:

Dividing equation (1) by (2) we have

Ans: Escape velocity on the surface of the moon is 2.504 km/s

Example – 09:

A planet A has a mass and radius twice that of planet B, find the ratio of the escape velocities from A & B

Given: M_A = 2 M_B, R_A = 2 R_B,

To find: the ratio of escape velocities =?

Solution:

Dividing equation (1) by (2) we have

Ans: The ratio of escapes velocities on the two planets is 1: 1

Example – 10:

The escape velocity from the earth’s surface is 11.2 km/s. If the mass of Jupiter is 318 times that of earth and its radius is 11.2 times that of earth, find the escape velocity from Jupiter’s surface.

Given: M_J = 318 M_E, R_J = 11.22 R_E, escape velocity on surface earth = v_eE = 11.2 km/s

To find:   v_eJ =?

Solution:

Dividing equation (1) by (2) we have

Ans: The escape velocity on the surface of Jupiter is 59.68 km/s

Previous Topic: Binding Energy of Satellite

For More Topics in Physics Click Here

Science > Physics > Gravitation > Escape Velocity of a Body