Packing in solids

Science > Chemistry > Solid State > Packing in solids

In this article, we shall study the different types of close packing of the constituent particles in solids.

The Principle of Packing in Solids:

In a crystal particle (atoms, molecules or ions) occupy the lattice points in the crystal lattice. These particles may be of various shapes and hence mode of packing of these particles will change according to their shape.

Constituent particles are considered rigid incompressible spheres of equal size. The constituent particles try to get closely packed together so that the maximum closely packed structure is attained. The maximum closely packed structure is such that the minimum empty space (void) is left. The closer the constituent particles lie, the greater is the stability of the packed system. By this arrangement, a maximum possible density of the crystal and stability of the crystal is achieved.

Close Packing in One Dimension:

There is only one way of arranging spheres in a one-dimensional close-packed structure, that is to arrange them in a row and touching each other.

In this arrangement, each sphere is in contact with two of its neighbours. The number of nearest neighbours of a particle is called its coordination number. Thus, in a one-dimensional close-packed arrangement, the coordination number is 2.

Close Packing in Two Dimensions:

A two-dimensional close-packed structure can be generated by placing the rows of close-packed spheres side by side horizontally. This can be done in two different ways.

a) Square Close Packing:

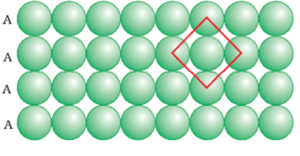
The second row may be placed in contact with the first one such that the spheres of the second row are exactly above those of the first row. The spheres of the two rows are aligned horizontally as well as vertically. If we call the first row as ‘A’ type row, the second row being exactly the same as the first one, is also of ‘A’ type. Similarly, we may place more rows to obtain AAA type of arrangement.

In this arrangement, each sphere is in contact with four of its neighbours. Thus, the two-dimensional coordination number is 4. Also, if the centres of these 4 immediate neighbouring spheres are joined, a square is formed. Hence this packing is called square close packing in two dimensions.

b) Hexagonal Close Packing:

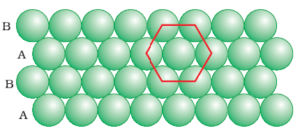
In this arrangement, the second row may be placed above the first one in a staggered manner such that its spheres fit in the depressions of the first row.

If the arrangement of spheres in the first row is called ‘A’ type, the one in the second row is different and may be called ‘B’ type. When the third row is placed adjacent to the second in a staggered manner, its spheres are aligned with those of the first row. Hence this row is also of ‘A’ type. The spheres of the similarly placed fourth row will be aligned with those of the second row (‘B’ type). Hence this arrangement is of ABAB type.

In this arrangement, there is less free space and this packing is more efficient than the square close packing. Each sphere is in contact with six of its neighbours and the two-dimensional coordination number is 6. The centres of these six spheres are at the corners of a regular hexagon hence this packing is called two-dimensional hexagonal close packing.

It can be seen in the figure that in these rows there are some voids (empty spaces). These are triangular in shape. The triangular voids are of two different types. In one row, the apexes of the triangles are pointing upwards and in the next layer downwards.

Close Packing in Three Dimensions:

A three-dimensional close-packed structure can be generated by stacking (placing) layers of close-packed spheres in two dimensions one over the other. This can be done in the following ways.

Three-dimensional close packing from a two-dimensional square layer:

In such an arrangement, the second layer is placed over the first layer such that the spheres of the upper layer are exactly above those of the first layer. In this arrangement spheres of both the layers are perfectly aligned horizontally as well as vertically as shown. Similarly, we may place more layers one above the other.

If the arrangement of spheres in the first layer is called ‘A’ type, all the layers have the same arrangement. Thus this lattice has AAA…. type pattern. The lattice thus generated is the simple cubic lattice, and its unit cell is the primitive cubic unit cell.

The coordination number for such arrangement is 6. The volume occupied by particles is 52% i.e. there is 48% void space. Of all metals in the periodic table, only polonium crystalizes in this crystal form.

Three-dimensional close packing from two-dimensional hexagonal close-packed layers:

This close-packed structure can be generated by placing layers one over the other.

All the voids are equivalent in the first layer they have marked alternately as ‘a’ and ‘b’. The spheres of the second layer can be either placed on voids which are marked ‘a’ or ‘b’ but it is impossible to place spheres on both types of voids simultaneously. When spheres of a new layer are placed on voids marked ‘a’ then voids marked as ‘b; remain unoccupied.

Let us take a two-dimensional hexagonal close-packed layer ‘A’ and place a similar layer above it such that the spheres of the second layer are placed in the depressions of the first layer. Since the spheres of the two layers are aligned differently, let us call the second layer as B.

T = Tetrahedral void and O = Octahedral void

Placing the third layer over the second layer can be done in two ways.

It can be observed from the figure that not all the triangular voids of the first layer are covered by the spheres of the second layer.

This gives rise to two different types of holes or voids. Wherever a sphere of the second layer is above the void of the first layer (or vice versa) a tetrahedral void is formed. These voids are called tetrahedral voids because a tetrahedron is formed when the centres of these four spheres are joined. At other places, the triangular voids in the second layer are above the triangular voids in the first layer, and the triangular shapes of these do not overlap. One of them has the apex of the triangle pointing upwards and the other downwards. These voids have been marked as ‘O’ in the figure above.

Covering Tetrahedral Voids (Hexagonal Closed Packing):

Tetrahedral voids of the second layer may be covered by the spheres of the third layer. In this case, the spheres of the third layer are exactly aligned with those of the first layer. Thus, the pattern of spheres is repeated in alternate layers. This pattern is often written as ABAB ……. pattern. This structure is called a hexagonal close-packed (hcp) structure.

This sort of arrangement of atoms is found in many metals like magnesium and zinc.

B) Covering Octahedral Voids (Cubic Closed Packing):

The third layer may be placed above the second layer in a manner such that its spheres cover the octahedral voids. When placed in this manner, the spheres of the third layer are not aligned with those of either the first or the second layer. This arrangement is called ‘C’ type.

Only when the fourth layer is placed, its spheres are aligned with those of the first layer as shown. This pattern of layers is often written as ABCABC ……….. This structure is called cubic close-packed (ccp) or face-centred cubic (fcc) structure. Metals such as copper and silver crystallise in this structure.

Note:

Both these types of close packing are highly efficient and 74% space in the crystal is filled. In either of them, each sphere is in contact with twelve spheres. Thus, the coordination number is 12 in either of these two structures.

Characteristics of Hexagonal Close Packing:

• In this three dimensional arrangement of the unit cell, spheres of the third layer are placed on triangular-shaped tetrahedral voids of the second layer.
• The spheres of the third layer lie exactly above the spheres of the first layer.
• The arrangements of the first layer and third layer are identical.
• The arrangement of hexagonal close packing is represented as ABAB type.
• Packing efficiency is 74%

Characteristics of Cubic Close Packing:

• In this three dimensional arrangement of the unit cell, spheres of the third layer are placed in the positions of tetrahedral voids having apices upward.
• In this arrangement, the spheres of the third layer do not lie exactly above the spheres of the first layer. Actually, the spheres of the fourth layer lie exactly above the spheres of the first layer.
• The arrangements of the first layer and third layer are different. The arrangements of the first layer and fourth layer are identical.
• The arrangement of cubic close packing is represented as ABCABC type.
• Packing efficiency is 74%

Science > Chemistry > Solid State > Packing in solids