Signs of Trigonometric Ratios

In this article, we shall study to find signs of trigonometric ratios (Functions) in different quadrants formed due to co-ordinate axes.

Signs of Trigonometric Ratios in Different Quadrants:

θ lies in Quadrant →	I	II	III	IV
Trigonometric Functions↓
sin θ	+ ve	+ ve	– ve	– ve
cos θ	+ ve	– ve	– ve	+ ve
tan θ	+ ve	– ve	+ ve	– ve
cosec θ	+ ve	+ ve	– ve	– ve
sec θ	+ ve	– ve	– ve	+ ve
cot θ	+ ve	– ve	+ ve	– ve

Diagram Showing Signs of Trigonometric Ratios in Different Quadrants

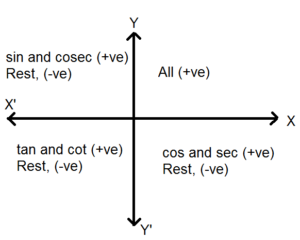
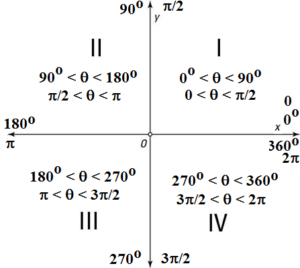
Diagram showing value of angle in different quadrants:

Examples Based on Signs of Trigonometric Ratios:

Example – 01:

State the signs of following Trigonometric Ratios (Functions)

sin 675^o

sin 675 ^o = sin (360^o + 315^o) = sin (315^o) = sin (270^o + 45^o)

Thus the angle 675^o lies in the fourth quadrant, where sin function is negative.

Hence sin 675 ^o is negative

sin 159^o

sin 159 ^o = sin (90^o + 69^o)

Thus the angle 159^o lies in second quadrant, where sin function is positive.

Hence sin 159 ^o is positive

cos 573 ^o

cos 573 ^o = cos (360^o + 213^o) = cos (213^o) = sin (180^o + 33^o)

Thus the angle 573^o lies in the third quadrant, where cos function is negative.

Hence cos 573 ^o is negative

cos 250 ^o

cos 250 ^o = cos (180^o + 70^o)

Thus the angle 250^o lies in the third quadrant, where cos function is negative.

Hence cos 250 ^o is negative

cos (-8π/3)^c

cos(-8π/3)^c = cos (-2π – 2π/3) = cos (- 2π/3) =  cos (- π/2 – π/6)

Thus the angle (-8π/3)^c lies in the third quadrant, where cos function is negative.

Hence cos(-8π/3)^c is negative

cos (11π/9)^c

cos(11π/9)^c = cos (π + 2π/9)

Thus the angle (11π/9)^c lies in the third quadrant, where cos function is negative.

Hence cos(11π/9)^c is negative

sec (3π/5)^c

sec(3π/5)^c = sec (π/2 + π/10)

Thus the angle (3π/5)^c lies in the second quadrant, where sec function is negative.

Hence sec(3π/5)^c is negative

Example – 02:

Determine the quadrant in which θ lies from following trigonometric functions.

sin θ > 0 and sec θ < 0

Given, sin θ > 0 and sec θ < 0. i.e. sin θ is positive and sec θ is negative

Hence θ lies in the second quadrant.

cos θ < 0 and cot θ > 0

Given, cos θ < 0 and cot θ > 0, i.e. cos θ is negative and cot θ is positive

Hence θ lies in the third quadrant.

sec θ > 0 and cosec θ < 0

Given, sec θ > 0 and cosec θ < 0, i.e. sec θ is positive and cosec θ is negative

Hence θ lies in the fourth quadrant.

Example – 04:

If tan θ= -2 and θ lies in second quadrant, find values of other trigonometric functions.

Solution:

Given tan θ = -2

We have, 1+ tan²θ = sec²θ

∴  1+ (-2)² = sec²θ

∴  5 = sec²θ

∴  sec θ = ± √5

Now θ lies in second quadrant where sec ratio is negative.

∴  sec θ = – √5

∴  cos θ = – 1/√5

Now, tan θ = sin θ / cos θ

∴  sin θ = tan θ x cos θ = (-2) x ( – 1/√5)  = 2/√5

Now, cot  θ = 1/ tan θ = 1/-2 = – 1/2

cosec θ = 1/sin θ = 1/(2/√5) = √5/2

Example – 05:

If tan θ = 5/12 and π < θ < 3π/2, find values of other trigonometric functions.

Solution:

Given tan θ = 5/12 and π < θ < 3π/2

We have, 1+ tan²θ = sec²θ

∴  1+ (5/12)² = sec²θ

∴  1 + 25/144 = sec²θ

∴  (144 + 25)/144 = sec²θ

∴ 169/144 = sec²θ

∴  sec θ = ± 13/12

Now π < θ < 3π/2, hence θ lies in the third quadrant where sec ratio is negative.

∴  sec θ = – 13/12

∴  cos θ =1/(- 13/12) = – 12/13

Now, tan θ = sin θ / cos θ

∴  sin θ = tan θ x cos θ = (5/12) x ( – 12/13)  =  – 5/13

Now, cot  θ = 1/ tan θ = 1/( 5/12 )= 12/5

cosec θ = 1/sin θ = 1/(- 5/13) = – 13/5

Example – 06:

If cos θ= 4/5 and 3π/2 < θ < 2π, find values of other trigonometric functions.

Solution:

Given cos θ= 4/5 and 3π/2 < θ < 2π

We have, sin²θ + cos²θ = 1

∴  sin²θ + (4/5)² = 1

∴  sin²θ = 1 – 16/25

∴  sin²θ = (25 – 16)/25

∴  sin²θ = 9/25

∴  sin θ = ± 3/5

Now 3π/2 < θ < 2π, hence θ lies in the fourth quadrant where sin ratio is negative.

∴  sin θ = – 3/5

Now, tan θ = sin θ / cos θ

∴  tan θ = (- 3/5) / (4/5) = – 3/4

Now, cosec θ = 1/sin θ = 1/(- 3/5) = – 5/3

sec θ = 1/cos θ = 1/(4/5) = 5/4

cot  θ = 1/ tan θ = 1/( -3/4 )= – 4/3

Example – 07:

If tan θ=  – 4/3 and 3π/2 < θ < 2π, find values of 3 sec θ + 5 tan θ.

Solution:

Given tan θ = – 4/3 and 3π/2 < θ < 2π

We have, 1+ tan²θ = sec²θ

∴  1+ (-4/3)² = sec²θ

∴  1 + 16/9 = sec²θ

∴  (9 + 16)/9 = sec²θ

∴ 25/9 = sec²θ

∴  sec θ = ± 5/3

Now 3π/2 < θ < 2π, hence θ lies in the fourth quadrant where sec ratio is positive.

∴  sec θ = 5/3

Now, 3 sec θ + 5 tan θ = 3 x (5/3) + 5 x (-4/3) = 5 – 20/3 = (15 – 20)/3 = – 5/3

Example – 08:

If sec θ= √2 and 3π/2 < θ < 2π, find values of

Solution:

Given sec θ= √2 and 3π/2 < θ < 2π

We have,1 + tan²θ = sec²θ

∴  1 + tan²θ = (√2)²

∴  1 + tan²θ = 2

∴  tan²θ = 1

∴  tan θ = ± 1

Now 3π/2 < θ < 2π, hence θ lies in the fourth quadrant where tan ratio is negative.

∴  tan θ = – 1

Now cot θ = 1/tan θ = 1 /(-1) = -1

cos θ = 1/sec θ = 1/√2

tan θ = sin θ / cos θ

∴  sin θ = tan θ x cos θ = (-1) x ( 1/√2)  =  – 1/√2

cosec θ = 1/sin θ = 1/(- 1/√2) = – √2

Example – 09:

If cos θ= – 3/5 and π < θ < 3π/2, find value of

Solution:

Given cos θ= – 3/5 and π < θ < 3π/2

We have, sin²θ + cos²θ = 1

∴  sin²θ + (- 3/5)² = 1

∴  sin²θ = 1 – 9/25

∴  sin²θ = (25 – 9)/25

∴  sin²θ = 16/25

∴  sin θ = ± 4/5

Now π/ < θ < 3π/2, hence θ lies in the thir quadrant where sin ratio is negative.

∴  sin θ = – 4/5

Now, tan θ = sin θ / cos θ

∴  tan θ = (- 4/5) / (- 3/5) = 4/3

Now, cosec θ = 1/sin θ = 1/(- 4/5) = – 5/4

sec θ = 1/cos θ = 1/(- 3/5) = – 5/3

cot  θ = 1/ tan θ = 1/( 4/3 )= 3/4

Example – 10:

If  5 tan A = √7  where π < A  < 3π/2 and sec B =  √11  where 3π/2 < B  < 2π, find value of cosec A – tan B.

Solution:

Given 5 tan A = √7  where π < A  < 3π/2

∴ tan A = √7/5

∴ cot A = 5/√7

We have,1 + cot²A = cosec²A

∴  1 + (5/√7)² = cosec²A

∴  1 + (25/7) = cosec²A

∴  (7 + 25)/7 = cosec²A

∴  cosec²A = 32/7

∴ cosec A = ±  √32/√ 7 = ± 4√2/√ 7

Now π < A < 3π/2, hence A lies in the third quadrant where cosec ratio is negative.

cosec A = – 4√2/√ 7

Given sec B =  √11  where 3π/2 < B  < 2π

We have,1 + tan²B = sec²B

∴  1 + tan²B = (√11)²

∴  1 + tan²B = 11

∴  tan²B = 10

∴  tan B = ± √10

Now 3π/2 < B < 2π, hence B lies in the fourth quadrant where tan ratio is negative.

∴  tan B = – √10

The value of the quantity cosec A – tan B =  – 4√2/√7 – √10

Example – 11:

Find the value of 4 cos A + 3 cos B if angles A and B lies in second quadrants and

Solution:

sin A = 3/5 and sin B = 4/5

We have, sin²A + cos²A = 1

∴ (3/5)² + cos²A = 1

∴ cos²A = 1 – 9/25

∴  cos²A = (25 – 9)/25

∴  cos²A = 16/25

∴  cosA = ± 4/5

Now A lies in the second quadrant where cos ratio is negative.

∴  cosA = – 4/5

sin B = 4/5

We have, sin²B + cos²B = 1

∴ (4/5)² + cos²B = 1

∴ cos²B = 1 – 16/25

∴  cos²B = (25 – 16)/25

∴  cos²B = 9/25

∴  cosB = ± 3/5

Now A lies in the second quadrant where cos ratio is negative.

∴  cosA = – 3/5

The value of

4 cos A + 3 cos B = 4 x (- 4/5) + 3 x (- 3/5)= -16/5 – 9/5 = -25/5 = -1

Example – 12:

If 2 sin x = 1, π/2 < x < π and √2 cos y = 1, 3π/2 < y < 2π, find the value of

Solution:

2sin x = 1, hence sin x = 1/2, π/2 < x < π

We have, sin²x + cos²x = 1

∴ (1/2)² + cos²x = 1

∴ cos²x = 1 – 1/4

∴  cos²x = (4-1)/4

∴  cos²x = 3/4

∴  cos x = ± √3/2

Now x lies in the second quadrant where cos ratio is negative.

∴  cos x = – √3/2

Now, tan x = sin x/ cos x = (1/2)/(- √3/2) = – 1/√3

√2 cos y = 1, hence cos y = 1/√2,  3π/2 < y < 2π

We have, sin²y + cos²y = 1

∴ sin²y + (1/√2)² = 1

∴sin²y = 1 – 1/2

∴  sin²y = (2 – 1)/2

∴  sin²y = 1/2

∴  sin y = ±  1/√2

Now y lies in the third quadrant where sin ratio is negative.

∴  sin y = –  1/√2

Now, tan y = sin y/ cos y = ( –  1/√2)/(1/√2) = – 1

Example – 13:

If cos A = sin B = -1/3, where π/2 < A < π and  π < B< 3π/2, then find the value of

Solution:

Given cos A = -1/3, π/2 < x < π

We have, sin²A + cos²A = 1

∴ sin²A + (-1/3)² = 1

∴ sin²A = 1 – 1/9

∴  sin²A = (9-1)/9

∴  sin²A = 8/9

∴  sin A = ± 2√2/3

Now A lies in the second quadrant where sin ratio is positive.

∴  sin A = 2√2/3

Now, tan A = sin A/ cos A = (2√2/3) / (- 1/3)= – 2√2

sin B = – 1/3, π < B< 3π/2

We have, sin²B + cos²B = 1

∴(- 1/3)² + cos²B = 1

∴ cos²B = 1 – 1/9

∴  cos²B = (9 – 1)/9

∴  cos²B = 8/9

∴  cos B = ± 2√2/3

Now y lies in the second quadrant where cos ratio is negative.

∴  cos B = –  2√2/3

Now, tan B = sin B/ cos B = ( –  1/3)/(-  2√2/3) =  1/2√2