Science > Physics > Surface Tension > Capillary Action
A capillary tube is a tube with a very fine bore. The phenomenon of a rise and fall of a liquid inside a capillary tube when it is dipped in the liquid is called capillary action or capillarity. When a glass capillary is immersed in water, the water rises inside the tube. When a glass tube is immersed in mercury, the level of mercury inside the tube falls below the level in the outer vessel.
Examples of capillarity: Blotting paper absorbs ink or water, ink rises in a pen, oil rises in wicks of a lamp.
When chalk piece is immersed in water, bubbles are emitted:
Chalk is porous in nature. In these pores, the air is trapped. When chalk piece is dropped in water, due to capillary action water enters the pores of the chalk. Due to which the trapped air comes out of the pores in the form of bubbles.
Everyday Examples of Capillarity:
- Due to capillary action oil rises through wicks of lamps.
- Due to capillary action water rises through the sap of trees.
- Due to capillary action ink is absorbed by blotting paper.
- Due to capillary action liquids are absorbed by sponges.
- Bricks and mortar, which are porous, permit the rise of soil water through them by capillary action. To avoid it, the base is made using damp-proof cement.
- Soil water rises to the surface by capillary action and evaporates. To conserve soil water, the farmers plough their fields in summer. The loosening of the top layer of the soil breaks up the fine capillaries through which the deeper down water is sucked up. Thus the loss of water in the soil is prevented.
- Due to depression in the level of mercury in a capillary tube, a correction is to be applied for mercury barometer.
Capillary Action in Water:
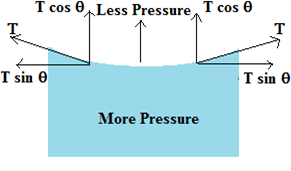
We know that when a liquid is taken in a vessel, the free surface of the liquid near the walls becomes curved either concave upwards or convex upwards.
In the case of the capillary tube, its bore is very small hence all the points on the free surface are very close to the walls, and hence, the entire free surface becomes curved. It is called a meniscus.
When a glass capillary immersed in water, the meniscus is concave upwards. The air pressure on the upper or concave side of the meniscus is the atmospheric pressure P. We know that the pressure on the convex side of the free surface is less than that on the concave side. It means that the pressure just below the meniscus i.e. on the convex side of the meniscus is less than atmospheric pressure.
The pressure at points just below the flat surface of the water in the surrounding vessel is atmospheric pressure. The system is, therefore not in equilibrium and the liquid rises in the capillary tube till the equilibrium is reached.

Capillary Action in case of Mercury:

We know that when a liquid is taken in a vessel, the free surface of the liquid near the wall becomes curved either concave upwards or convex upwards.
In case of a capillary tube, its bore is very small hence all the points on the free surface are very close to the walls, and hence, the entire free surface becomes curved. It is called a meniscus.
When a glass capillary immersed in mercury, the meniscus is convex upwards. The air pressure on the upper or convex side of the meniscus is the atmospheric pressure P. We know that the pressure on the concave side of the free surface is greater than that on the convex side. It means that the pressure just below the meniscus i.e. on the concave side is more than atmospheric pressure.
The pressure of points just below the flat surface of the mercury in the surrounding vessel is atmospheric pressure. The system is therefore not in equilibrium and the liquid descends in the capillary tube till equilibrium is reached.

Expression for the Rise in the Liquid in a Capillary Tube:
If a glass tube of a smaller bore (capillary tube) is immersed in a liquid which wets the glass (water), then the liquid level inside the tube rises. If the tube is immersed in a liquid which does not wet the glass (mercury), then the liquid level inside the tube decreases. This phenomenon of the rise or fall of liquid in a capillary tube is called capillary action or capillarity.
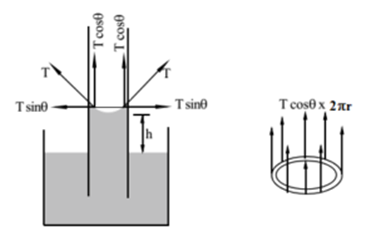
Consider a capillary tube immersed in a liquid that wets it. The liquid will rise in the capillary tube. The surface of the liquid will be concave.

The surface tension ‘T’ acts along the tangent to the liquid surface at the point of contact as shown. Let θ be the angle of contact. The force of surface tension is resolved into two components vertical Tcos θ and horizontal T sin θ. The components Tsinθ cancel each other as they are equal in magnitude and radially outward (opposite to each other). The unbalanced component T cos θ will push the liquid up into the capillary tube. This explains the rise in the liquid layer in the capillary tube.
If ‘r’ is the radius of the bore of the capillary tube, the length along which the force of surface tension acts is 2πr. Hence total upward force is 2πr T cos θ.
Due to this force the liquid rise up in the tube. The weight of liquid acts vertically downward. The liquid goes on rising till the force of surface tension is balanced by the weight of the liquid column.
Total upward force = Weight of liquid in the capillary tube.
2πr T cos θ = mg
Where ‘m’ is the mass of liquid in the capillary tube.
2πr T cos θ = V ρ g
Where ‘V’ is the volume of liquid in capillary and ρ is the density of the liquid in the capillary tube.
2πr T cos θ = π r²h ρ g
Where ‘h’ is the height of the liquid column in the capillary tube, then

This is an expression for the rise in the liquid in the capillary tube.
Factor’s Affecting the Rise in a Capillary Tube:
The height to which the liquid rises in the capillary tube is
- inversely proportional to the radius of the bore of the capillary tube.
- inversely proportional to the density of the liquid
- directly proportional to the surface tension of the liquid.
Important Observations:
- For those liquids whose angle of contact is acute, the liquid rises in the capillary tube.
- For those liquids whose angle of contact is obtuse, the liquid depresses in the capillary tube.
- For a particular liquid-solid interface, h r = constant. This relation is known as Jurin’s Law.
Determination of the Surface Tension of Liquid:
A glass capillary tube is dipped in a beaker containing liquid (say water) whose surface tension is to be measured. Due to surface tension, the liquid level rises up inside the capillary. The phenomenon is known as capillary action or capillarity.
Using a travelling microscope, the microscope is focused on the lower meniscus of the curved concave surface. The reading is taken using the microscope. The microscope is lowered and it is focused on the water level inside the beaker. The reading is taken again. The difference between the two readings gives the height of the water column inside capillary (h). The inner diameter of the capillary tube is measured using the travelling microscope. Hence we calculate the radius of the bore (r).
Using the following formula Surface tension (T) can be calculated.

where h = height of liquid level in the capillary
T = Surface tension
ρ = Density of liquid
r = Radius of the bore of the capillary tube
g = Acceleration due to gravity
θ = Angle of contract
Previous Topic: Laplace’s Law of Spherical Membrane
Next Topic: Numerical Problems on Capillary Action