Concept of Gravitational Potential

Science > Physics > Gravitation > Concept of Gravitational Potential

In this article, we shall discuss the concept of the gravitational potential at a point in a gravitational field. It is defined as the work done in bringing the unit mass from infinity to that point without acceleration. Considering magnitude only.

V_G = W / m

It is a scalar quantity. It is denoted by V. Its S.I. unit is J kg^-1. Its c.g.s. unit is erg g^-1.

Its dimensions are [M⁰ L² T^-2].

Expression for Gravitational Potential:

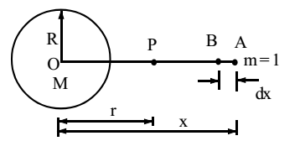
R = Radius of the Erath

Let M be the mass of the Erath. Let P be the point at a distance of ‘r’ (r > R) at which the potential is to be calculated. Let A be the point at a distance of ‘x’ from the centre of the Earth O. Let us consider a unit mass at A. The Force acting on the unit mass is given by

Let the mass m be moved from A to B without acceleration through a very small distance ‘dx’. The work done is given by

Total work done can be obtained by integrating the above expression

This work is gravitational potential at that point which is given by

Where G is universal gravitation constant and M is the mass of the earth.

Characteristics of Gravitational Potential:

• As gravitational field intensity is zero at infinity and it goes on decreasing as the test mass approach the attracting body. Thus the body moves from higher potential to lower potential, hence gravitational potential is a negative quantity.
• It is maximum at infinity (zero).
• It is a scalar quantity.
• Its dimensions are [M⁰ L² T^-2].
• Its S.I. unit is J kg^-1. Its c.g.s. unit is erg g^-1.
• If its value at all the points on the surface is the same, then the surface is called an equipotential surface.
• It can be studied in analogy with the electrical potential. As the current moves from higher electrical potential to the lower electrical potential, similarly mass moves from higher potential to the lower potential.

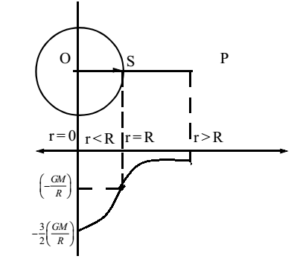
Gravitational Potential due to the Earth at Different Points:

The same formulae are applicable to a solid sphere

Gravitational Potential due to a Spherical Shell (Hollow Sphere) at Different Points:

Gravitational Potential Difference:

It is defined as the work done to move unit mass from one point to the other in the gravitational field.

Potential Energy at a Point:

The gravitational potential energy at a point is defined as the work done in bringing the unit mass from infinity to that point without acceleration. This work is stored as the potential energy of that mass.

The expression for Gravitational Potential Energy at a Point in a Gravitational Field:

Let M be the mass of the Earth. Let P be the point at a distance of ‘r’ (r > R) at which gravitational potential energy is to be calculated. Let A be the point at a distance of ‘x’ from the centre of the Earth O. Let us consider a mass ‘m’ at A. The Force acting on the unit mass is given by

Let the mass be moved from A to B without acceleration through very small distance dx. The work done is given by

This work is stored as potential energy in the body.

Thus gravitational potential energy = Gravitational potential at a point x Mass of the body at that point. Where G is universal gravitation constant and M is the mass of the earth and r is the distance of the body from the centre of the earth.

Note:

Conventionally gravitational potential energy on the surface of the earth is considered to be zero. Thus the surface of the earth is equipotential as it is same (zero) all over the surface.

Relation Between Gravitational Intensity and Gravitational Potential Difference:

Let us consider an isolated mass M. Let A be the point in the field at a distance of x from the centre of the isolated mass.

Let the body be moved from A to B through small distance dx as shown. Then E is the force experienced by unit mass placed at A. Then work done in moving the mass from A to B is given by

dW = – E.dx

This work is equal to the potential difference dV between points A and B.

Hence, dV = – E.dx

Hence E = dV/dx   in magnitude

Note:

The concept of the gravitational field, gravitational intensity, and gravitational potential was not introduced by Newton. Actually, such a concept was developed by Michael Faraday (1791-1867) in electricity and magnetism. In gravitation, it is used in an analogy.

Gravitational Potential Energy of mass m at Height h above the surface of the Earth:

The gravitational-potential energy of a body at a distance of r from the centre of the Erath is given by

Where     r = R + h

R = Radius of the Earth

h = Height of the body above the surface of the Earth.

Potential energy = Gravitational-potential x mass of the body

Work Done in Raising a Body From the Surface of the Earth to a Height ‘h’:

Where m = Mass of the body

g = acceleration due to gravity.

R = Radius of the planet.

Special Case: When h = R

Gravitational Self Energy:

The gravitational self-energy of a body (or a system of particles) is defined as work done by an external agent in assembling the body (or the system of particles) from infinitesimal elements (or particles) that are initially at the infinite distance apart

Where Us= Gravitational self-energy

G = Universal gravitational constant

n = Number of particles

m = Mass of each particle

r = Average distance between two particles

Previous Topic: Concept of Gravitational Potential

Next Topic: Acceleration Due to Gravity

Science > Physics > Gravitation > Concept of Gravitational Potential