Science > Physics > Current Electricity > Numerical Problems on Potential Drop
In this article, we shall study to solve numerical problems on the calculation of potential drop (potential gradient) on potentiometer wire.
Example 01:
A potentiometer wire is 10 m long and a P.D. of 6 V is maintained between its ends. Find the potential drop per centimeter of the wire. Also, find the e.m.f. of a cell which balances against a length of 180 cm of the wire.
Given: Length of potentiometer wire = lAB = 10 m = 1000 cm, P.D. across potentiometer wire = VAB = 6 V, Balancing length = l = 180 cm
To Find: Potential drop per cm =? e.m.f. of cell =?
Solution:
Potential drop per cm = VAB/lAB in cm = 6/1000 = 0.006 V cm-1
E.m.f. of cell = Potential drop per cm x Balancing length in cm
E.m.f. of cell = 0.006 x 180 = 1.08 V
Ans: Potential drop = 0.006 V cm-1 and e.m.f. of cell = 1.08 V
Example 02:
A potentiometer wire is 8 m long and a P.D. of 10 V is maintained between its ends. Find the potential drop of the wire. Also find the e.m.f. of a cell which balances against a length of 208 cm of the wire.
Given: Length of potentiometer wire = lAB = 8 m, P.D. across potentiometer wire = VAB = 10 V, Balancing length = l = 208 cm = 2.08 m
To Find: Potential drop =? e.m.f. of cell =?
Solution:
Potential drop = VAB/lAB = 10/8 = 1.25 V m-1
E.m.f. of cell = Potential drop x Balancing length
E.m.f. of cell = 1.25 x 2.08 = 2.6 V
Ans: Potential drop = 1.25 V m-1 and e.m.f. of cell = 2.6 V
Example 03:
A potentiometer wire has a length of 2 m and a resistance of 10 ohm. It is connected in series with a cell of e.m.f. 4 V and internal resistance 6 ohm. Find the potential gradient on the wire. Find also where a cell of e.m.f. 1 volt will balance on the wire.
Given: Length of potentiometer wire = lAB = 2 m, Resistance of potentiometer wire = RAB = 10 ohm, e.m.f. of cell = E = 4 V, Internal resistance of cell = r = 6 ohm, e.m.f. of cell = e = 1 V
To Find: Potential gradient =? Balancing length = l =?
Solution:
I = E/(RAB + r) = 4/(10 + 6) = 4/16 = 0.25 A
VAB = I RAB = 0.25 x 10 = 2.5 V
Potential gradient = VAB/lAB = 2.5/2 = 1.25 V m-1
E.m.f. of cell = Potential drop x Balancing length
1 = 1.25 x lAB
lAB = 1/1.25 = 0.8 m
Ans: Potential gradient = 1.25 V m-1 and balancing length = 0.8 m
Example 04:
A potentiometer wire has a length of 4 m and a resistance of 10 ohm. It is connected in series with a cell of e.m.f. 4 V and internal resistance 2 ohm. Find the potential gradient on the wire. Find also where a cell of e.m.f. 1.5 volt will balance on the wire.
Given: Length of potentiometer wire = lAB = 4m, Resistance of potentiometer wire = RAB = 10 ohm, e.m.f. of cell = E = 4 V, Internal resistance of cell = r = 2 ohm, e.m.f. of cell = e = 1.5 V
To Find: Potential gradient =? Balancing length = l =?
Solution:
I = E/(RAB + r) = 4/(10 + 2) = 4/12 = (1/3) A
VAB = I RAB = (1/3) x 10 = (10/3) V
Potential gradient = VAB/lAB = (10/3)/4 = 10/12 = 5/6 = 0.8333V m-1
E.m.f. of cell = Potential drop x Balancing length
1.5 = (5/6) x lAB
lAB = (1.5 x 6)/5 = 1.8 m
Ans: Potential gradient = 0.8333 V m-1 and balancing length = 1.8 m
Example 05:
A potentiometer wire has a length of 2 m and a resistance of 10 ohm. It is connected in series with a cell of e.m.f. 2 V and a resistance 990 ohm. Find the potential gradient on the wire.
Given: Length of potentiometer wire = lAB = 2m, Resistance of potentiometer wire = RAB = 10 ohm, e.m.f. of cell = E = 2 V, Resistance in series = R = 990 ohm.
To Find: Potential gradient =?
Solution:
I = E/(RAB + R) = 2/(10 + 990) = 2/1000 = 0.002 A
VAB = I RAB = 0.002 x 10 = 0.02 V
Potential gradient = VAB/lAB = 0.02/2 = 0.01 V m-1
Ans: Potential gradient = 0.01 V m-1
Example 06:
A potentiometer wire has a length of 2 m and a resistance of 5 ohm. It is connected in series with a cell of e.m.f. 2 V and internal resistance 2 ohm and resistance of 993 ohm. Find the potential gradient on the wire. Find also where a cell of e.m.f. 4 mV will balance on the wire.
Given: Length of potentiometer wire = lAB = 2 m, Resistance of potentiometer wire = RAB = 5 ohm, e.m.f. of cell = E = 2 V, Internal resistance of cell = r = 2 ohm, Resistance in series = R = 993 ohm, e.m.f. of cell = e = 4mV = 4 x 10-3 V
To Find: Potential gradient =? Balancing length = l =?
Solution:
I = E/(RAB + r + R) = 2/(5 + 2 + 993) = 2/1000 = 2 x 10-3 A
VAB = I RAB = 2 x 10-3 x 5 = 0.01 V
Potential gradient = VAB/lAB = 0.01 /2 = 5 x 10-3 V m-1
E.m.f. of cell = Potential drop x Balancing length
4 x 10-3 = 5 x 10-3 x lAB
lAB = 4 x 10-3 /5 x 10-3 = 0.8 m
Ans: Potential gradient = 5 x 10-3 V m-1 and balancing length = 0.8 m
Example 08:
A potentiometer wire of length 5 m has a resistance of 5 ohm. What resistance must be connected in series with this wire and an accumulator of e.m.f. 4 volt so as to get a potential drop of 10-2 Vm-1 on the wire?
Given: Length of potentiometer wire = lAB = 5 m, Resistance of potentiometer wire = RAB = 5 ohm, e.m.f. of cell = E = 4 V, Resistance in series = R ohm, Potential drop = 10-2 V m-1.
To Find: Resistance R =?
Solution:
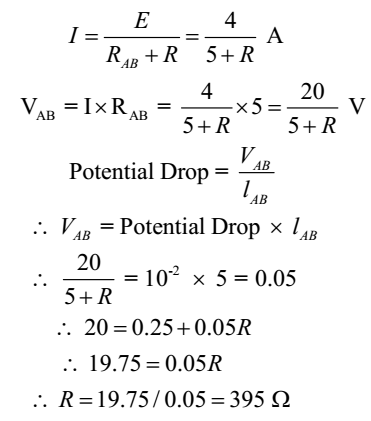
Ans: A resistance of 395 ohm to be connected in series with the wire
Example 09:
A potentiometer wire of length 8 m has a resistance of 8 ohm. A resistance box is connected in series with it. An accumulator of e.m.f. 2 volt so as to get a potential drop of 1µ V mm-1. What resistance must be the value of the resistance in the resistance box?
Given: Length of potentiometer wire = lAB = 5 m, Resistance of potentiometer wire = RAB = 5 ohm, e.m.f. of cell = E = 4 V, Resistance in series = R ohm, Potential drop = 1µ V mm-1= 10-3 V m-1.
To Find: Resistance R =?
Solution:
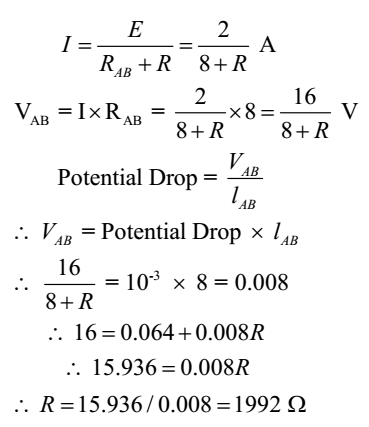
Ans: A resistance of 1992 ohm to be connected in series with the wire
Example 10:
A potentiometer wire of length 4 m has a resistance of 4 ohm. What resistance must be connected in series with this wire and an accumulator of e.m.f. 2 volt so as to get a potential drop of 10-3 V cm-1 on the wire?
Given: Length of potentiometer wire = lAB = 4 m = 400 cm, Resistance of potentiometer wire = RAB = 4 ohm, e.m.f. of cell = E = 2 V, Resistance in series = R ohm, Potential drop = 10-3 V cm-1.
To Find: Resistance R =?
Solution:
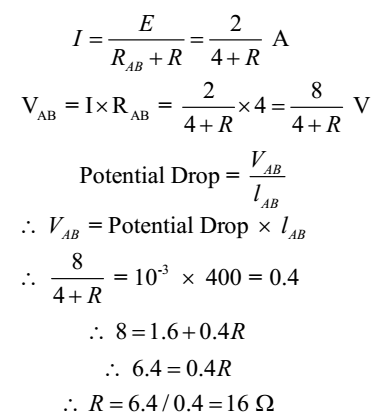
Ans: A resistance of 16 ohm to be connected in series with the wire
Example 11:
A potentiometer wire of length 4 m has a resistance of 4 ohm. What resistance must be connected in series with this wire and an accumulator of e.m.f. 2 volt and internal resistance 2 ohm so as to get a potential drop of 10-3 V cm-1 on the wire?
Given: Length of potentiometer wire = lAB = 4 m = 400 cm, Resistance of potentiometer wire = RAB = 4 ohm, e.m.f. of cell = E = 2 V, Internal resistance = r = 2 ohm, Resistance in series = R ohm, Potential drop = 10-3 V cm-1.
To Find: Resistance R =?
Solution:

Ans: A resistance of 14 ohm to be connected in series with the wire
Example 12:
A potentiometer wire of length 5 m has a resistance of 10 ohm. It is connected in series with a cell of e.m.f. 2 V and resistance R. If a P.D. of 3 mV is balanced against a length of 3 m. Find R.
Given: Length of potentiometer wire = lAB = 5 m, Resistance of potentiometer wire = RAB = 10 ohm, e.m.f. of cell = E = 2 V, Resistance in series = R ohm, Banaced P.D. = 3 mV = 3 x 10-3 V, Balancing length = 3m.
To Find: Resistance R =?
Solution:
Balanced P. D. = Potential drop x Balancing length
3 x 10-3 = Potential drop x 3
Potential drop = 3 x 10-3 / 3 = 10-3 Vm-1

Ans: A resistance of 3990 ohm to be connected in series with the wire
Example 13:
A potentiometer wire of length 10 m has a resistance of 10 ohm. It is connected in series with a cell of e.m.f. 4 V and resistance R. If a source of 100 mV is balanced against a length of 4 m of potentiometer wire. Find R.
Given: Length of potentiometer wire = lAB = 10 m, Resistance of potentiometer wire = RAB = 10 ohm, e.m.f. of cell = E = 4 V, Resistance in series = R ohm, Balanced e.m.f. = 100 mV = 100 x 10-3 V = 0.1 V, Balancing length = 4m.
To Find: Resistance R =?
Solution:
Balanced P. D. = Potential drop x Balancing length
0.1 = Potential drop x 4
Potential drop = 0.1 / 4 = 0.025Vm-1

Ans: A resistance of 150 ohm to be connected in series with the wire
Previous Topic: Theory of Potentiometer
Next Topic: Numerical Problems on to Find Internal Resistance of a cell Using Potentiometer