Refraction Through Prism

Science > Physics > Refraction of Light > Refraction Through Prism

In this article, we shall study refraction through prism.

Prism:

A prism is a portion of a transparent medium which is bounded by non-parallel plane surfaces. The two plane surfaces ABCD and AEFD intersecting each other in line AD are called the refracting faces of the prism.  The angle between the two refracting faces ABCD and AECD is called the refracting angle of the prism.  The face BEFC is called the base of the prism.

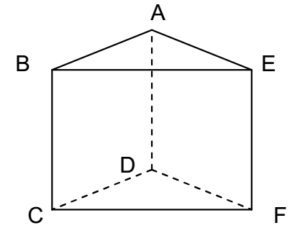
Terminology of Prism:

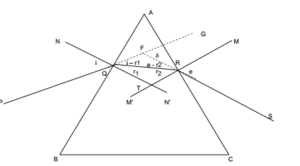
AB & AC	Retracting surfaces of prism	NN’ and MM’	Normals
BC	Base of prism	r1 and r2	Angle of refractions
A	Refracting angle of prism	R	Point of emergence
Ray PQ	Incident ray	Ray RS	Emergent ray
Point Q	Point of Incidence	e	Angle of emergence
i	Angle of Incidence	δ	Angle of deviation

Prism Formula:

From figure, ∠ NQP = i and ∠  RQT = r₁

∴   ∠  FQR = i – r₁

From figure, ∠ MRS = e and ∠ QRT = r₂

∴  ∠ FRQ = e – r₂

Now ∠ GFR is external angle of ΔFQR by property at external angle

∠ GFR = ∠ FRG + ∠ FQR

∴  ∠ GFR = e – r₂ + i – r₁

∴  ∠  GFR = i + e – r₁ – r₂

∴   ∠ GFR = i + e – (r₁ + r₂)

∴   δ = I + e – (r₁ + r₂ ) ………………(1)

Consider ΔQTR

∴  ∠ RQT + ∠  QTR + ∠ TRQ = 180°

∴   r₁ + ∠ QTR+ r₂ = 180°

∴   ∠ QTR = 180° – r₁ – r₂

∴   ∠ QTR = 180° – (r₁ + r₂) …………….(2)

□ AQRT is cyclic quadrilateral, by property of cyclic quadrilateral

∴   ∠ QAR + ∠ QTR = 180°

∴   A + ∠ QTR = 180°

∴   ∠ QTR = 180° – A …………… (3)

From (2) & (3)

∴  180° – A = 180°  – (r₁ + r₂)

A =  –  (r₁ + r₂)

∴  A =  r₁  +  r₂  …………..(4)

from (1) and (4)

= i + e – A ……………(5)

As angle of incidence increases angle of deviation (ð) decreases up to certain minimum value ð_m called angle of minimum deviation then it starts increasing

At minimum deviation ð =  ð_m and i = e.

Substituting these values in equation (5)

ð_m = i + i – A

∴ ð_m + A = 2 i

∴ i = (A + ð_m) /2   ………….(6)

similarly at minimum deviation, r₁ = r₂ = r

substituting this value in equation (4)

r₁ + r₂ = A

r + r = A

2r = A

∴ r =  A/2  ………(7)

by Snell’s Law

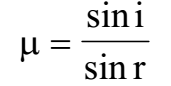
where μ = refractive index of the material of the prism

This formula is known as prism formula.

Terminology:

Deviation:

When a ray of light passes from one medium to another it changes its path, this phenomenon of light is called the deviation of light. When a ray of light passes through a prism it suffers refraction twice. Once while it enters the prism and again while it emerges from it.

When light rays pass from optically rarer medium to optically denser medium, the light rays bend towards the normal. When light rays pass from optically denser medium to optically rarer medium, the light rays bend away from the normal.

Angle of deviation:

The angle between the direction of the incident ray and the direction of the emergent ray is called the angle of deviation.

Angle of Minimum Deviation:

For a given prism the value of the angle deviation depends on the value of the angle of incidence. As the angle of incidence is increase the angle of deviation steadily decreases up to a certain minimum value of the angle of deviation. This minimum value of the angle of deviation is called angle of minimum deviation.  It can be shown graphically as follows.

For a particular angle of incidence, the angle of deviation is the least. This least angle of deviation is called the angle of minimum deviation.

At minimum deviation angle of incidence and angle of emergence are equal i.e. i = e. Similarly, the angle of refraction are equal i.e. r₁ = r₂

The angle of minimum deviation as produced by a prism depends upon (i) the refracting angle of the prism and (ii) Refractive index of the material of the prism.

Thin Prism:

The prism whose refracting angle is less than 5° is called thin prism.

By prism formula we have

∴   μ A = A + δ

∴  δ = μ A – A

∴  δ = A(μ  – 1)

This is an expression for the angle of deviation in case of a thin prism.

Science > Physics > Refraction of Light > Refraction Through Prism