Concept of Strain Energy

Science > Physics > Elasticity > Concept of Strain Energy

In this article, we shall study, work done in stretching wire and the concept of strain energy.

Work done in Stretching a Wire:

Consider a wire of length ‘L’ and area of cross-section ‘A’ be fixed at one end and stretched by suspending a load ‘M’ from the other end. The extension in the wire takes place so slowly that it can be treated as quasi-static change; because internal elastic force in the wire is balanced by the external applied force and hence acceleration is zero.

Let at some instant during stretching the internal elastic force be ‘f’ and the extension produced be ‘x’. Then,

Since at any instant, the external applied force is equal and opposite to the internal elastic force, we can say that the work done by the external applied force in producing a further infinitesimal dx is

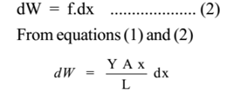
Let ‘ l ‘ be the total extension produced in the wire, and work done during the total extension can be found by integrating the above equation.

This is an expression for the work done in stretching wire.

Strain Energy:

The work done by the external applied force during stretching is stored as potential energy (U) in the wire and is called as strain energy in the wire. Thus the strain energy is given by

Its S.I. unit is J (joule) and its dimensions are [L²M¹T ^-2].

Strain Energy Per Unit Volume of a Wire:

The work done by external applied force during stretching is stored as potential energy (U) in the wire and is called as strain energy in the wire. Dividing both sides above equation by AL, the volume of the wire.

This is an expression for strain energy or potential energy per unit volume of stretched wire.  This is also called as the energy density of the strained wire.  Its S.I. unit is J m^-3 and its dimensions are [L^-1M¹T ^-2].

Different Forms of Expression of Strain Energy per Unit Volume:

By definition of Young’s modulus of elasticity

Now. Young’s modulus of elasticity for a material of a wire is constant.

Thus, strain energy per unit volume ∝ (stress)² i.e. strain energy per unit volume is directly proportional to the square of the stress.

Note:

More work is to be done for stretching a steel wire than stretching a copper wire because steel is more elastic than copper. Due to which more restoring force is produced in the steel, hence we have to do more work to overcome these larger restoring forces.

Numerical Problems:

Example – 1:

Find the work done in stretching a wire of length 2 m and of sectional area 1 mm² through 1 mm if Young’s modulus of the material of the wire is 2  × 10¹¹ N/m².

Given: Area = A = 1 mm² = 1 × 10^-6 m², Length of wire = L = 2m, Extension in wire = l = 1mm = 1 × 10^-3 m, Young’s modulus = Y =2 × 10¹¹ N/m².

To Find: Work done = W =?

Solution:

Young’s modulus of elasticity = Y = FL/Al

∴ F = YAl/L

∴   F = (2 × 10¹¹ × 1 × 10^-6 × 1 × 10^-3)/2

∴   F = 100 N

Now Work done in stretching wire = ½ Load × Extension

∴ Work done = ½ × 100 × 1 × 10^-3

∴ Work done = 0.05 J

Ans: Work done in stretching wire is 0.05 J

Example – 2:

Calculate the work done in stretching a wire of length 3 m and cross-sectional area 4 mm² when it is suspended from rigid support at one end and a load of 8 kg is attached at the free end. Y = 12 × 10¹⁰ N/m² and g = 9.8 m/s².

Given: Area = A = 4 mm² = 4 × 10^-6 m², Length of wire = L = 3m, Load = 8 kg-wt = 8 × 9.8 N, Young’s modulus = Y = 12 × 10¹⁰ N/m².

To Find: Work done = W =?

Solution:

Young’s modulus of elasticity = Y = FL/Al

∴ l = FL/AY

∴ l = (8 × 9.8 × 3) / (4 × 10^-6 × 12 × 10¹⁰)

∴ l = 4.9 × 10^-4 m

Now Work done in stretching wire = ½ Load ×Extension

∴ Work done = ½ × 8 × 9.8 × 4.9 × 10^-4

∴ Work done = 1.921 × 10^-2 J = 0.0192 J

Ans: Work done in stretching wire is 0.0192 J

Example – 3:

When the load on a wire is increased slowly from 3 to 5 kg wt, the elongation increases from 0.6 to 1 mm. How much work is done during the extension? g = 9.8 m/s².

Given: Initial Load = F₁ = 3 kg wt = 3 × 9.8 N, Final load =F₂ = 5 kg-wt = 5 × 9.8 N, Initial extension l₁ = 0. 6 mm = 0.6  × 10^-3  m = 6  × 10^-4  m, Final extension = l₂ = 1mm = 1  × 10^-3  m = 10  × 10^-4  m, g = 9.8 m/s² .

To Find: Work done = W =?

Solution:

Work done = W = W2 – W1

∴ Work done = ½ × F₂× l₂ – ½ × F₁ × l₁

∴ Work done = ½ × (F₂ × l₂ – F₁ × l₁)

∴ Work done = ½ × (5 × 9.8 × 10 × 10^-4 – 3 × 9.8 × 6 × 10^-4)

∴ Work done = ½ × 9.8 × 10^-4(50 – 18)

∴ Work done = ½ × 9.8 × 10^-4 × 32

∴ Work done =1.568 × 10^-2 = 0.01568 J

Ans: Work done is 0.01568 J

Example – 4:

A spring is compressed by 1 cm by a force of 3.92 N. What force is required to compress it by 5 cm? What is the work done in this case? Assume the Hooke’s Law.

Given: Initial Load = F₁ = 3.92 N, Initial extension l₁ = 1 cm = 1 × 10^-2 m, Final extension = l₂ = 5 cm = 5  × 10^-2  m.

To Find: Final Load = F₂ =? Work done = W =?

Solution:

We have Force constant = K = F/l

Hence F₁/l₁ = F₂/l₂

Hence F₂   = (F₁ × l₂)/ l₁ = (3.92× 5 × 10^-2) /(1  × 10^-2)

∴ F₂ = (3.92× 5 × 10^-2) / (1 × 10^-2)

Ans: (9.8 N; 0.49)

Example – 5:

A wire 4m long and 0.3 mm in diameter is stretched by a load of 0.8 kg. If the extension caused in the wire is 1.5 mm, find the strain energy per unit volume of the wire.g = 9.8 m/s²

Given: Length of wire = L = 4m, Diameter = 0.3 mm, Radius of wire = r = 0.3/2 = 0.15 mm = 015 × 10^-3 m = 1.5 × 10^-4 m, Area = Load applied = F = 0.8 kg-wt = 0.8 × 9.8 N, Extension in wire = l = 1.5 mm = 1.5 × 10^-3 m, .g = 9.8 m/s².

To Find: Strain energy per unit volume = dU/V =?

Solution:

Strain energy per unit volume =½ × Stress × Strain

∴ dU/V =½ × (F/A) × (l/L)

∴ dU/V =½ × (Fl/AL)

∴ dU/V =½ × (Fl/πr²L)

∴ dU/V =½ × (0.8 × 9.8 × 1.5 × 10^-3) / (3.142 × (1.5 × 10^-4)² × 4)

∴ dU/V =½ × (0.8 × 9.8 × 1.5 × 10^-3) / (3.142 × 2.25 × 10^-8 × 4)

∴ dU/V = 2.08 × 10⁴   J/m³

Ans : The strain energy per unit volume of the wire  2.08 × 10⁴   J/m³

Example – 6:

Find the energy stored in a stretched brass wire of 1 mm² cross-section and of an unstretched length 1 m when loaded by 2 kg wt. What happens to this energy when the load is removed? Y = 10¹¹ N/m².

Given: Area = A = 1 mm² = 1 × 10^-6 m², Length of wire = L = 1 m, Load = 2 kg-wt = 2 × 9.8 N, Young’s modulus  = Y  = 10¹¹  N/m².

To Find: Energy stored = dU =?

Solution:

Young’s modulus of elasticity = Y = FL/Al

∴ l = FL/AY

∴ l = (2 × 9.8 × 1) / (1 × 10^-6 × 10¹¹)

∴ l = 1.96 × 10^-4 m

Now Work done in stretching wire = ½ Load ×Extension

∴ Work done = ½ × 2 × 9.8 × 1.96 × 10^-4

∴ Work done = 1.921 × 10^-3 J

Now energy stored = Work done in stretching wire

Ans:  Energy stored is 1.921 × 10^-3 J

Example – 7:

A metal wire of length 2.5 m and are of cross section 1.5 × 10^-6 m² is stretched through 2 mm. Calculate work done during stretching. Y = 1.25 × 10¹¹ N/m².

Given: Area = A = 1.5 × 10^-6 m², Length of wire = L = 2.5 m, Extension = l = 2mm = 2 × 10^-3 m, Young’s modulus = Y  = 1.25 × 10¹¹ N/m².

To Find: Energy stored = dU =?

Solution:

Young’s modulus of elasticity = Y = FL/Al

∴ F = YAl/L

∴ F = (1.25 × 10¹¹ × 1.5 × 10^-6 × 2 × 10^-3)/2.5

∴ F = 150 N

Now Work done in stretching wire = ½ Load ×Extension

∴ Work done = ½ × 150 × 2 × 10^-3

∴ Work done = 0.150 J

Now energy stored = Work done in stretching wire

Ans:  Energy stored is 0.150 J

Example – 8:

A copper wire is stretched by 0.5% of its length. Calculate the energy stored per unit volume in the wire. Y = 1.2 × 10¹¹ N/m².

Given: Strain = l/L = 0.5 % = 0.5 × 10^-2 = 5 × 10^-3, Young’s modulus = Y  = 1.2 × 10¹¹ N/m².

To Find: Strain energy per unit volume = dU/V =?

Solution:

Strain energy per unit volume = dU/V = ½ × (Strain)² × Y

∴   dU/V = ½ × (5 × 10^-3)² × 1.2 × 10¹¹

∴   dU/V = ½ × 25 × 10^-6 × 1.2 × 10¹¹

∴   dU/V = 1.5 × 10⁶    J/m³

Ans: The strain energy per unit volume of the wire 1.5 × 10⁶    J/m³

Example – 9:

Calculate the strain energy per unit volume in a brass wire of length 2.0 m and cross-sectional area 0.5 mm2, when it is stretched by 2mm and a force of 5 kg-wt is applied to its free end.

Given: Area = A = 0.5 mm² = 0.5 × 10^-6 m² = 5 × 10^-7 m², Length of wire = L = 2.0 m, Extension in wire = l = 2 mm = 2 × 10^-3 m, Load applied = F = 5 kg-wt = 5 × 9.8 N

To Find: Strain energy per unit volume = dU/V =?

Solution:

Strain energy per unit volume = dU/V = ½ × Stress × Strain

∴    Strain energy per unit volume = ½ × (F/A) × (l/L)

∴    Strain energy per unit volume = ½ × (Fl/AL)

∴    Strain energy per unit volume = ½ × (5 × 9.8 × 2 × 10^-3) / (5 × 10^-7 × 2)

∴    Strain energy per unit volume = 4.9 × 10⁴ J/m³

Ans: The strain energy per unit volume of the wire 4.9 × 10⁴ J/m³

Example – 10:

Calculate the work done in stretching a wire of length 2 m and cross-sectional area 0.0225 mm² when a load of 100 N is applied slowly to its free end. Young’s modulus of elasticity = 20 × 10¹⁰ N/m².

Solution:

Given: Area = A =0.0225 mm² =0.0225 × 10^-6 m² = 2.25 × 10^-8 m², Length of wire = L = 2 m, Load applied = F = 100 N, Young’s modulus of elasticity = Y = 20 × 10¹⁰ N/m².

To Find: Work done = W =?

Young’s modulus of elasticity = Y = FL/Al

∴ l = FL/AY

∴ l = (100 × 2) / (2.25 × 10^-8 × 20 × 10¹⁰)

∴ l = 4.444 × 10^-2 m

Now Work done in stretching wire = ½ Load ×Extension

∴ Work done = ½ × 100 × 4.444 × 10^-2

∴ Work done = 2.222 J

Ans: Work done in stretching wire is 2.222 J

Example – 11:

A uniform steel wire of length 3 m and area of cross-section 2 mm² is extended through 3mm. Calculate the energy stored in the wire, if the elastic limit is not exceeded. Young’s modulus of elasticity = Y = 20 × 10¹⁰

Given: Area = A =2 mm² =2 × 10^-6 m², Length of wire = L = 3 m, Extension = l = 3 mm = 3 × 10^-3 m, Young’s modulus of elasticity = Y = 20 × 10¹⁰ N/m².

To Find: Energy stored = dU =?

Solution:

Young’s modulus of elasticity = Y = FL/Al

∴ F = YAl/L

∴   F = (20 × 10¹⁰ × 2 × 10^-6 × 3 × 10^-3)/3

∴   F = 400 N

Now Work done in stretching wire = ½ Load ×Extension

∴ Work done = ½ × 400 × 3 × 10^-3

∴ Work done = 0.6 J

Energy stored = work done in stretching wire = 0.6 J

Ans: Energy stored is 0.6 J

Previous Topic: Shear Stress, Shear Starin, and Modulus of Rigidity

For More Topics in Physics Click Here

Science > Physics > Elasticity > Concept of Strain Energy