Science > Physics > Interference of Light > Numerical Problems on Fringe Width
In this article, we shall study numerical problems based on Young’s experiment and biprism experiment to find the fringe width of the interference pattern and to find the wavelength of light used.
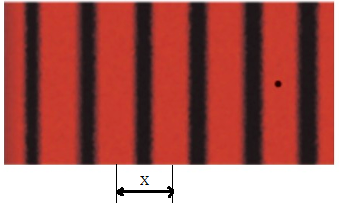
Example – 01:
In Young’s experiment, the distance between the two slits is 0.8 mm and the distance of the screen from the slits is 1.2m. If the fringe width is 0.75 mm, calculate the wavelength of light.
Given: Distance between slits = d = 0.8 mm = 0.8 x 10-3 m = 8 x 10-4 m. Distance between slit and screen = D = 1.2 m, Fringe width = X = 0.75 mm = 0.75 x 10-3 m = 7.5 x 10-4 m.
To Find: Wavelength of light used = λ =?
Solution:
The fringe width is given by X = λD/d
∴ λ = Xd/D = (7.5 x 10-4 x 8 x 10-4)/1.2 = 5 x 10-7 m = 5000 x 10-10 m = 5000 Å
Ans: Wavelength of light used is 5000 Å
Example – 02:
In Young’s experiment, the distance between the two images of the sources is 0.6 mm and the distance between the source and the screen is 1.5 m. Given that the overall separation between 20 fringes on the screen is 3 cm, calculate the wavelength of light used.
Given: Distance between images = d = 0.6 mm = 0.6 x 10-3 m = 6 x 10-4 m. Distance between source and screen = D = 1.5 m, Fringe width = X = (3/20) cm = 0.15 cm = 0.15 x 10-2 m = 1.5 x 10-3 m.
To Find: Wavelength of light used = λ =?
Solution:
The fringe width is given by X = λD/d
∴ λ = Xd/D = (1.5 x 10-4 x 6 x 10-3)/1.5 = 6 x 10-7 m = 6000 x 10-10 m = 6000 Å
Ans: Wavelength of light used is 6000 Å
Example – 03:
The distance between two consecutive bright bands in Young’s experiment is 0.32 mm when the red light of wavelength 6400 Å is used. By how much will this distance change if the light is substituted by the blue light of wavelength 4800 Å with the same setting?
Given: For red light: fringe width = Xr = 0.32 mm, wavelength = λr = 6400 Å, For blue light: wavelength = λb = 4800 Å
To Find: Change in fringe width = ∆X =?
Solution:
The fringe width is given by X = λD/d
For red light Xr = λrD/d ………….. (1)
For blue light, Xb = λbD/d ………….. (2)
Dividing Equation (2) by (1)
Xb/ Xr = (λbD/d) x (d/ λrD)
∴ Xb/ Xr = (λb/λr)
∴ Xb = (λb/λr) x Xr = (4800/6400) x 0.32 = 0.24 mm
∴ ∆X = Xb – Xr = 0. 32 – 0.24 = 0.08 mm
Ans: The distance between two consecutive bright bands will change by 0.08 mm
Example – 04:
Calculate the fringe width in the pattern produced in a biprism experiment given that the wavelength of light employed is 6000 Å, distance between sources is 1.2 mm and distance between the source and the screen is 100 cm. what will be the change in fringe width if the entire apparatus is immersed in water for which refractive index is 4/3.
Part – I:
Given: Distance between sorces = d = 1.2 mm = 1.2 x 10-3 m, Distance between sources and screen = D = 100 cm = 1 m, Wavelength of light = λ = 6000 Å = 6000 x 10-10 m = 6 x 10-7 m
To Find: Fringe width = X =?
X = λD/d = (6 x 10-7 x 1) / (1.2 x 10-3) = 5 x 10-4 m = 0.5 mm
Part – II:
Given: Fringe width in air = X1 = 0.5 mm, wavelength = λ1 = 6000 Å, for the second medium refractive index = μ = 4/3
To Find: Change in fringe width = ∆X =?
Solution:
μ = 4/3 =λ1 / λ2
The fringe width is given by X = λD/d
For first medium X1 = λ1D/d ………….. (1)
For second medium, X2 = λ2D/d ………….. (2)
Dividing Equation (1) by (2)
X1/ X2 = (λ1D/d) x (d/ λ2D)
∴ X1/ X2 = (λ1/λ2) = 4/3
∴ X2 = (3/4) X1 = (3/4) x 0.5 = 0.375 mm
∴ ∆X = X1 – X2r = 0. 5 – 0.375 = 0.125 mm
Ans: The initial fringe width is 0.5 mm and the fringe width changes by 0.125 mm
Example – 05:
In a Young’s experiment, a source of light having wavelength 6500 Å is replaced by a source of light of wavelength 5500 Å. Find the change in fringe width if the screen is at a distance of 1 m from the two sources which are 1 mm apart.
Part – I:
Given: Distance between sorces = d = 1 mm = 1 x 10-3 m, Distance between sources and screen = D = 100 cm = 1 m, Initial wavelength of light = λ1 = 6500 Å = 6500 x 10-10 m = 6.5 x 10-7 m, final wavelength of light = λ2 = 5500 Å = 5500 x 10-10 m = 5.5 x 10-7 m,
To Find: Change in fringe width = ∆X =?
The fringe width is given by X = λD/d
For first medium X1 = λ1D/d ………….. (1)
For second medium, X2 = λ2D/d ………….. (2)
∴ ∆X = X1 – X2 = λ1D/d – λ2D/d = (D/d)( λ1 – λ2) = (1/1 x 10-3)(6.5 x 10-7 – 5.5 x 10-7)
∴ ∆X = X1 – X2 = λ1D/d – λ2D/d = (D/d)( λ1 – λ2) = 103 x (6.5 – 6.5) x 10-7 = 1 x 10-4 m = 0.1 mm
Ans: The fringe width changes by 0.1 mm
Example – 06:
In a biprism experiment, the width of a fringe is 0.75 mm when the eye-piece is at a distance of one metre from the slits. The eye-piece is now moved away from the slits by 50 cm. Find the fringe width.
Given: For first case: fringe width = X1 = 0.75 mm, distance of eye piece from the slits = D1 = 1 m, For second case: distance of eye piece from the slits = D2 = 1 m + 50 cm = 1m + 0.5 m = 1.5 m
To Find: New fringe width =?
Solution:
The fringe width is given by X = λD/d
For first case X1 = λD1/d ………….. (1)
For second case, X2 = λD2/d ………….. (2)
Dividing equation (2) by (1)
X2 / X1 = (λD2/d) x (d/ λD1)
∴ X2 / X1 = D2/D1
∴ X2 = (D2/D1) x X1
∴ X2 = (1.5/1) x 0.75 = 1.125 mm
Ans: New fringe width is 1.125 mm
Example – 07:
In Young’s experiment the distance between the slits is 1 mm and fringe width is 0.60 mm when light of certain wavelength is used. When the screen is moved through 0.25 m the fringe width increases by 0.15 mm. What is the wavelength of light used?
Given: For first case: fringe width = X1 = 0.60 mm = 0.60 x 10-3 m, distance between the slits = d = 1mm = 1 x 10-3 m, Distance of screen from slit D1 = D m. For second case: fringe width = X2 = 0.6 + 0.15 = 0.75 mm, as the fringe width is increasing the screen is moved away from the slits, distance of the screen from the slits = D2 = D m + 0.25 m = (D + 0.25) m
To Find: New fringe width =?
Solution:
The fringe width is given by X = λD/d
For first case X1 = λD1/d ………….. (1)
For second case, X2 = λD2/d ………….. (2)
Dividing equation (2) by (1)
X2 / X1 = (λD2/d) x (d/ λD1)
∴ X2 / X1 = D2/D1
∴ 0.75 / 0.60= (D + 0.25)/D
∴ 5/4= (D + 0.25)/D
∴ 5D = 4D + 1
∴ D = 1 m
Now, X1 = λD1/d
∴ λ =(X1d) /D = (0.60 x 10-3 x 1 x 10-3) /1 = 6 x 10-7 m
∴ λ = 6000 x 10-10 m = 6000 Å
Ans: Wavelength of light used is 6000 Å
Example – 08:
In a Young’s experiment, the width of a fringe is 0.12 mm when the screen is at a distance of 100 cm from the slits. The screen is now moved towards the slit by 25 cm. Find the fringe width.
Given: For first case: fringe width = X1 = 0.12 mm, distance of eye piece from the slits = D1 = 100 cm = 1 m, For second case: distance of eye piece from the slits = D2 = 1 m – 25 cm = 1m – 0.25 m = 0.75 m
To Find: New fringe width =?
Solution:
The fringe width is given by X = λD/d
For first case X1 = λD1/d ………….. (1)
For second case, X2 = λD2/d ………….. (2)
Dividing equation (2) by (1)
X2 / X1 = (λD2/d) x (d/ λD1)
∴ X2 / X1 = D2/D1
∴ X2 = (D2/D1) x X1
∴ X2 = (0.75/1) x 0.12 = 0.09 mm
Ans: New fringe width is 0.09 mm
Example – 09:
In Young’s experiment, interference bands are produced on the screen placed 1.5 m from two slits 0.15 mm apart and illuminated by the light of wavelength 6000 Å Find (1) fringe width (2) change in fringe width if the screen is taken away from the slits by 50 cm.
Solution:
Part – I:
Given: Distance between slits = d = 0.15 mm = 0.15 x 10-3 m = 1.5 x 10-4 m. Distance between slit and screen = D = 1.5 m, Wavelength of light = λ = 6000 Å = 6000 x 10-10 m = 6 x 10-7 m
To Find: Fringe width = X =?
X = λD/d = (6 x 10-7 x 1.5) / ( 1.5 x 10-4) = 6 x 10-3 m = 6 mm
Part – II:
Given: For first case: fringe width = X1 = 6 mm, distance of eye piece from the slits = D1 = 1.5 m, for second case: distance of eye piece from the slits = D1 = 1.5 m + 50 cm = 1.5 m + 0.5 m = 2 m
To Find: Change fringe width = ∆X =?
The fringe width is given by X = λD/d
For first case X1 = λD1/d ………….. (1)
For second case, X2 = λD2/d ………….. (2)
Dividing equation (2) by (1)
X2 / X1 = (λD2/d) x (d/ λD1)
∴ X2 / X1 = D2/D1
∴ X2 = (D2/D1) x X1
∴ X2 = (2/1.5) x 6 = 8 mm
∴ Change in fringe width = ∆X = X2 – X1 = 8 – 6 = 2mm
Ans: Initial fringe width is 6 mm and the change in fringe width is 2 mm
Example – 10:
In Young’s experiment, interference bands are produced on the screen placed 1.5 m from two slits 1.5 mm apart and illuminated by the light of wavelength 4500 Å Find (1) fringe width (2) change in fringe width if the screen is moved towards the slits by 50 cm.
Solution:
Part – I:
Given: Distance between slits = d = 1.5 mm = 1.5 x 10-3 m = 1.5 x 10-4 m. Distance between slit and screen = D = 1.5 m, Wavelength of light = λ = 4500 Å = 4500 x 10-10 m = 4.5 x 10-7 m
To Find: Fringe width = X =?
X = λD/d = (4.5 x 10-7 x 1.5) / (1.5 x 10-3) = 4.5 x 10-4 m = 0.45 mm
Part – II:
Given: For first case: fringe width = X1 = 0.45 mm, distance of eye piece from the slits = D1 = 1.5 m, For second case: distance of eye piece from the slits = D1 = 1.5 m – 50 cm = 1.5 m – 0.5 m = 1 m
To Find: Change fringe width = ∆X =?
The fringe width is given by X = λD/d
For first case X1 = λD1/d ………….. (1)
For second case, X2 = λD2/d ………….. (2)
Dividing equation (2) by (1)
X2 / X1 = (λD2/d) x (d/ λD1)
∴ X2 / X1 = D2/D1
∴ X2 = (D2/D1) x X1
∴ X2 = (1/1.5) x 0.45 = 0.30 mm
∴ Change in fringe width = ∆X = X2 – X1 = 0.45 – 0.30 = 0.15 mm
Ans: Initial fringe width is 0.45 mm and the change in fringe width is 0.15 mm
Example – 11:
In Young’s experiment, the fringe width is 0.65 mm when the screen is at a distance of 1.5 m from the slits. What will be the fringe width if the screen is moved towards the slits by 50 cm.
Solution:
Given: For first case: fringe width = X1 = 0.65 mm, distance of eye piece from the slits = D1 = 1.5 m, For second case: , distance of eye piece from the slits = D1 = 1.5 m – 50 cm = 1.5 m – 0.5 m = 1 m
To Find: fringe width = X2 =?
The fringe width is given by X = λD/d
For first case X1 = λD1/d ………….. (1)
For second case, X2 = λD2/d ………….. (2)
Dividing equation (2) by (1)
X2 / X1 = (λD2/d) x (d/ λD1)
∴ X2 / X1 = D2/D1
∴ X2 = (D2/D1) x X1
∴ X2 = (1/1.5) x 0.65 = 0.43 mm
Ans: New fringe width is 0.43 mm
Previous Topic: Concept of Fringe Width and Path Difference
Next Topic: More Problems on Fringe Wdth and Change of Fringe Width
Next Topic: