Science > Chemistry > Molecule and Molecular Mass > Molecular Mass by Molar Volume Method
In this article, we shall study the concept of molecular mass (molar mass) and molar volume method to determine it.
Molecule:
When two or more atoms are firmly held together by a chemical bond, a molecule is formed. The molecule of an element may consist of one or more atoms of the same kind, while that of the chemical compound consists of different kinds of atoms.
The smallest particle of an element or compound which can exist in a free state and does not take part in a chemical reaction is called molecule.
Molecules are denoted by formula indicating the number of constituent elements in the compound. For example molecular formula for oxygen is O2. Thus one molecule oxygen consists of two atoms of oxygen
Molecular Mass or Molar Mass:
The molecular mass or molar mass of a substance is defined as the ratio of the mass of one molecule of a substance to 1/12 th of the mass of 6C12 isotope taken as 12000 units.
Gram Molecular Mass or Molar Mass:
The molecular mass expressed in grams is called gram molecular mass (GMM)
Method – I (Molar Volume Method)
Principle:
In this method we find a known volume of a gas at S.T.P. and using the concept that one mole of every gas occupies 22.4 dm3 by volume, we calculate the molar mass of the gas.
Procedure:
- Find the volume of the gas at STP, from given data.
- Find molecular mass, using the formula
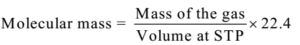
Numerical Problems:
Example – 01:
10 dm3 of gas at 14 °C and 729 mm pressure has a mass of 17.925 g. Calculate the relative molecular mass of the gas.
Given: V = 10 dm3, t = 14 °C, T = 14 + 273 = 287 K, P = 729 mm of Hg , W = 17.925 g
Solution:
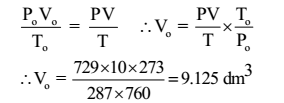
Thus the mass of 9.125 dm3 of gas is 17.925 g. Hence the mass of 22.4 dm3 of a gas
Molecular mass = (17.975/9.125) x 22.4 = 44
Ans: The relative molecular mass of the gas is 44 g
Example – 02:
5 litres of a gas at NTP has a mass of 14.4 g. Find the relative molecular mass of the gas.
Given: Vo = 5 litres = 5 dm3, W = 14.4 g
Solution:
The mass of 5 dm3 of a gas at NTP is 14.4 g. Hence mass of 22.4 dm3 of a gas
Molecular mass = (14.4/5) x 22.4 = 64.51
Ans: The molecular mass of the gas is 64.51 g.
Example – 03:
240 ml of a dry gas measured at 300 K and 750 mm of mercury has a mass of 0.42336 g. calculate the relative molecular mass of the gas.
Given: V = 240 ml = 0.240 dm3, T = 300 K, P = 750 mm of Hg , W = 0.42336 g
Solution:
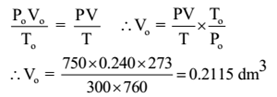
The mass of 0.2155 dm3 of gas is 0.42336 g. Hence mass of 22.4 dm3 of a gas
Molecular mass = (0.42336/0.2115) x 22.4 = 44
Ans: The molecular mass of the gas is 44 g.
Example – 04:
1.25 g of a pure carbonate on ignition leave a residue of 0.70 g and evolved is 312 mm at 27 °C and 755 mm of mercury. Calculate the molecular mass of the gas.
Given: Mass of carbonate = 1.25 g, Mass of residue = 0.70 g, Mass of the gas = 1.25 – 0.70 = 0.55 g, V = 312 ml = 0.312 dm3 T = 27 °C = 27 + 273 = 300 K, P = 755 mm of Hg , W = 0.55 g
Solution:

The mass of 0.2821 dm3 of gas is 0.55 g. Hence the mass of 22.4 dm3 of a gas
Molecular mass = (0.55/0.2821) x 22.4 = 43.67
Ans: The molecular mass of the gas is 43.67 g.