Science > Physics > Electrostatics > Gauss’s Theorem and its Applications
In this article, we shall study the gauss’s theorem and its applications to find electric intensity at a point outside charged bodies of different shapes.
Gauss’s Theorem:
Statement:
Total Normal Electric Induction over any closed surface of any shape in an electric field is equal to the algebraic sum of electric charges enclosed by that surface.
Explanation:
Consider a closed surface enclosing number of charges such as +q1, + q2, – q3 …… then
(TNEI)S = q1 + q2 – q3 + ……= ∑ q.
Proof:
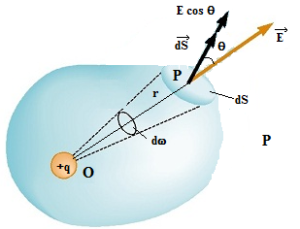
Consider a charge +q situated at a point ‘O’ inside a closed surface of any shape. Such a surface is called a Gaussian surface. Consider a small element dS on its surface. at a distance of ‘r’ from the charge ‘+q’.
The electric intensity at any point over element dS is given by

The normal drawn to the area dS makes an angle θ with the direction of electric intensity E.
Therefore, the total normal electric induction over area dS is given by

The total Normal Electric Induction over the whole Gaussian surface can be obtained by integrating the above expression.

The same argument is true for any other charge present inside the closed surface. Thus if the closed surface enclosed a number of charges such as +q1, + q2, – q3 …… then
(TNEI)S = q1 + q2 – q3 + ……= ∑ q
Thus Gauss’s theorem is proved.
Electric Intensity at a Point Outside a Charged Sphere: (Application of Gauss’s Theorem):
Consider a conducting sphere of radius R on which a charge +q is deposited. The deposited charge gets distributed uniformly over the surface of the sphere. The lines of induction will be normal to the surface, radially outwards. Consider a point P at a distance r from the centre of the sphere (r > R) at which electric intensity is to be found.

To find the electric intensity at point P, construct an imaginary Gaussian spherical surface of radius ‘r’ through P.
The total Normal electric induction over element dS is given by
(TENI)dS = k E εo cos θ dS
As electric intensity vector and area vector are parallel to each other,
θ = 0° and hence cos θ = cos 0° = 1
∴ (TENI)dS = k E εo dS.
Total Normal Induction over whole Gaussian surface is

This is the expression for an electric intensity at a point outside a charged sphere. This relation is the same as the expression electric intensity at distance ‘r’ due to a point charge q. Hence a charged sphere behaves as if the entire charge is concentrated at its centre.
Electric Intensity at a Point Outside a Charged Sphere in Terms of Surface Charge Density of the Sphere:
The electric intensity at a point outside a charged sphere is given by

The surface area of charged sphere = A = 4πR²
Let σ be the charge per unit area of the sphere (surface charge density)
By definition of surface charge density
σ = q /A = q / 4πR²
q = σ × 4πR²
Substituting for q, in equation (1) we get

Where R = Radius of the sphere
r = distance of the point from the centre of the sphere.
This is the expression for an electric intensity at a point outside a charged sphere in terms of surface charge density.
Electric Intensity at a Point on the Surface of a Charged Sphere:
The expression for an electric intensity at a point outside a charged sphere in terms of surface charge density is

This is the expression for an electric intensity at a point on the surface of a charged sphere.
Expression for an Electric Intensity at Point Outside a Charged Cylinder: (Application of Gauss’s Theorem)
Consider a long uniform cylinder of radius ‘R’ carrying charge +q per unit length. Let ‘P’ be a point at a perpendicular distance ‘r’ from the axis (r > R) at which electric intensity is to be found.

To find the electric intensity at point P, let us consider a coaxial imaginary Gaussian cylindrical surface of radius ‘r’ and height ‘l ‘ passing through the point P.
If we consider an element dS on the flat face of the Gaussian cylinder, for this element the electric intensity vector and the area vector are perpendicular to each other. Hence θ = 90°, cos 90° = 0.
Hence TNEI over flat face = 0
Therefore, the Total Normal Electric Induction over the whole surface is the same as the TNEI over a curved surface. Consider an element dS over the curved surface of the Gaussian cylinder. Over this element,
(TENI)dS = k E εo cos θ dS
As electric intensity vector and area vector are parallel to each other,
θ = 0° and hence cos θ = cos 0° = 1
∴ (TENI)dS = k E εo dS.
Total Normal Induction over whole Gaussian surface is

As ‘q’ is the charge per unit length of the cylinder, the charge enclosed by the Gaussian cylinder is ‘ql’.
Applying Gauss’s theorem,

This is the expression for an electric intensity at a point outside a charged cylinder.
Electric Intensity at a Point Outside a Charged Sphere in Terms of Surface Charge Density of the Cylinder:
The electric intensity at a point outside a charged cylinder is given by

Let σ be the charge per unit area of the cylinder (surface charge density)
Let, q = Charge per unit length of the cylinder
∴ q = Charge per unit area × area of unit length.

R = radius of the cylinder
r = perpendicular distance of the point from the axis.
This is the expression for an electric intensity at a point outside a charged cylinder in terms of surface charge density.
Electric Intensity at a Point on the Surface of a Charged Cylinder:
The expression for an electric intensity at a point outside a charged cylinder in terms of surface charge density is

This is the expression for an electric intensity at a point on the surface of a charged cylinder.
Expression for an Electric Intensity at a Point Near a Charged Conductor: (Application of Gauss’s Theorem)
Consider a uniform charged conductor of any shape. Let σ be the surface charge density or charge per unit area of its surface. Consider a point P very close to the surface of the conductor.

Consider an element dS near point P. Construct an imaginary Gaussian surface of cross-sectional area dS with its axis normal to the surface, partly inside and partly outside the conductor. As the electric Intensity inside a conductor is zero, there is no TNEI through cross-sectional area dS outside the conductor. Therefore TNEI through cross-sectional area dS outside the conductor is
(TENI)dS = k E εo cos θ dS
As electric intensity vector and area vector are parallel to each other,
θ = 0° and hence cos θ = cos 0° = 1
∴ (TENI)dS = k E εo dS. …. (1)
Since is the charge density, the total charge enclosed by Gaussian surface over area dS is dS. Hence by Gauss’s theorem,
(TNEI) ds = σ dS …. (2)
From (1) and (2)
k E εo dS = σ dS
∴ k E εo = σ
∴ E = σ / k εo
This is the expression for an electric intensity at a point on near a charged conductor.
Previous Topic: Normal Electric Induction
Next Topic: Numerical Problems on Electric Intensity Due to Charged Sphere