Ampere’s law

Science > Physics > Magnetic Effect of Electric Current > Ampere’s law

In this article, we shall study the Ampere’s law and its application in finding magnetic induction due to long straight conductor, solenoid and toroid.

Statement:

The line integral of the magnetic field B around any closed path is equal to μ₀ times the net steady current enclosed by this path.

Mathematically,

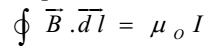
Proof:

The magnetic field produced by a long straight conductor is in the form of concentric circles. These circles are in the plane perpendicular to the length of the conductor. The direction of the field is given by right-hand grip rule.

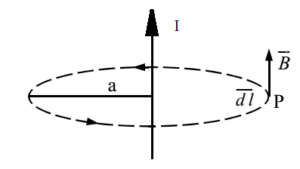
The magnitude of the magnetic field due to a long straight conductor at a point P on the closed-loop of radius ‘a’ is given by

Let us consider a field line through point P and of a very small length ‘dl’ of this field element. Now both B and dl are tangential to the field line at P.

Applications of Ampere’s Law:

Expression for Magnetic Field Due to Solenoid and Toroid:

A cylindrical coil of a large number of turns is called a solenoid. The magnetic field inside the solenoid is given by 

B = μ₀nI.

Where n = number of turns per unit length and

I = Current through the wire of a solenoid

A toroid is a coil which is wound on a torus or a doughnut-shaped structure. For a toroid, 

B = μ₀nI. 

Where n = number of turns per unit circumference and

I = Current through the wire of a toroid

Expression for Magnetic Induction at a Point Due to a Long Straight Conductor Carrying Current:

Let us consider a very long straight conductor carrying electric current ‘I’ as shown. Let us consider a point P at a distance of ‘a’ from the conductor. Let us consider an Amperian loop as an imaginary circle, having radius ‘a’ and passing through point P. Let us consider a very small element of length ‘dl’ of this Amperian loop. Now both  B and dl are tangential to the field line at P.

This is an expression for magnetic induction at a point due to a long straight conductor carrying current.

Expression for Magnetic Induction at a Point  on the Axis of a Long Straight Solenoid and Well Inside it:  

Let us consider a very long straight solenoid having ’n’ turns per unit length and carrying electric current ‘I’ as shown. Let us consider a point well inside the solenoid at which the magnetic induction is to be found.

Consider a rectangular path ABCD of the line of induction such that AB = L = length of the rectangular path. The number of turns enclosed by the rectangle is nL. Hence the total electric current flowing through the rectangular path is nLI. According to  Ampere’s law

Near the ends of the solenoid, the lines of the field are crowded. While for the rest of the space the lines are so widely spaced that the magnetic field is negligible.

This is an expression for magnetic induction at a point on the axis of long straight solenoid and well inside it.

The expression for magnetic induction at a point near and at the end of long straight solenoid is

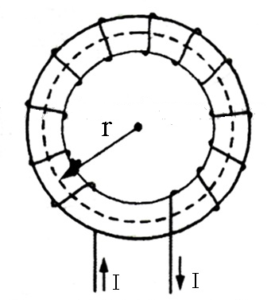
Expression for Magnetic Induction at a Point  on the Axis of a Toroid:

A toroid is a solenoid bent into a shape of a hollow doughnut. Let us consider a toroidal solenoid of average radius ‘r’ having centre O and carrying current ‘I’. Let us consider an Amperian loop of radius r and traverse it in a clockwise direction. Let N be the number of turns of the toroid. Then the total current flowing through the toroid is ‘NI’. According to Ampere’s law

This is an expression for magnetic induction at a point on the axis of a toroid.

Previous Topic: Biot-Savart’s Law and its Applications

Next Topic: Numerical Problems on Toroids

Science > Physics > Magnetic Effect of Electric Current > Ampere’s law