Science > Chemistry > Solutions and Their Colligative Properties > Concentration of Solution
The concentration of a solution is the measure of the composition of a solution. For a given solution, the amount of solute dissolved in a unit volume of solution (or a unit volume of solvent) is called the concentration of the solution. It can be expressed either qualitatively or quantitatively. For example, qualitatively we can say that the solution is dilute (i.e., relatively very small quantity of solute) or it is concentrated (i.e., relatively very large quantity of solute). But in practice, it is not useful hence it is not used in chemistry. The quantitative description method gives an exact concentration of the solution and hence its concentration can be compared with the concentration of other solutions.
Methods of Expressing Concentration of the Solution Quantitatively:
Percentage by Mass or Mass Percentage (w/w):
This method is used for a solid in a liquid solution. The mass of solute in gram dissolved in the solvent to form 100 grams of the solution is called percentage by mass. The ratio of the mass of solute to the mass of the solution is called a mass fraction.
For example, if a solution is described by 10% glucose in water by mass, it means that 10 g of glucose is dissolved in 90 g of water resulting in a 100 g solution.
Concentration described by mass percentage is commonly used in industrial chemical applications. For example, a commercial bleaching solution contains a 3.62 mass percentage of sodium hypochlorite in water.
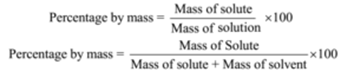
Percentage by Volume (V/V):
This method is used for liquid in a liquid solution. For example, a 10% ethanol solution in water means that 10 mL of ethanol is dissolved in 90 mL water such that the total volume of the solution is 100 mL.
Percentage by Mass by Volume (w/V):
It is the mass of solute dissolved in 100 mL of the solution. This method is commonly used in medicine and pharmacy.
Parts per million:
When a solute is present in trace quantities, it is convenient to express concentration in parts per million (ppm) and is defined as:
As in the case of percentage, concentration in parts per million can also be expressed as mass to mass, volume to volume and mass to volume.
Example: A litre of seawater (which weighs 1030 g) contains about 6 × 10–3 g of dissolved oxygen (O2). Such a small concentration is also expressed as 5.8 g per 106 g (5.8 ppm) of seawater. The concentration of pollutants in water or atmosphere is often expressed in terms of ¼ g mL–1 or ppm.
Strength or Concentration (Grams per litre):
It is defined as the amount of the solute in gram present in the one litre of the solution.
Mole Fraction:
The mole fraction of any component of a solution is defined as the ratio of the number of moles of that component present in the solution to the total number of moles of all components of the solution.
It is to be noted that the sum of the mole fraction of the solute and mole fraction of liquid is 1. The concept of mole fraction is very useful in relating some physical properties of solutions, such as vapour pressure with the concentration of the solution and quite useful in describing the calculations involving gas mixtures. Mole fraction is independent of temperature
Molarity (Molar Concentration):
Molarity (M) is defined as a number of moles of solute dissolved in one litre (or one cubic decimetre) of the solution. The unit of molarity is mol L-1 0r mol dm-3 or M.
Number of moles of a substance can be found using the formula
Molarity changes with temperature because volume changes with temperature.
Molarity can be expressed as
- Decimolar = M/10 (0.1 M)
- Semimolar = M/2 (0.5 M)
- Pentimolar = M/5 (0.2 M)
- Centimolar = M/100 (0.01 M)
- milimolar = M/1000 (0.001 M).
Molality:
Molality (m) is defined as a number of moles of solute expressed in kg dissolved in one kg of solvent, Molality has no unit.
Molality is a better way of expressing concentration than molarity because there is no term of volume of solvent is involved. The volume of the solvent depends on the temperature of the solvent. Thus there is no effect of the change of temperature on the molality.
Molality is related to solubility as
Normality:
Normality (N) is defined as gram-equivalent of solute dissolved in one litre (or one cubic decimetre) of the solution, Unit of molarity is N.
A solution having normality equal to unity is called a normal solution.
Decinormal = N/10 (0.1 N), seminormal = N/2 (0.5 N)
Normality × equivalent mass = strength of solution in g/L.
Formality:
Formality is the number of formula mass in gram present per litre of a solution.
If the formula mass of solute is equal to its molar mass, then the formality is equal to molarity. The formality of a solution depends on temperature. This concept is used in the case of ionic substances.
A mole of an ionic compound is called formole and its molarity is called formality. Thus, the formality of a solution may be defined as a number of moles of ionic solute present in one litre of the solution.
The Relation Between Mole Fraction and Molality:
The mole fraction of any component of a solution is defined as the ratio of the number of moles of that component present in the solution to the total number of moles of all components of the solution.
Let us consider a binary solution components solvent (A) and solute (B).
Let xA = Mole fraction of solvent
xB = Mole fraction of solute
nA = Number of moles of solvent
nB = Number of moles of solute
WA = Mass of solvent
WB = Mass of solute
MA = Molar mass of solvent
MB = Molar mass of solute
Molarity of Dilution:
Molarity of Mixing:
Relation Between Molarity and Molality:
The density of a solution is in g/mL
Relation Between Molarity and Mole Fraction:
Let xA = Mole fraction of solvent
xB = Mole fraction of solute
nA = Number of moles of solvent
nB = Number of moles of solute
M = molarity of solution
d = Density of solution
MA = Molar mass of solvent
MB = Molar mass of solute
Mass of solution = nAMA + nBMB
Volume os solution = Mass of solution/density of solution
Volume os solution = (nAMA + nBMB)/d
The density of a solution is in g/mL
If density is in g/litre then the molarity is given as
Relation Between Normality and Molarity:
The density of a solution is in g/mL and x is the percentage of solute by mass
Previous Topic: Solutions of Solids and Liquids
Next Topic: Numerical Problems on Percentage by Mass
One reply on “Concentration of Solution”
So helpful