Science > Chemistry > Solutions and Their Colligative Properties > Colligative Properties
In this article, we shall learn the meaning of colligative properties, colligative properties of solutions, and the lowering of vapour pressure due to the addition of solute in a solvent.
Colligative Properties:
Colligative properties are those properties of dilute solutions that depend only on the number of solute particles (atoms or molecules, ions or aggregates of molecules) in the solution and not on the nature of solute particles. These properties are important because they are used to determine molar masses of non-electrolyte solutes. Similarly, they are related to one another. If one is measured then other properties can be calculated. These properties can be observed clearly if the solution is very dilute, the solute is non-volatile and the solute does not dissociate or associate in the solution. There are four colligative properties of solutions
- Lowering of vapour pressure.
- Elevation of the boiling point of the solvent in the solution.
- Depression in the freezing point of the solvent in the solution.
- Osmotic pressure
The Concept of Vapour Pressure:
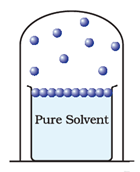
If a container is partially filled with a liquid, a portion of the liquid evaporates to fill the remaining volume of the container with vapour. The molecules of liquid evaporated are in continuous random motion, they collide with the walls of the container and with each other. Thus they create pressure on the walls of the container and on the liquid. At the same time some molecules which have left liquid return back to the liquid, the process is called condensation. After some interval of time, an equilibrium is established between the two phases of substance. At this stage, the rate of evaporation is equal to the rate of condensation.
The pressure exerted by the vapours of the liquid on the surface of the liquid when equilibrium is established between liquid and its vapour is called vapour pressure of the liquid. The temperature at which vapour pressure of the liquid is equal to the external pressure is called boiling temperature at that pressure.
Relation Between Vapour Pressure and Temperature:
Vapour pressure of liquid increases with the increase in the temperature.
Clausius Clapeyron Equation:
Using this relation we can find vapour pressure at some another temperature when its value at some temperature is known.
Lowering of Vapour Pressure:
Liquids at a given temperature vapourize and under equilibrium conditions, the pressure exerted by the vapours of the liquid over the liquid phase is called vapour pressure [Fig (a)].
In a pure liquid, the entire surface is occupied by the molecules of the liquid. If a non-volatile solute is added to a solvent to give a solution [Fig. (b)], the vapour pressure of the solution is solely from the solvent alone. This vapour pressure of the solution at a given temperature is found to be lower than that of the pure solvent at the same temperature.
In the solution, the surface has both solute and solvent molecules; thereby the fraction of the surface covered by the solvent molecules gets reduced. Consequently, the number of solvent molecules escaping from the surface is correspondingly reduced thus, the vapour pressure is also reduced.
The decrease in the vapour pressure of solvent depends on the quantity of non-volatile solute present in the solution, irrespective of its nature.
Reasons for Lowering of Vapour Pressure:
The evaporation of liquid and evaporation of the liquid is a surface phenomenon. It is directly proportional to the surface area available for evaporation. Due to the addition of non-volatile solute the surface area of liquid decreases. It results in a decrease in the rate of evaporation and vapour pressure decreases.
By Graham’s law, the rate of evaporation is inversely proportional to the square root of the density of the liquid. When a solute is added to a solvent, the density of the resulting solution is more than the pure solvent. Hence the rate of evaporation decreases which results in the decrease of the vapour pressure of the solution.
Relative Lowering of Vapour Pressure:
The relative
lowering of vapour pressure for the given solution is the ratio of vapour
pressure lowering of solvent from the solution to the vapour pressure of the
pure solvent.
Mathematically, the relative lowering of vapour pressure is given by
Where,
p10= Vapour pressure of the pure solvent
Δp= Lowering of vapour pressure
p= Vapour pressure of the solution
Raoult’s Law:
The partial vapour pressure of any volatile component of a solution is the product of vapour pressure of that pure component and the mole fraction of the component in the solution.
Explanation:
Let us consider a solution containing two volatile components say A1 and A2, with mole fractions x1 and x2 respectively. Let p1o and p2o be the vapour pressures of the pure components A1 and A2 respectively, then by Raoult’s law
p1 = p1ox1 and p2 = p2o x2
The total vapour pressure of the solutions of two volatile components is the sum of partial vapour pressures of the two components
pT = p1 + p2
pT = p1ox1 + p2o x2
But x1 + x2 = 1
∴ x2 = 1 – x1
∴ pT = p1ox1 + p2o (1 – x1)
∴ pT = p1ox1 + p2o – p2ox1
∴ pT = p2o + ( p1o – p2o)x1
The solution which obeys Raoult’s law over the entire range of concentration is called an ideal solution. If a solution does not obey Raoul’s law are called non-ideal solutions.
Raoult’s Law for a Solution of Non-Volatile Solute:
Let us consider a solution containing two volatile component A1 and non-volatile component A2, with mole fractionsx1 and x1 respectively.
Let p10 and p20 be the vapour pressures of the pure components A1 and A2 respectively. Now component A2 is non-volatile, hence it will not contribute to vapour pressure. Thus p20 = 0. We have
pT = p2o + ( p1o – p2o)x1
∴ pT = 0 + ( p1o -0)x1
∴ pT = p1ox1
Thus vapour pressure of a solution of non-volatile solute is the product of vapour pressure p10 of pure solvent and mole fraction x1 of the solvent, which is Raoult’s law.
The equation shows that vapour pressure of the solution p < p10 , i.e. there is a lowering of the vapour pressure of the solution mathematically, it is given by
Δp = p1o – p
∴ Δp = p1o – p1ox1
∴ Δp = p1o( 1 – x1)
∴ Δp = p1ox2
In a
solution containing several non-volatile solutes, the lowering of the vapour
pressure depends on the sum of the mole fraction of different solutes.
Now, the relative lowering of vapour pressure is given by
This relation proves that the lowering of vapour pressure is colligative property because it depends on the concentration of non-volatile solute.
Raoult’s Law as a Special Case of Henry’s Law:
By Raoult’s law, we have
p = p1ox …………… (1)
By Henry’s law, we have
p = KHx …………… (2)
If we compare the two equations for Raoult’s law and Henry’s law, it can be seen that the partial pressure of the volatile component or gas is directly proportional to its mole fraction of solute in the solution. Only the proportionality constant KH differs from p10. Thus, Raoult’s law becomes a special case of Henry’s law in which KH is equal to p10.
Relation Between Molar Mass of Solute and Lowering of Vapour Pressure:
Let W2 g of the solute of molar mass M2 be dissolved in W1 g of the solvent of molar mass M1. The numbers of moles of solvent and solute are given by n1 = W1/M1 and n2 = W2/M2 respectively
The mole fraction of solute is given by
Now for dilute solutions n2 << n1. Hence n2 can be neglected.
This is the relation between the molar mass of solute and the lowering of vapour pressure.
Measurement of Lowering of Vapour Pressure:
Barometric Method:
Raoult introduced the liquid or the solution into the Torricellian vacuum of a barometer tube and measured the depression of the mercury level.
This method is neither practicable nor accurate as the lowering of vapour pressure is too small.
Manometric Method:
This method is generally used for aqueous solutions.
The bulb of the apparatus is charged with the liquid or solution. The air in the connecting tube is then removed with a vacuum pump. When the stopcock is closed, the pressure inside is due only to the vapour evaporating from the solution or liquid. The manometric liquid can be mercury or n-butyl phthalate which has a low density and low volatility.
Ostwald and Walker’s Dynamic Method (Gas Saturation Method):
In this method, the relative lowering of vapour pressure can be determined directly. The measurement of the individual vapour pressures of a solution and solvent is thus eliminated.
Apparatus: The apparatus used is shown in the figure. It consists of two sets of bulbs. The first set of three bulbs is filled with the solution to half of their capacity and second set of another three bulbs is filled with the pure solvent. Each set is separately weighed accurately. Both sets are connected to each other and then with the accurately weighed set of guard tubes filled with anhydrous calcium chloride or some other dehydrating agents like P2O5, concentrated H2SO4etc. The bulbs of the solution and pure solvent are kept in a thermostat maintained at a constant temperature.
Procedure: A current of pure dry air is bubbled through. The air gets saturated with the vapours in each set of bulbs. The air takes up the amount of vapours proportional to the vapour pressure of the solution first and then it takes up more amount of vapours from the solvent which is proportional to the difference in the vapour pressure of the solvent and the vapour pressure of the solution, i.e. p0 – p. The two sets of bulbs are weighed again. The guard tubes are also weighed.
Calculation:
The loss in the mass in the solution bulbs ∝ p
The loss in the mass in the solvent bulbs ∝ (p0 – p)
The total loss of the mass in both sets of bulbs ∝ [ps + (p0 – p)] ∝ p0
The total loss in the mass of both sets of bulbs is equal to gain in mass of guard tubes.
Thus measuring quantities on R.H.S. relative lowering of vapour pressure can be found.
Previous Topic: Ideal and Non-Ideal Solutions
Next Topic: Numerical Problems on Lowering of Vapour Pressure